Adding propositional connectives to countable infinitary logic

Abstract

For countable admissible α, one can add a new infinitary propositional connective to so that the extended language obeys the Barwise compactness theorem, and the set of valid sentences is complete α-r.e.

Aside from obeying the compactness theorem and a completeness theorem, ordinary finitary predicate calculus is also truth-functionally complete.

In (1), Barwise shows that for countable admissible A, 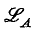provides a fragment of 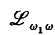which obeys a compactness theorem and a completeness theorem. However, we of course lose truth-functional completeness, with respect to infinitary propositional connectives that operate on infinite sequences of propositional variables. This raises the question of studying extensions of the language 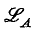obtained by adding infinitary propositional connectives, in connexion with the Barwise compactness and completeness theorems, and other metatheorems, proved for 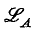Some aspects of this project are proposed in (3). It is the purpose of this paper to answer a few of the more basic questions which arise in this connexion.

We have not attempted to study the preservation of interpolation or implicit definability. This could be quite interesting if done systematically.