Published online by Cambridge University Press: 24 October 2008
Let M be a closed manifold of dimension 3 and let Fol(M) be the space of codimension one C∞-foliations on M. A foliation 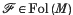 ∈ Fol(M) is said to be Cr- structurally stable if there exists a neighbourhood V of
∈ Fol(M) is said to be Cr- structurally stable if there exists a neighbourhood V of 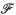 in Fol(M) in the (Epstein) Cr-topology such that every foliation
in Fol(M) in the (Epstein) Cr-topology such that every foliation 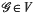 is topologically conjugate to
is topologically conjugate to 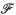 , through a homeomorphism near to the identity. Some background on the problem of structural stability of foliations can be found in [8]. In this paper we shall be concerned with proper foliations, i.e. foliations all of whose leaves are proper.
, through a homeomorphism near to the identity. Some background on the problem of structural stability of foliations can be found in [8]. In this paper we shall be concerned with proper foliations, i.e. foliations all of whose leaves are proper.