Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-25T05:05:09.968Z Has data issue: false hasContentIssue false
  • English
  • Français

On the summation of certain trigonometric series

Published online by Cambridge University Press:  24 October 2008

L. S. Goddard
L. S. Goddard
Affiliation:
St John's CollegeCambridge
Get access Rights & Permissions [Opens in a new window]

Extract

In the present note, which is introductory to the following paper, closed expressions, suitable for computational purposes, are found for the sums of the series

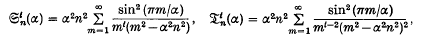
where α > 1, t = 1, 2, 3, …, and n is a positive integer. In each case a recurrent relation is found giving the values of 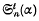and 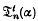for t > 2 in terms of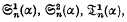 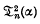and the series Θκ(α) (κ = 1, 2, …, t), where

When κ is even the last series is expressed in closed form in terms of the Bernoullian polynomial φκ(l/α) and, when κ is odd and α is rational, a closed form is found involving the polygamma function Ψ(κ)(z), where 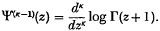The general expressions for 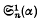and 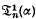involve Ψ(z) and Ψ′(z) when α is rational, but for special values of α they reduce to a form independent of the Ψ-function. 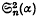and 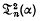are independent of n and are expressible as simple rational functions of α.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 41 , Issue 2 , June 1945 , pp. 145 - 160
Copyright
Copyright © Cambridge Philosophical Society 1945

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Bromwich, T. J. I'a.Introduction to the theory of infinite series (Cambridge, 1942).Google Scholar
(2)Pairman, , Eleanor, . Tables of the Digamma and Trigamma functions: Tracts for Computers, no. 1 (Cambridge, 1919).Google Scholar
(3)Goddard, L. S.Proc. Cambridge Phil. Soc. 39 (1943), 200.CrossRefGoogle Scholar
(4)Whittaker, E. T. and Watson, G. N.Modern analysis (Cambridge, 1927), p. 241.Google Scholar
(5)Schelkunoff, S. A.Quart. Appl. Math. 2 (1944), 90.CrossRefGoogle Scholar
(6)Macrobert, T. M.Functions of a complex variable (London, 1938).Google Scholar