Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-26T00:37:22.814Z Has data issue: false hasContentIssue false
  • English
  • Français

On Ramanujan's Arithmetical Function Σr, s(n)

Published online by Cambridge University Press:  24 October 2008

J. R. Wilton
J. R. Wilton
Affiliation:
Trinity College
Get access Rights & Permissions [Opens in a new window]

Extract

Let σs(n) denote the sum of the sth powers of the divisors of n,

and let 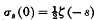, where ζ(s) is the Riemann ζfunction. Ramanujan, in his paper “On certain arithmetical functions*”, proves that, if

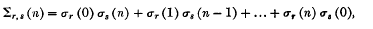
and

then 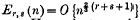, whenever r and s are odd positive integers. He conjectures that the error term on the right of (1·1) is of the form

for every ε > 0, and that it is not of the form 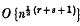. He further conjectures that

for all positive values of r and s; and this conjecture has recently been proved to be correct.

Type
Articles
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 25 , Issue 3 , July 1929 , pp. 255 - 264
Copyright
Copyright © Cambridge Philosophical Society 1929

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

* Trans. Camb. Phil. Soc. 22 (1916), 159–184;Google ScholarCollected Papers, No. 18. It is to be observed, in (1·1), that ζ(1-r)=0 if r≠=1, ζ(0)=−½.

† Ingham, A. E., Journal London Math. Soc. 2 (1927), 202–208.CrossRefGoogle Scholar

‡ Ramanujan, , loc. cit.Google Scholar; Hardy, Q. H., Proc. Camb. Phil. Soc. 23 (1927), 675–680.CrossRefGoogle Scholar

* The error term is then actually zero—as also when r + s is equal to 4, 6, 8 or 12.

† Wigert, S., Acta Math. 37, (1914), p. 113–140.CrossRefGoogle Scholar

‡ Compare Hardy, and Ramanujan, , “Asymptotic formulae in combinatory analysis”, Proc. London Math. Soc. (2) 17 (1918), 75–115, Lemma 4·31;CrossRefGoogle Scholar Ramanujan's Collected Papers, No. 36.

* In particular (0, q) = q.Google Scholar

† Vorlesungen über Zahlentheorie (1927), 1, 217–218.Google Scholar

* It is a corollary from (2·32) and the transformation equation of the modular function

that Ramanujan's function τ (n) is O(n 6).—Hardy, loc. cit.

* For the proof of (2·51) see, for example, Landau, loc. cit. 1, 188. The inner sum is to be taken over the common divisors d of q and n.Google Scholar

* The case k = 1 leads merely to an identity.Google Scholar

* When h = k, the term v = k; is merely a repetition of that for which v = k − 1.Google Scholar

† It is not, in general, true (as it is when k = 2) that Q 1(x) disappears from this polynomial.Google Scholar

‡ Hardy, , loc. cit.Google Scholar

* It is true when k = 2, as Ramanujan shows.Google Scholar

† I require one additional entry in the case of Σ1117(n).Google Scholar

‡ T(n)= Er, s(n)/Er, s(1) if r + s = 14.—Ramanujan, loc. cit., equation (95).

* For a remarkable deduction from, this congruence—a deduction stated and partly proved by Ramanujan—see Miss Stanley, G. K., “Two assertions made by Ramanujan”, Journal London Math. Soc. 3 (1928), 232–237, where, on p. 235, 1. 8 from the end, “number of” should read “sum of the”.CrossRefGoogle Scholar

† This relation follows immediately from equation (1·63) of Ramanujan's posthumous paper, “Congruence properties of partitions”, Math. Zeitschrift, 9 (1921), 147–153 (Collected Papers, No. 30), but Ramanujan does not seem to have noticed the fact. The same formula (1·63) occurs as line 6 of Table I of the paper “On certain arithmetical functions”, No. 18.CrossRefGoogle Scholar

‡ This relation was conjectured by Ramanujan and was subsequently proved by Mordell, , Proc. Camb. Phil. Soc. 19 (1919), 117–124.Google Scholar

§ Mordell, , loc. cit. 119, equation (4), in which κ = 16, ξ = 1.Google Scholar