Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Gerl, Peter
1986.
Probability Measures on Groups VIII.
Vol. 1210,
Issue. ,
p.
285.
Franchi, J.
1994.
Enroulements asymptotiques du mouvement brownien autour de lacets dans une variété riemannienne compacte de dimension 3.
Stochastic Processes and their Applications,
Vol. 52,
Issue. 2,
p.
251.
Kholodenko, Arkady L.
2001.
Some thoughts about random walks on figure eight.
Physica A: Statistical Mechanics and its Applications,
Vol. 289,
Issue. 3-4,
p.
377.
Rasetti, Mario
2014.
Is quantum simulation of turbulence within reach?.
International Journal of Quantum Information,
Vol. 12,
Issue. 07n08,
p.
1560008.


 be the 3-dimensional sphere and let
be the 3-dimensional sphere and let 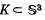 be a smooth knot, i.e.
be a smooth knot, i.e. 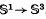 . We say that
. We say that 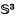 onto the unit sphere of
onto the unit sphere of 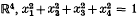 , that brings
, that brings 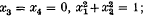 otherwise we say that
otherwise we say that 