No CrossRef data available.
Published online by Cambridge University Press: 23 January 2015
In [1] Niven used the integral
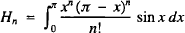
to give a well-known proof of the irrationality of π. Recently Zhou and Markov [2] used a recurrence relation satisfied by this integral to present an alternative proof which may be more direct than Niven's.
Niven did not cite any references in [1] and thus the origin or Hn seems rather mysterious and ingenious. However if we heed Abel's advice to ‘study the masters’, we find that Hn emerged much more naturally from the great works of Lambert [3] and Hermite [4].