Published online by Cambridge University Press: 01 August 2016
In common with, I suspect, many people the author does not have access to the NAG library and so, when I was asked recently to calculate the value of the integral
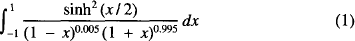
correct to 10 decimal places my first reaction was to try several different calculators as well as several mathematical software packages. On doing so it was disappointing to find they either gave widely differing values such as 7.9065200767, 4.1317217452 or 0.9174196842 or an error message indicating that the method had not converged.