No CrossRef data available.
Article contents
THÉORIE DE BRUHAT-TITS POUR LES GROUPES QUASI-RÉDUCTIFS
Published online by Cambridge University Press: 25 January 2021
Abstract
Soient K un corps discrètement valué et hensélien,
 ${\mathcal {O}}$
son anneau d’entiers supposé excellent,
${\mathcal {O}}$
son anneau d’entiers supposé excellent,
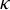 $\kappa $
son corps résiduel supposé parfait et G un K-groupe quasi-réductif, c’est-à-dire lisse, affine, connexe et à radical unipotent déployé trivial. On construit l’immeuble de Bruhat-Tits
$\kappa $
son corps résiduel supposé parfait et G un K-groupe quasi-réductif, c’est-à-dire lisse, affine, connexe et à radical unipotent déployé trivial. On construit l’immeuble de Bruhat-Tits
 ${\mathcal {I}}(G, K)$
pour
${\mathcal {I}}(G, K)$
pour
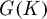 $G(K)$
de façon canonique, améliorant les constructions moins canoniques de M. Solleveld sur les corps locaux, et l’on associe un
$G(K)$
de façon canonique, améliorant les constructions moins canoniques de M. Solleveld sur les corps locaux, et l’on associe un
 ${\mathcal {O}}$
-modèle en groupes
${\mathcal {O}}$
-modèle en groupes
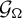 ${\mathcal {G}}_{\Omega }$
de G à chaque partie non vide et bornée
${\mathcal {G}}_{\Omega }$
de G à chaque partie non vide et bornée
 $\Omega $
contenue dans un appartement de
$\Omega $
contenue dans un appartement de
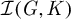 ${\mathcal {I}}(G,K)$
. On montre que les groupes parahoriques
${\mathcal {I}}(G,K)$
. On montre que les groupes parahoriques
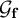 ${\mathcal {G}}_{\textbf {f}}$
attachés aux facettes peuvent être caractérisés en fonction de la géométrie de leurs grassmanniennes affines, ainsi que dans la thèse de T. Richarz. Ces résultats sont appliqués ailleurs à l’étude des grassmanniennes affines tordues entières.
${\mathcal {G}}_{\textbf {f}}$
attachés aux facettes peuvent être caractérisés en fonction de la géométrie de leurs grassmanniennes affines, ainsi que dans la thèse de T. Richarz. Ces résultats sont appliqués ailleurs à l’étude des grassmanniennes affines tordues entières.
Keywords
- Type
- Research Article
- Information
- Journal of the Institute of Mathematics of Jussieu , Volume 21 , Issue 4 , July 2022 , pp. 1331 - 1362
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press



