Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-27T14:24:18.423Z Has data issue: false hasContentIssue false
  • English
  • Français

UNIQUENESS OF THE WELDING PROBLEM FOR SLE AND LIOUVILLE QUANTUM GRAVITY

Part of: Markov processes Special processes Geometric probability and stochastic geometry

Published online by Cambridge University Press:  05 July 2019

Oliver McEnteggart ,
Jason Miller  and
Wei Qian Open the ORCID record for Wei Qian [Opens in a new window]
Oliver McEnteggart
Affiliation:
Department of Pure Mathematics and Mathematical Statistics, University of Cambridge, Cambridge, UK ([email protected])
Jason Miller
Affiliation:
Department of Pure Mathematics and Mathematical Statistics, University of Cambridge, Cambridge, UK ([email protected])
Wei Qian
Affiliation:
Department of Pure Mathematics and Mathematical Statistics, University of Cambridge, Cambridge, UK ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

We give a simple set of geometric conditions on curves $\unicode[STIX]{x1D702}$, 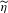$\widetilde{\unicode[STIX]{x1D702}}$ in 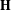$\mathbf{H}$ from $0$ to 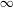$\infty$ so that if $\unicode[STIX]{x1D711}:\mathbf{H}\rightarrow \mathbf{H}$ is a homeomorphism which is conformal off $\unicode[STIX]{x1D702}$ with 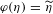$\unicode[STIX]{x1D711}(\unicode[STIX]{x1D702})=\widetilde{\unicode[STIX]{x1D702}}$ then $\unicode[STIX]{x1D711}$ is a conformal automorphism of 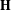$\mathbf{H}$. Our motivation comes from the fact that it is possible to apply our result to random conformal welding problems related to the Schramm–Loewner evolution (SLE) and Liouville quantum gravity (LQG). In particular, we show that if $\unicode[STIX]{x1D702}$ is a non-space-filling 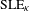$\text{SLE}_{\unicode[STIX]{x1D705}}$ curve in 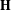$\mathbf{H}$ from $0$ to $\infty$, and $\unicode[STIX]{x1D711}$ is a homeomorphism which is conformal on 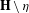$\mathbf{H}\setminus \unicode[STIX]{x1D702}$, and 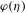$\unicode[STIX]{x1D711}(\unicode[STIX]{x1D702})$, $\unicode[STIX]{x1D702}$ are equal in distribution, then $\unicode[STIX]{x1D711}$ is a conformal automorphism of 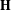$\mathbf{H}$. Applying this result for $\unicode[STIX]{x1D705}=4$ establishes that the welding operation for critical (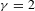$\unicode[STIX]{x1D6FE}=2$) LQG is well defined. Applying it for $\unicode[STIX]{x1D705}\in (4,8)$ gives a new proof that the welding of two independent $\unicode[STIX]{x1D705}/4$-stable looptrees of quantum disks to produce an 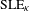$\text{SLE}_{\unicode[STIX]{x1D705}}$ on top of an independent 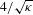$4/\sqrt{\unicode[STIX]{x1D705}}$-LQG surface is well defined.

Keywords

Conformal weldingLiouville quantum gravitySchramm–Loewner evolution

MSC classification

Primary: 60D05: Geometric probability and stochastic geometry 60J67: Stochastic (Schramm-)Loewner evolution (SLE)
Secondary: 60K35: Interacting random processes; statistical mechanics type models; percolation theory
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 20 , Issue 3 , May 2021 , pp. 757 - 783
Check for updates
Copyright
© Cambridge University Press 2019

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahlfors, L. V., Lectures on Quasiconformal Mappings, second edition, University Lecture Series, Volume 38 (American Mathematical Society, Providence, RI, 2006). With supplemental chapters by C. J. Earle, I. Kra, M. Shishikura and J. H. Hubbard.Google Scholar
Aru, J., Huang, Y. and Sun, X., Two perspectives of the 2D unit area quantum sphere and their equivalence, Commun. Math. Phys. 356(1) (2017), 261–283.CrossRefGoogle Scholar
Astala, K., Jones, P., Kupiainen, A. and Saksman, E., Random conformal weldings, Acta Math. 207(2) (2011), 203–254.CrossRefGoogle Scholar
Bass, R. F., Probabilistic Techniques in Analysis, Probability and its Applications (New York), (Springer, New York, 1995).Google Scholar
Berestycki, N., An elementary approach to Gaussian multiplicative chaos, Electron. Commun. Probab. 22(Paper No. 27) (2017), 1–12.CrossRefGoogle Scholar
David, F., Kupiainen, A., Rhodes, R. and Vargas, V., Liouville quantum gravity on the Riemann sphere, Commun. Math. Phys. 342(3) (2016), 869–907.CrossRefGoogle Scholar
Ding, J., Dubédat, J., Dunlap, A. and Falconet, H., Tightness of Liouville first passage percolation for $\unicode[STIX]{x1D6FE}\in (0,2)$ , Preprint, April 2019, arXiv:1904.08021.CrossRefGoogle Scholar
Dubédat, J., SLE and the free field: partition functions and couplings, J. Amer. Math. Soc. 22(4) (2009), 995–1054.CrossRefGoogle Scholar
Dubédat, J., Falconet, H., Gwynne, E., Pfeffer, J. and Sun, X., Weak LQG metrics and Liouville first passage percolation, Preprint, May 2019, arXiv:1905.00380.CrossRefGoogle Scholar
Duplantier, B., Miller, J. and Sheffield, S., Liouville quantum gravity as a mating of trees, Preprint, September 2014, ArXiv e-prints.Google Scholar
Duplantier, B., Rhodes, R., Sheffield, S. and Vargas, V., Critical Gaussian multiplicative chaos: convergence of the derivative martingale, Ann. Probab. 42(5) (2014), 1769–1808.CrossRefGoogle Scholar
Duplantier, B., Rhodes, R., Sheffield, S. and Vargas, V., Renormalization of critical Gaussian multiplicative chaos and KPZ relation, Commun. Math. Phys. 330(1) (2014), 283–330.CrossRefGoogle Scholar
Duplantier, B. and Sheffield, S., Liouville quantum gravity and KPZ, Invent. Math. 185(2) (2011), 333–393.CrossRefGoogle Scholar
Gwynne, E., Kassel, A., Miller, J. and Wilson, D. B., Active spanning trees with bending energy on planar maps and SLE-decorated Liouville quantum gravity for 𝜅 > 8, Commun. Math. Phys. 358(3) (2018), 1065–1115.CrossRefGoogle Scholar
Gwynne, E. and Miller, J., Convergence of the self-avoiding walk on random quadrangulations to SLE $_{8/3}$ on $\sqrt{8/3}$ -Liouville quantum gravity, Preprint, August 2016, ArXiv e-prints.Google Scholar
Gwynne, E. and Miller, J., Convergence of percolation on uniform quadrangulations with boundary to SLE $_{6}$ on $\sqrt{8/3}$ -Liouville quantum gravity, Preprint, January 2017, ArXiv e-prints.Google Scholar
Gwynne, E. and Miller, J., Confluence of geodesics in Liouville quantum gravity for $\unicode[STIX]{x1D6FE}\in (0,2)$ , Preprint, May 2019, arXiv:1905.00381.CrossRefGoogle Scholar
Gwynne, E. and Miller, J., Conformal covariance of the Liouville quantum gravity metric for $\unicode[STIX]{x1D6FE}\in (0,2)$ , Preprint, May 2019, arXiv:1905.00384.Google Scholar
Gwynne, E. and Miller, J., Existence and uniqueness of the Liouville quantum gravity metric for $\unicode[STIX]{x1D6FE}\in (0,2)$ , Preprint, May 2019, arXiv:1905.00383.CrossRefGoogle Scholar
Gwynne, E. and Miller, J., Local metrics of the Gaussian free field, Preprint, May 2019, arXiv:1905.00379.Google Scholar
Gwynne, E., Miller, J. and Sun, X., Almost sure multifractal spectrum of Schramm–Loewner evolution, Duke Math. J. 167(6) (2018), 1099–1237.CrossRefGoogle Scholar
Høegh Krohn, R., A general class of quantum fields without cut-offs in two space–time dimensions, Commun. Math. Phys. 21 (1971), 244–255.CrossRefGoogle Scholar
Holden, N. and Powell, E., Conformal welding for critical Liouville quantum gravity, Preprint, December 2018, arXiv:1812.11808.Google Scholar
Huang, Y., Rhodes, R. and Vargas, V., Liouville quantum gravity on the unit disk, Ann. Inst. Henri Poincaré Probab. Stat. 54(3) (2018), 1694–1730.CrossRefGoogle Scholar
Jones, P. W., On removable sets for Sobolev spaces in the plane, in Essays on Fourier Analysis in Honor of Elias M. Stein (Princeton, NJ, 1991), Princeton Mathematical Series, Volume 42, pp. 250–267 (Princeton University Press, Princeton, NJ, 1995).CrossRefGoogle Scholar
Jones, P. W. and Smirnov, S. K., Removability theorems for Sobolev functions and quasiconformal maps, Ark. Mat. 38(2) (2000), 263–279.CrossRefGoogle Scholar
Junnila, J. and Saksman, E., Uniqueness of critical Gaussian chaos, Electron. J. Probab. 22(Paper No. 11) (2017), 1–31.CrossRefGoogle Scholar
Kahane, J.-P., Sur le chaos multiplicatif, Ann. Sci. Math. Québec 9(2) (1985), 105–150.Google Scholar
Kaufman, R. and Wu, J.-M., On removable sets for quasiconformal mappings, Ark. Mat. 34(1) (1996), 141–158.CrossRefGoogle Scholar
Kenyon, R., Miller, J., Sheffield, S. and Wilson, D. B., Bipolar orientations on planar maps and SLE $_{12}$ , Ann. Probab., Preprint, November 2015, ArXiv e-prints, to appear.Google Scholar
Koskela, P. and Nieminen, T., Quasiconformal removability and the quasihyperbolic metric, Indiana Univ. Math. J. 54(1) (2005), 143–151.CrossRefGoogle Scholar
Lawler, G. F. and Rezaei, M. A., Minkowski content and natural parameterization for the Schramm–Loewner evolution, Ann. Probab. 43(3) (2015), 1082–1120.CrossRefGoogle Scholar
Li, Y., Sun, X. and Watson, S. S., Schnyder woods, SLE(16), and Liouville quantum gravity, Preprint, May 2017, ArXiv e-prints.Google Scholar
Miller, J., Dimension of the SLE light cone, the SLE fan, and SLE𝜅(𝜌) for 𝜅 ∈ (0, 4) and $\rho~\in~[\frac{\kappa}{2}-4,-2)$ , Commun. Math. Phys. 360(3) (2018), 1083–1119.CrossRefGoogle Scholar
Miller, J. and Sheffield, S., Liouville quantum gravity and the Brownian map I: the QLE(8/3,0) metric, Preprint, July 2015, ArXiv e-prints.Google Scholar
Miller, J. and Sheffield, S., Gaussian free field light cones and SLE $_{\unicode[STIX]{x1D705}}(\unicode[STIX]{x1D70C})$ , Ann. Probab., Preprint, June 2016, ArXiv e-prints, to appear.Google Scholar
Miller, J. and Sheffield, S., Imaginary geometry I: interacting SLEs, Probab. Theory Related Fields 164(3–4) (2016), 553–705.CrossRefGoogle Scholar
Miller, J. and Sheffield, S., Imaginary geometry III: reversibility of SLE𝜅 for 𝜅 ∈ (4, 8), Ann. of Math. (2) 184(2) (2016), 455–486.CrossRefGoogle Scholar
Miller, J. and Sheffield, S., Liouville quantum gravity and the Brownian map II: geodesics and continuity of the embedding, Preprint, May 2016, arXiv:1605.03563.Google Scholar
Miller, J. and Sheffield, S., Liouville quantum gravity and the Brownian map III: the conformal structure is determined, Preprint, August 2016, arXiv:1608.05391.Google Scholar
Miller, J. and Sheffield, S., Imaginary geometry IV: interior rays, whole-plane reversibility, and space-filling trees, Probab. Theory Related Fields 169(3–4) (2017), 729–869.CrossRefGoogle Scholar
Miller, J., Sheffield, S. and Werner, W., CLE percolations, Forum Math. Pi 5(e4) (2017), 1–102.CrossRefGoogle Scholar
Miller, J., Sheffield, S. and Werner, W., (2018). In preparation.Google Scholar
Ntalampekos, D., A removability theorem for Sobolev functions and detour sets, Preprint, June 2017, arXiv:1706.07687.Google Scholar
Ntalampekos, D., Non-removability of the Sierpinski Gasket, Inventiones, Preprint, April 2018, arXiv:1804.10239, to appear.CrossRefGoogle Scholar
Powell, E., Critical Gaussian chaos: convergence and uniqueness in the derivative normalisation, Electron. J. Probab. 23(Paper No. 31) (2018), 1–26.CrossRefGoogle Scholar
Rezaei, M. A. and Zhan, D., Green’s functions for chordal SLE curves, Probab. Theory Related Fields 171(3–4) (2018), 1093–1155.CrossRefGoogle Scholar
Rezaei, M. A. and Zhan, D., Higher moments of the natural parameterization for SLE curves, Ann. Inst. Henri Poincaré Probab. Stat. 53(1) (2017), 182–199.CrossRefGoogle Scholar
Rhodes, R. and Vargas, V., Gaussian multiplicative chaos and applications: a review, Probab. Surv. 11 (2014), 315–392.CrossRefGoogle Scholar
Robert, R. and Vargas, V., Gaussian multiplicative chaos revisited, Ann. Probab. 38(2) (2010), 605–631.CrossRefGoogle Scholar
Rohde, S. and Schramm, O., Basic properties of SLE, Ann. of Math. (2) 161(2) (2005), 883–924.CrossRefGoogle Scholar
Schramm, O., Scaling limits of loop-erased random walks and uniform spanning trees, Israel J. Math. 118 (2000), 221–288.CrossRefGoogle Scholar
Schramm, O. and Sheffield, S., A contour line of the continuum Gaussian free field, Probab. Theory Related Fields 157(1–2) (2013), 47–80.CrossRefGoogle Scholar
Sheffield, S., Exploration trees and conformal loop ensembles, Duke Math. J. 147(1) (2009), 79–129.CrossRefGoogle Scholar
Sheffield, S., Conformal weldings of random surfaces: SLE and the quantum gravity zipper, Ann. Probab. 44(5) (2016), 3474–3545.CrossRefGoogle Scholar
Sheffield, S., Quantum gravity and inventory accumulation, Ann. Probab. 44(6) (2016), 3804–3848.CrossRefGoogle Scholar
Sheffield, S. and Werner, W., Conformal loop ensembles: the Markovian characterization and the loop-soup construction, Ann. of Math. (2) 176(3) (2012), 1827–1917.CrossRefGoogle Scholar
Werness, B. M., Regularity of Schramm–Loewner evolutions, annular crossings, and rough path theory, Electron. J. Probab. 17(81) (2012), 1–21.CrossRefGoogle Scholar
Zhan, D., Reversibility of chordal SLE, Ann. Probab. 36(4) (2008), 1472–1494.CrossRefGoogle Scholar