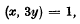Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Gupta, N. D.
1969.
Certain locally metanilpotent varieties of groups.
Archiv der Mathematik,
Vol. 20,
Issue. 5,
p.
481.
Gupta, C. K.
Gupta, N. D.
and
Newman, M. F.
1969.
Some Finite Nilpotent p-Groups.
Journal of the Australian Mathematical Society,
Vol. 9,
Issue. 3-4,
p.
287.
Bachmuth, S.
and
Mochizuki, H.Y.
1971.
Third Engel groups and the Macdonald-Neumann conjecture.
Bulletin of the Australian Mathematical Society,
Vol. 5,
Issue. 3,
p.
379.
Kappe, L.-C.
and
Kappe, W.P.
1972.
On three-Engel groups.
Bulletin of the Australian Mathematical Society,
Vol. 7,
Issue. 3,
p.
391.
Gupta, Narain
1972.
Third-Engel 2-Groups are Soluble.
Canadian Mathematical Bulletin,
Vol. 15,
Issue. 4,
p.
523.
Newman, M. F.
1973.
Some varieties of groups.
Journal of the Australian Mathematical Society,
Vol. 16,
Issue. 4,
p.
481.
Plotkin, B. I.
1973.
General theory of groups.
Journal of Soviet Mathematics,
Vol. 1,
Issue. 5,
p.
527.
Chunikhin, S. A.
and
Shemetkov, L. A.
1973.
Finite groups.
Journal of Soviet Mathematics,
Vol. 1,
Issue. 3,
p.
291.
Macdonald, I. D.
1974.
A computer application to finite p-groups.
Journal of the Australian Mathematical Society,
Vol. 17,
Issue. 1,
p.
102.
Gupta, Narain
Levin, Frank
and
Rhemtulla, Akbar
1974.
Chains of Varieties.
Canadian Journal of Mathematics,
Vol. 26,
Issue. 1,
p.
190.
Gupta, N. D.
and
Newman, M. F.
1989.
Third Engel groups.
Bulletin of the Australian Mathematical Society,
Vol. 40,
Issue. 2,
p.
215.
Endimioni, G�rard
1991.
Groupes de type [d?3(d?1)].
Archiv der Mathematik,
Vol. 57,
Issue. 1,
p.
6.
Traustason, Gunnar
2011.
Groups St Andrews 2009 in Bath.
p.
520.