Article contents
SOME HOMOLOGICAL PROPERTIES OF CATEGORY 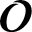 $\boldsymbol {\mathcal {O}}$ FOR LIE SUPERALGEBRAS
$\boldsymbol {\mathcal {O}}$ FOR LIE SUPERALGEBRAS
Published online by Cambridge University Press: 21 January 2022
Abstract
For classical Lie superalgebras of type I, we provide necessary and sufficient conditions for a Verma supermodule
 $\Delta (\lambda )$
to be such that every nonzero homomorphism from another Verma supermodule to
$\Delta (\lambda )$
to be such that every nonzero homomorphism from another Verma supermodule to
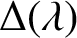 $\Delta (\lambda )$
is injective. This is applied to describe the socle of the cokernel of an inclusion of Verma supermodules over the periplectic Lie superalgebras
$\Delta (\lambda )$
is injective. This is applied to describe the socle of the cokernel of an inclusion of Verma supermodules over the periplectic Lie superalgebras
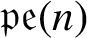 $\mathfrak {pe} (n)$
and, furthermore, to reduce the problem of description of
$\mathfrak {pe} (n)$
and, furthermore, to reduce the problem of description of
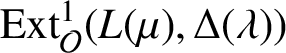 $\mathrm {Ext}^1_{\mathcal O}(L(\mu ),\Delta (\lambda ))$
for
$\mathrm {Ext}^1_{\mathcal O}(L(\mu ),\Delta (\lambda ))$
for
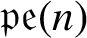 $\mathfrak {pe} (n)$
to the similar problem for the Lie algebra
$\mathfrak {pe} (n)$
to the similar problem for the Lie algebra
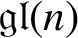 $\mathfrak {gl}(n)$
. Additionally, we study the projective and injective dimensions of structural supermodules in parabolic category
$\mathfrak {gl}(n)$
. Additionally, we study the projective and injective dimensions of structural supermodules in parabolic category
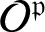 $\mathcal O^{\mathfrak {p}}$
for classical Lie superalgebras. In particular, we completely determine these dimensions for structural supermodules over the periplectic Lie superalgebra
$\mathcal O^{\mathfrak {p}}$
for classical Lie superalgebras. In particular, we completely determine these dimensions for structural supermodules over the periplectic Lie superalgebra
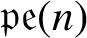 $\mathfrak {pe} (n)$
and the orthosymplectic Lie superalgebra
$\mathfrak {pe} (n)$
and the orthosymplectic Lie superalgebra
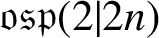 $\mathfrak {osp}(2|2n)$
.
$\mathfrak {osp}(2|2n)$
.
MSC classification
- Type
- Research Article
- Information
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.
Footnotes
Communicated by Anthony Henderson
The first author is partially supported by MoST grant 108-2115-M-008-018-MY2. For the second author, the research was partially supported by the Swedish Research Council and Göran Gustafssons Stiftelse.
References
- 1
- Cited by



