FURTHER RESULTS ON VANISHING COEFFICIENTS IN INFINITE PRODUCT EXPANSIONS

Abstract

We extend results of Andrews and Bressoud [‘Vanishing coefficients in infinite product expansions’, J. Aust. Math. Soc. Ser. A27(2) (1979), 199–202] on the vanishing of coefficients in the series expansions of certain infinite products. These results have the form that if

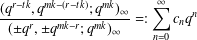$$\begin{eqnarray}\frac{(q^{r-tk},q^{mk-(r-tk)};q^{mk})_{\infty }}{(\pm q^{r},\pm q^{mk-r};q^{mk})_{\infty }}=:\mathop{\sum }_{n=0}^{\infty }c_{n}q^{n}\end{eqnarray}$$

for certain integers $k$, $m$, $s$ and $t$, where 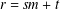$r=sm+t$, then 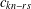$c_{kn-rs}$ is always zero. Our theorems also partly give a simpler reformulation of results of Alladi and Gordon [‘Vanishing coefficients in the expansion of products of Rogers–Ramanujan type’, in: The Rademacher Legacy to Mathematics (University Park, PA, 1992), Contemporary Mathematics, 166 (American Mathematical Society, Providence, RI, 1994), 129–139], but also give results for cases not covered by the theorems of Alladi and Gordon. We also give some interpretations of the analytic results in terms of integer partitions.