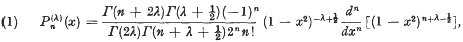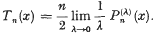Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Elliott, David
1968.
Error analysis of an algorithm for summing certain finite series.
Journal of the Australian Mathematical Society,
Vol. 8,
Issue. 2,
p.
213.
1975.
Mathematical Functions and their Approximations.
p.
517.
Lewanowicz, Stanisław
1979.
Construction of a recurrence relation for modified moments.
Journal of Computational and Applied Mathematics,
Vol. 5,
Issue. 3,
p.
193.
Lewanowicz, Stanisław
1986.
Recurrence Relations for the Coefficients in Jacobi Series Solutions of Linear Differential Equations.
SIAM Journal on Mathematical Analysis,
Vol. 17,
Issue. 5,
p.
1037.
DOHA, E.H.
1988.
Mathematical Analysis and its Applications.
p.
251.
Doha, E.H.
1998.
The ultraspherical coefficients of the moments of a general-order derivative of an infinitely differentiable function.
Journal of Computational and Applied Mathematics,
Vol. 89,
Issue. 1,
p.
53.
Yang, S. A.
2002.
Evaluation of 2‐D Green's boundary formula and its normal derivative using Legendre polynomials, with an application to acoustic scattering problems.
International Journal for Numerical Methods in Engineering,
Vol. 53,
Issue. 4,
p.
905.
Boyd, John P.
2009.
Large-degree asymptotics and exponential asymptotics for Fourier, Chebyshev and Hermite coefficients and Fourier transforms.
Journal of Engineering Mathematics,
Vol. 63,
Issue. 2-4,
p.
355.
De Micheli, Enrico
and
Viano, Giovanni Alberto
2013.
The expansion in Gegenbauer polynomials: A simple method for the fast computation of the Gegenbauer coefficients.
Journal of Computational Physics,
Vol. 239,
Issue. ,
p.
112.
Salazar, Robert
Téllez, Gabriel
and
Bayona-Roa, Camilo
2021.
Expansion formula for the magnetic field of a periodically deformed circular current loop.
Physica Scripta,
Vol. 96,
Issue. 12,
p.
125502.
Nedaei Janbesaraei, Sherwin
Azmoon, Amirreza
and
Baleanu, Dumitru
2023.
Learning with Fractional Orthogonal Kernel Classifiers in Support Vector Machines.
p.
93.
Al-Masaeed, Mohamed Ghaleb
Rabei, Eqab M.
Muslih, Sami I.
and
Baleanu, Dumitru
2024.
Solving Conformable Gegenbauer Differential Equation and Exploring Its Generating Function.
International Journal of Applied and Computational Mathematics,
Vol. 10,
Issue. 6,


 (
(