Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-25T08:58:37.924Z Has data issue: false hasContentIssue false
  • English
  • Français

The convergence of Rayleigh-Ritz approximations in hydrodynamics

Published online by Cambridge University Press:  09 April 2009

P. E. Lush
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

It is known that various cases of the steady isentropic irrotational motion of a compressible fluid are expressible as variational principle [1], [5]. in particular, the aerofoil problem i.e. the case of plane flow in which a uniform stream is locally deflected, without circulation, by a bounded obstacle, can be expressed in such a form. Thus we make stationary 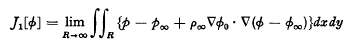where the region R is that bounded internally by the obstacle (C0) and externally by a circle (CR) of radius R. In this expression φ∞ is the velocity potential for a uniform stream, and φ0 is the velocity potential for the corresponding incompressible flow.

Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 3 , Issue 1 , February 1963 , pp. 99 - 103
Copyright
Copyright © Australian Mathematical Society 1963

References

[1]Lush, P. E. and Cherry, T. M., Variational method in hydrodynamics. Quart. J. of Mech. and Appl. Math. 9 (1956) 6–21.CrossRefGoogle Scholar
[2]Shiffman, M., On the existence of subsonic flows of a compressible fluid. J. Rat Mech. and Anal. 1 (1962), 605–652.Google Scholar
[3]Courant, R., Dirichlet' Principe, Conformal Mapping, and Minimal Surfaces. Interscience. New York (1950).Google Scholar
[4]Morry, C. B., Problems in the Calculus of Variations and Related Topics. Univ. of Calif. Publ. in Math. 1 (1943).Google Scholar
[5]Serrion, J., Mathematical Principles of Classical Fluid Dynamics. Handbuch der Physik Vol. 8, Springer (1959).Google Scholar