Article contents
Solution of a problem of Leon Henkin1
Published online by Cambridge University Press: 12 March 2014
Extract
If Σ is any standard formal system adequate for recursive number theory, a formula (having a certain integer q as its Gödel number) can be constructed which expresses the proposition that the formula with Gödel number q is provable in Σ. Is this formula provable or independent in Σ? [2].
One approach to this problem is discussed by Kreisel in [4]. However, he still leaves open the question whether the formula (Ex)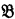 (x, a), with Gödel-number a, is provable or not. Here
(x, a), with Gödel-number a, is provable or not. Here 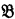 (x, y) is the number-theoretic predicate which expresses the proposition that x is the number of a formal proof of the formula with Gödel-number y.
(x, y) is the number-theoretic predicate which expresses the proposition that x is the number of a formal proof of the formula with Gödel-number y.
In this note we present a solution of the previous problem with respect to the system Zμ [3] pp. 289–294, and, more generally, with respect to any system whose set of theorems is closed under the rules of inference of the first order predicate calculus, and satisfies the subsequent five conditions, and in which the function 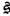 (k, l) used below is definable.
(k, l) used below is definable.
The notation and terminology is in the main that of [3] pp. 306–326, viz. if 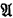 is a formula of Zμ containing no free variables, whose Gödel number is a, then
is a formula of Zμ containing no free variables, whose Gödel number is a, then  ({
({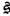 }) stands for (Ex)
}) stands for (Ex)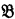 (x, a) (read: the formula with Gödel number a is provable in Zμ); if
(x, a) (read: the formula with Gödel number a is provable in Zμ); if 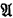 is a formula of Zμ containing a free variable, y say,
is a formula of Zμ containing a free variable, y say,  ({
({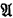 }) stands for (Ex)
}) stands for (Ex)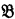 (x, g(y)}, where g(y) is a recursive function such that for an arbitrary numeral
(x, g(y)}, where g(y) is a recursive function such that for an arbitrary numeral  the value of g(
the value of g( ) is the Gödel number of the formula obtained from
) is the Gödel number of the formula obtained from 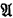 by substituting
by substituting  for y in
for y in 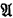 throughout. We shall, however, depart trivially from [3] in writing
throughout. We shall, however, depart trivially from [3] in writing  (
( ), where
), where  is an arbitrary numeral, for (Ex)
is an arbitrary numeral, for (Ex)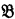 {x,
{x,  ).
).
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1955
Footnotes
The substance of this note was contained in a lecture held at the International Congress of Mathematicians, Amsterdam, 1954.
References
BIBLIOGRAPHY
- 156
- Cited by


