Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-12-02T19:58:02.697Z Has data issue: false hasContentIssue false
  • English
  • Français

The universal complementation property

Published online by Cambridge University Press:  12 March 2014

R.G. Downey  and
J.B. Remmel
R.G. Downey
Affiliation:
National University of Singapore, Kent Ridge 0511, Singapore
J.B. Remmel
Affiliation:
University of California at San Diego, La Jolla, California 92093
Get access Rights & Permissions [Opens in a new window]

Extract

Let V∞ be a fully effective infinite dimensional vector space over a recursive field F. That is, we assume that the universe of V∞ is a recursive set, the operations of addition and scalar multiplication are recursive, and there is a uniform effective procedure to decide whether any finite set {υ0, …, υn} of vectors from V∞ is independent. The lattice 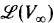of recursively enumerable subspaces has been extensively studied since its introduction by Metakides and Nerode [MN1] (see for example, [Do2], [Gu], [KR], [Re1], [Re2], and [Sh]). For those unfamiliar with the literature on 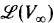, we shall give a list of basic definitions required for this paper in §0.

It is well known that complements in V∞ are not unique. For example, in [Re2] Remmel constructed r.e. spaces M1 and M2 and co-r.e. spaces Q1 and Q2 such that for all i, j ∈ {1, 2}, Mi ⊕ Qj = V∞ and M1 is supermaximal, M2 is not maximal, Q1 has a fully extendible basis, and Q2 has no extendible basis. We say a subspace Q of V∞ is fully co-r.e. if Q is generated by a co-r.e. subset of some recursive basis of V∞. Downey [Do2] has shown that every r.e. subspace of V∞ has a complement which is a fully co-r.e. subspace. Moreover suppose Q is any fully co-r.e. subspace, say Q = (C)* where C is a co-r.e. subset of a recursive basis B of V∞; if C is nonrecursive, then it is shown in [Do2] that Q has a decidable complement as well as a nondecidable nowhere simple complement.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 49 , Issue 4 , December 1984 , pp. 1125 - 1136
Copyright
Copyright © Association for Symbolic Logic 1984

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[AD]Ash, C. J. and Downey, R. G., Decidable subspaces and recursively enumerable subspaces, this Journal, Vol. 49 (1984), pp. 1137–1145.Google Scholar
[Do1]Downey, R. G., Abstract dependence, recursion theory and the lattice of recursively enumerable filters, Ph.D. Thesis, Monash University, Clayton, Victoria, Australia, 1982.Google Scholar
[Do2]Downey, R. G., Co-immune subspaces and complementation in V ∞, this Journal, vol. 49 (1984), pp. 528–538.Google Scholar
[Do3]Downey, R. G., On a question of A. Retzlaff, Zeitschrift für Mathematische Logik and Grundlagen der Mathematik, vol. 29 (1983), pp. 379–384.CrossRefGoogle Scholar
[DH]Downey, R. G. and Hird, G. R., Automorphisms of supermaximal subspaces, this Journal (to appear).Google Scholar
[DW]Downey, R. G. and Welch, L., Splitting properties and recursively enumerable degrees (in preparation).Google Scholar
[Gu]Guichard, D., Automorphisms of L(V ∞) (preprint).Google Scholar
[KR]Kalantari, I. and Retzlaff, A., Maximal vector spaces under automorphisms of the lattice of recursively enumerable vector spaces, this Journal, vol. 42 (1977), pp. 481–491.Google Scholar
[LR1]Lerman, M. and Remmel, J. B., The universal splitting property. I, Logic Colloquium '80 (van Dalen, D., Lascar, D. and Smiley, T. J., editors), Studies in Logic and the Foundations of Mathematics, vol. 108, North-Holland, New York, 1982, pp. 181–209.Google Scholar
[LR2]Lerman, M. and Remmel, J. B., The universal splitting property. II, this Journal, vol. 49 (1984), pp. 137–150.Google Scholar
[MN1]Metakides, G. and Nerode, A., Recursively enumerable vector spaces, Annals of Mathematical Logic, vol. 11 (1977), pp. 147–171.CrossRefGoogle Scholar
[MN2]Metakides, G. and Nerode, A., Recursion theory on fields and abstract dependence, Journal of Algebra, vol. 65 (1980), pp. 36–59.CrossRefGoogle Scholar
[MR]Miller, D. and Remmel, J. B., Effectively nowhere simple sets, this Journal, vol. 49 (1983), pp. 129–136.Google Scholar
[Re1]Remmel, J. B., Maximal and cohesive vector spaces, this Journal, vol. 42 (1977), pp. 400–418.Google Scholar
[Re2]Remmel, J. B., On r.e. and co-r.e. vector spaces with nonextendible bases, this Journal, vol. 45 (1980), pp. 20–34.Google Scholar
[Ro]Rogers, H. Jr., Theory of recursive functions and effective computability, McGraw-Hill, New York, 1967.Google Scholar
[Sh]Shore, R. A., Controlling the dependence degree of a recursively enumerable vector space, this Journal, vol. 43 (1978), pp. 13–23.Google Scholar
[So]Soare, R. I., The infinite injury priority method, this Journal, vol. 41 (1976), pp. 513–530.Google Scholar