Hostname: page-component-586b7cd67f-2brh9 Total loading time: 0 Render date: 2024-11-28T14:59:36.030Z Has data issue: false hasContentIssue false
  • English
  • Français

Scott incomplete Boolean ultrapowers of the real line

Published online by Cambridge University Press:  12 March 2014

Masanao Ozawa
Masanao Ozawa*
Affiliation:
Department of Mathematics, College of General Education, Nagoya University, Nagoya 464-01, Japan, E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

An ordered field is said to be Scott complete iff it is complete with respect to its uniform structure. Zakon has asked whether nonstandard real lines are Scott complete. We prove in ZFC that for any complete Boolean algebra B which is not (ω, 2)-distributive there is an ultrafilter 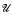of B such that the Boolean ultrapower of the real line modulo 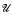is not Scott complete. We also show how forcing in set theory gives rise to examples of Boolean ultrapowers of the real line which are not Scott complete.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 60 , Issue 1 , March 1995 , pp. 160 - 171
Copyright
Copyright © Association for Symbolic Logic 1995

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Bell, J. L., Boolean-valued models and independence proofs in set theory, 2nd ed., Oxford University Press, Oxford, 1985.Google Scholar
[2]Chang, C. C. and Keisler, H. J., Model Theory, 3rd ed., North-Holland, Amsterdam, 1990.Google Scholar
[3]Cohen, L. W. and Goffman, C., The topology of ordered abelian groups, Transactions of the American Mathematical Society, vol. 67 (1949), pp. 310–319.CrossRefGoogle Scholar
[4]Kamo, S., Nonstandard natural number systems and nonstandard models, this Journal, vol. 46 (1981), pp. 365–376.Google Scholar
[5]Kamo, S., Non-standard real number systems with regular gaps, Tsukuba Journal of Mathematics, vol. 5 (1981), pp. 21–24.CrossRefGoogle Scholar
[6]Keisler, H. J. and Schmerl, J. H., Making the hyperreal line both saturated and complete, this Journal, vol. 56 (1991), pp. 1016–1025.Google Scholar
[7]Mansfield, R., The theory of Boolean ultrapowers, Annals of Mathematical Logic, vol. 2 (1971), pp. 297–323.CrossRefGoogle Scholar
[8]Ozawa, M., Forcing in nonstandard analysis, Annals of Pure and Applied Logic, vol. 68 (1994), pp. 263–297.CrossRefGoogle Scholar
[9]Robinson, A., Non-standard analysis, North-Holland, Amsterdam, 1966.Google Scholar
[10]Schmerl, J. H., Models of Peano arithmetic and a question of Sikorski on ordered fields, Israel Journal of Mathematics, vol. 50 (1985), pp. 145–159.CrossRefGoogle Scholar
[11]Scott, D., On completing ordered fields, Applications of model theory of algebra, analysis, and probability (Luxemburg, W. A. J., editor), Holt, Rinehart, and Winston, New York, 1969, pp. 274–278.Google Scholar
[12]Takeuti, G. and Zaring, W. M., Axiomatic set theory, Springer-Verlag, New York, 1973.CrossRefGoogle Scholar
[13]Zakon, E., Remarks on the nonstandard real axis, Applications of model theory to algebra, analysis, and probability (Luxemburg, W. A. J., editors), Holt, Rinehart, and Winston, New York, 1969, pp. 195–227.Google Scholar