Article contents
ON THE INEVITABILITY OF THE CONSISTENCY OPERATOR
Published online by Cambridge University Press: 14 March 2019
Abstract
We examine recursive monotonic functions on the Lindenbaum algebra of 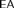 $EA$. We prove that no such function sends every consistent φ to a sentence with deductive strength strictly between φ and
$EA$. We prove that no such function sends every consistent φ to a sentence with deductive strength strictly between φ and 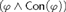 $\left( {\varphi \wedge Con\left( \varphi \right)} \right)$. We generalize this result to iterates of consistency into the effective transfinite. We then prove that for any recursive monotonic function f, if there is an iterate of
$\left( {\varphi \wedge Con\left( \varphi \right)} \right)$. We generalize this result to iterates of consistency into the effective transfinite. We then prove that for any recursive monotonic function f, if there is an iterate of  $Con$ that bounds f everywhere, then f must be somewhere equal to an iterate of
$Con$ that bounds f everywhere, then f must be somewhere equal to an iterate of 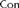 $Con$.
$Con$.
- Type
- Articles
- Information
- Copyright
- Copyright © The Association for Symbolic Logic 2019
References
REFERENCES
- 2
- Cited by


