No CrossRef data available.
Article contents
On the compactness of some Boolean algebras
Published online by Cambridge University Press: 12 March 2014
Extract
We say that the Boolean algebra B is λ-compact, where λ is a cardinal number, if for every family Z ⊆ B∖{0} of power at most λ, if inf Z = 0 then for some finite subfamily Z0 ⊆ Z we have inf Z0 = 0.
On the set of all finite subsets of a cardinal number κ, which is denoted [κ]<ω, the sets of the form 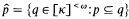 for any p Є [κ]<ω generate the filter Tκ.
for any p Є [κ]<ω generate the filter Tκ.
This filter is a standard example of a κ-regular filter (see [2]). Because of the importance of κ-regular filters in studying the saturatedness of ultraproducts and reduced products by model-theoretic methods, the question of compactness of the algebra Bκ = P([κ]<ω/Tκ was natural. This question in the most optimistical way was formulated by M. Benda [1, Problem 5c]: is the algebra Bκω-compact for every uncountable κ?
In this paper we show that for most of the cardinal numbers which are greater or equal to 2ω the algebra Bκ is not ω-compact. Hence, in view of obtained results, the following question appears: does there exist an uncountable κ such that the algebra Bκ is κ-compact?
We use standard set-theoretical notations. CH denotes the Continuum Hypothesis, GCH denotes the General Continuum Hypothesis and MA denotes Martin's Axiom.
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1984


