Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-28T17:16:37.784Z Has data issue: false hasContentIssue false
  • English
  • Français

On choice sequences determined by spreads

Published online by Cambridge University Press:  12 March 2014

Gerrit van der Hoeven  and
Ieke Moerdijk
Gerrit van der Hoeven
Affiliation:
Twente University of Technology, Enschede, The Netherlands
Ieke Moerdijk
Affiliation:
University of Amsterdam, Amsterdam, The Netherlands
Get access Rights & Permissions [Opens in a new window]

Extract

From the moment choice sequences appear in Brouwer's writings, they do so as elements of a spread. This led Kreisel to take the so-called axiom of spreaddata as the basic axiom in a formal theory of choice sequences (Kreisel [1965, pp. 133–136]). This axiom expresses the idea that to be given a choice sequence means to be given a spread to which the choice sequence belongs. Subsequently, however, it was discovered that there is a formal clash between this axiom and closure of the domain of choice sequences under arbitrary (lawlike) continuous operations (Troelstra [1968]). For this reason, the formal system CS was introduced (Kreisel and Troelstra [1970]), in which spreaddata is replaced by analytic data. In this system CS, the domain of choice sequences is closed under all continuous operations, and therefore it provides a workable basis for intuitionistic analysis. But the problem whether the axiom of spreaddata is compatible with closure of the domain of choice sequences under the continuous operations from a restricted class, which is still rich enough to validate the typical axioms of continuous choice, remained open. It is precisely this problem that we aim to discuss in this paper.

Recall that a spread is a (lawlike, inhabited) decidable subtree S of the tree N< N of all finite sequences, having all branches infinite:

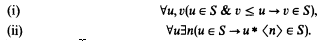
Type
Research Article
Information
The Journal of Symbolic Logic , Volume 49 , Issue 3 , September 1984 , pp. 908 - 916
Copyright
Copyright © Association for Symbolic Logic 1984

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

van Dalen, D. and Troelstra, A. S. [1970], Projections of lawlike sequences, Intuitionism and proof theory (Kino, A., Myhill, J. and Vesley, R. E., editors), North-Holland, Amsterdam, pp. 163–186.Google Scholar
Fourman, M. P. [1982], Notions of choice sequence, Proceedings of the Brouwer centenary conference (van Dalen, D. and Troelstra, A. S., editors), North-Holland, Amsterdam, pp. 91–105.Google Scholar
Kreisel, G. [1965], Mathematical logic, Lectures on modern mathematics, vol. III (Saaty, T. L., editor), Wiley, New York, pp. 95–195.Google Scholar
Kreisel, G. and Troelstra, A. S. [1970], Formal systems for some branches of intuitionistic analysis, Annals of Mathematical Logic, vol. 1, pp. 229–387.CrossRefGoogle Scholar
van der Hoeven, G. F. and Moerdijk, I. [1981], Sheaf models for choice sequences, preprint, University of Amsterdam, Amsterdam (to appear in Annals of Pure and Applied Logic).Google Scholar
Troelstra, A. S. [1968], The theory of choice sequences, Logic, methodology and philosophy of science. III (van Rootselaar, B. and Staal, J. F., editors), North-Holland, Amsterdam, pp. 201–223.CrossRefGoogle Scholar
Troelstra, A. S. [1970], Notes on the intuitionistic theory of sequences. II, Indagationes Mathematicae, vol. 32, pp. 99–109.CrossRefGoogle Scholar