Article contents
Cuts in hyperfinite time lines
Published online by Cambridge University Press: 12 March 2014
Abstract
In an ω1-saturated nonstandard universe a cut is an initial segment of the hyperinlegers which is closed under addition. Keisler and Leth in [KL] introduced, for each given cut U, a corresponding U-topology on the hyperintegers by letting O be U-open if for any x ϵ O there is a y greater than all the elements in U such that the interval [x − y, x + y] ⊆ O. Let U be a cut in a hyperfinite time line 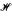 , which is a hyperfinite initial segment of the hyperintegers. U is called a good cut if there exists a U-meager subset of
, which is a hyperfinite initial segment of the hyperintegers. U is called a good cut if there exists a U-meager subset of 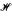 of Loeb measure one. Otherwise U is bad. In this paper we discuss the questions of Keisler and Leth about the existence of bad cuts and related cuts. We show that assuming b > ω1, every hyperfinite time line has a cut with both cofinality and coinitiality uncountable. We construct bad cuts in a nonstandard universe under ZFC. We also give two results about the existence of other kinds of cuts.
of Loeb measure one. Otherwise U is bad. In this paper we discuss the questions of Keisler and Leth about the existence of bad cuts and related cuts. We show that assuming b > ω1, every hyperfinite time line has a cut with both cofinality and coinitiality uncountable. We construct bad cuts in a nonstandard universe under ZFC. We also give two results about the existence of other kinds of cuts.
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1992
References
REFERENCES
- 3
- Cited by


