Accessible sets and (L_ω1ω)_t-equivalence for T₃ spaces

Extract

Ziegler studies in [2] the expressive power of (L_ωω)_t for T₃ topological spaces. He defines for every natural number n the set 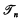of n-types by induction: 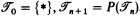. If A is a T₃ space, the n-type of a ∈ A is defined inductively by: 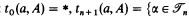: in every neighborhood of a there is an a′ ≠ a with t_n(a′, A) = α}. These types are (L_ωω)_t-definable. Then, it is shown that two T₃ spaces are (L_ωω)_t-equivalent precisely if for every n-type α they have the same number of points with n-type α (cf. [2]).

In order to study the expressability of (L_ω1ω)_t, for T₃ spaces, we introduce in this paper the notion of accessible set. Looking at the behaviour of convergence by means of this notion, we refine Ziegler's notion of n-type and introduce a new set S_n of n-types, which are (L_ω1ω)_t-definable. Then, we prove a characterization of (L_ω1ω)_t-equivalence for a wide class of T₃ spaces. A T₃ space A belongs to this class if there is a κ ∈ ω such that, for every n ∈ ω, there are at most κ n-types in S_n which are satisfiable in A. Such a space is said to be of a-finite type. Some relations between these spaces and the spaces of finite type in the sense of [2] are shown in the last section.

The contents of the present paper are treated in more detail in [3].