Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-25T07:04:57.097Z Has data issue: false hasContentIssue false
  • English
  • Français

Linear stability of exponential density profiles

Published online by Cambridge University Press:  13 March 2009

R. Croci  and
R. Saison
R. Croci
Affiliation:
Institut für Plasmaphysik, Garching bei München, Germany
R. Saison
Affiliation:
Institut für Plasmaphysik, Garching bei München, Germany
Get access Rights & Permissions [Opens in a new window]

Abstract

The problem of the linear stability of a plasma with density profiles

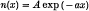
in a homogeneous magnetic field B = îzB0 has been solved exactly. The dispersion relation leads to stability criteria less severe than those obtained in the local approximation. As examples, we consider Bernstein waves and the low-frequency drift instability. In the second example the marginal stability curve

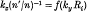
is given for different values of the parameters kx, Ri, Ri n′/n, with Ti = Te. Further, the same method is applied to a plasma with equilibrium distribution function in a linear electric potential φ = αx and a homogeneous magnetic field B = îxB0.

Type
Research Article
Information
Journal of Plasma Physics , Volume 5 , Issue 3 , June 1971 , pp. 375 - 383
Copyright
Copyright © Cambridge University Press 1971

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Croci, R. & Saison, R. 1969 Institut für Plasmaphysik, Garching bei München, Rept. IPP 6–82.Google Scholar
Davidson, J. N. & Kammash, T. 1968 Nucl. Fus. 8, 203.Google Scholar
Galeev, A. A. 1964 Soviet Phys. Doklady 8, 444.Google Scholar
Hoh, F. C. 1965 Phys. Fluids 8, 1741.CrossRefGoogle Scholar
Horton, C. W. 1968 Phys. Fluids 11, 1154.CrossRefGoogle Scholar
Mikhailovsky, A. B. 1967 Reviews of Plasmaphysics 3 (Consultants Bureau).Google Scholar
Pearlstein, L. D. 1965 Phys. Fluids 8, 1743.CrossRefGoogle Scholar
Rutherford, P. H. & Frieman, E. A. 1968 Phys. Fluids 11, 569.Google Scholar