Article contents
Effect of relativistic self-focusing on plasma wave excitation by a hollow Gaussian beam
Published online by Cambridge University Press: 01 June 2011
Abstract
A paraxial-like approach has been invoked to understand the nature of propagation of a hollow Gaussian beam (HGB) propagating in plasma under the influence of relativistic non-linearity. In this approach, the parameters are expanded in terms of the radial distance from the maximum of irradiance rather than that from the axis. This paper investigates the excitation of plasma wave in a hot collision less plasma by HGB. On account of the 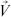 ×
× 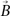 force, a plasma wave at 2ω0 (here, ω0 is the pump laser frequency) is generated. The solution of the HGB has been obtained within the paraxial ray approximation. Filamentary structures of the laser beam are observed due to relativistic non-linearity.
force, a plasma wave at 2ω0 (here, ω0 is the pump laser frequency) is generated. The solution of the HGB has been obtained within the paraxial ray approximation. Filamentary structures of the laser beam are observed due to relativistic non-linearity.
- Type
- Papers
- Information
- Copyright
- Copyright © Cambridge University Press 2011
References
- 7
- Cited by


