Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-25T06:11:11.613Z Has data issue: false hasContentIssue false
  • English
  • Français

A collisionless shock in a plasma which is almost stable

Published online by Cambridge University Press:  13 March 2009

A. Smith
A. Smith
Affiliation:
Department of Mathematics, University of the West Indies, Jamaica
Get access Rights & Permissions [Opens in a new window]

Abstract

The possibility of a collisionless shock in a plasma not subject to electrostatic instabilities nor immersed in a permanent magnetic field is investigated. The equations governing a steady shock in an electron-ion plasma are deduced and their limitations are discussed. Attention is given to the fact that the velocity distribution in a collisionless plasma may not be Maxwellian. This yields a parameter which is a measure of high-energy particle density. It is found that almost always no steady shock exists. The inverse of the electron plasma wave-number k0=ωp/c provides a scale length for the shock, though the dampening effect of the ions indicates a shock thickness much in excess of 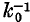. It is concluded that the mechanism for such shocks may be important in a gas such as the solar wind.

Type
Research Article
Information
Journal of Plasma Physics , Volume 3 , Issue 4 , December 1969 , pp. 525 - 541
Copyright
Copyright © Cambridge University Press 1969

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Gbad, H. 1949 Comm. Pure Appl. Math. 2, 331.Google Scholar
Huang, K. 1963 Statistical Mechanics. Wiley.Google Scholar
Kahn, F. D. 1964 J. Fluid Mech. 19, 210.CrossRefGoogle Scholar
Landatj, L. D. & Lipshitz, E. M. 1963 Fluid Mechanics. Pergamon.Google Scholar
Noebdlingeb, P. D. 1961 Ap. J. 133, 1034.Google Scholar
Paekeb, E. N. 1963 Interplanetary Dynamical Processes. Interseience.Google Scholar
Smith, A. 1969a J. Plasma Phys. 3, 281.CrossRefGoogle Scholar
Smith, A. 1969b J. Plasma Phys. 3, 295.CrossRefGoogle Scholar
Sonnet, C. P. et al. 1964 Phys. Rev. Lett. 13, 153.CrossRefGoogle Scholar
Weibel, E. S. 1959 Phvs. Rev. Lett. 22, 83.CrossRefGoogle Scholar