Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-26T09:20:26.837Z Has data issue: false hasContentIssue false
  • English
  • Français

Size-dependent vibration analysis of carbon nanotubes

Published online by Cambridge University Press:  21 January 2019

Wu-Rong Jian ,
Xiaohu Yao Open the ORCID record for Xiaohu Yao [Opens in a new window] ,
Yugang Sun ,
Zhuocheng Xie  and
Xiaoqing Zhang
Wu-Rong Jian
Affiliation:
Department of Engineering Mechanics, South China University of Technology, Guangzhou, Guangdong 510640, People’s Republic of China
Xiaohu Yao*
Affiliation:
Department of Engineering Mechanics, South China University of Technology, Guangzhou, Guangdong 510640, People’s Republic of China
Yugang Sun
Affiliation:
Department of Engineering Mechanics, South China University of Technology, Guangzhou, Guangdong 510640, People’s Republic of China
Zhuocheng Xie
Affiliation:
Department of Engineering Mechanics, South China University of Technology, Guangzhou, Guangdong 510640, People’s Republic of China
Xiaoqing Zhang
Affiliation:
Department of Engineering Mechanics, South China University of Technology, Guangzhou, Guangdong 510640, People’s Republic of China
*
a)Address all correspondence to this author. e-mail: [email protected]
Get access

Abstract

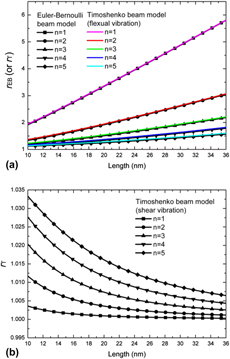
Considering the nonlocal small-scale effect and surface effect, we perform the size-dependent vibration analysis of carbon nanotube (CNT). The modified governing equations for CNT’s vibration behaviors are derived by using the nonlocal Euler–Bernoulli and Timoshenko beam models, together with the consideration of surface tension and surface elasticity. According to the numerical experiments, both small-scale effect and surface effect make a substantial difference. For flexural vibration, size effect for CNT’s vibration behaviors weakens with the increase of its diameter, but strengthens with the increase of the length–diameter ratio; for shear vibration with constant length–diameter ratio, a nonlinear correlation between size effect and CNT’s diameter exists, suggesting that there is a typical diameter for CNTs, which corresponds to the “strongest” size effect. In addition, the effects of elastic substrate modulus, temperature change, and axial preloading on the vibration behaviors and their size-dependence are analyzed, respectively.

Keywords

microelectromechanical (MEMS)nanoscalenanostructure
Type
Invited Paper
Information
Journal of Materials Research , Volume 34 , Issue 13: Focus Issue: Intrinsic and Extrinsic Size Effects in Materials , 15 July 2019 , pp. 2148 - 2160
Copyright
Copyright © Materials Research Society 2019 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Li, F., Cheng, H.M., Bai, S., Su, G., and Dresselhaus, M.S.: Tensile strength of single-walled carbon nanotubes directly measured from their macroscopic ropes. Appl. Phys. Lett. 77, 3161–3163 (2000).CrossRefGoogle Scholar
Liu, K., Sun, Y., Zhou, R., Zhu, H., Wang, J., Liu, L., Fan, S., and Jiang, K.: Carbon nanotube yarns with high tensile strength made by a twisting and shrinking method. Nanotechnology 21, 045708 (2010).CrossRefGoogle ScholarPubMed
Zhang, X., Li, Q., Holesinger, T.G., Arendt, P.N., Huang, J., Kirven, P.D., Clapp, T.G., Depaula, R.F., Liao, X., and Zhao, Y.: Ultrastrong, stiff, and lightweight carbon-nanotube fibers. Adv. Mater. 19, 4198–4201 (2010).CrossRefGoogle Scholar
Treacy, M.M.J., Ebbesen, T.W., and Gibson, J.M.: Exceptionally high Young’s modulus observed for individual carbon nanotubes. Nature 381, 678–680 (1996).CrossRefGoogle Scholar
Jorio, A., Dresselhaus, G., and Dresselhaus, M.S.: Carbon nanotubes: Advanced topics in the synthesis, structure, properties and applications. Mater. Today 11, 52–60 (2008).Google Scholar
Li, C. and Chou, T.W.: Single-walled carbon nanotubes as ultrahigh frequency nanomechanical resonators. Phys. Rev. B 68, 338–344 (2003).CrossRefGoogle Scholar
Li, C. and Chou, T.W.: Mass detection using carbon nanotube-based nanomechanical resonators. Appl. Phys. Lett. 84, 5246–5248 (2004).CrossRefGoogle Scholar
Lohrasebi, A. and Rafii-Tabar, H.: Computational modeling of an ion-driven nanomotor. J. Mol. Graphics Modell. 27, 116–123 (2008).CrossRefGoogle ScholarPubMed
Han, J., Globus, A., Jaffe, R., and Deardorff, G.: Molecular dynamics simulations of carbon nanotube-based gears. Nanotechnology 8, 95–102 (1997).CrossRefGoogle Scholar
Fennimore, A.M., Yuzvinsky, T.D., Han, W.Q., Fuhrer, M.S., Cumings, J., and Zettl, A.: Rotational actuators based on carbon nanotubes. Nature 424, 408 (2003).CrossRefGoogle ScholarPubMed
Durov, S.S. and Ogloblya, O.V.: Molecular dynamics simulation of mechanical, vibrational and electronic properties of carbon nanotubes. Comput. Mater. Sci. 17, 352–355 (2000).Google Scholar
Duan, W.H., Wang, C.M., and Zhang, Y.Y.: Calibration of nonlocal scaling effect parameter for free vibration of carbon nanotubes by molecular dynamics. J. Appl. Phys. 101, 195412–196312 (2007).CrossRefGoogle Scholar
Wang, L., Hu, H., and Guo, W.: Thermal vibration of carbon nanotubes predicted by beam models and molecular dynamics. Proc. R. Soc. London, Ser. A 466, 2325–2340 (2010).CrossRefGoogle Scholar
Ansari, R., Ajori, S., and Arash, B.: Vibrations of single- and double-walled carbon nanotubes with layerwise boundary conditions: A molecular dynamics study. Curr. Appl. Phys. 12, 707–711 (2012).CrossRefGoogle Scholar
Chang, I.: Molecular dynamics investigation of carbon nanotube resonance. Modell. Simul. Mater. Sci. Eng. 21, 045011 (2013).CrossRefGoogle Scholar
Liu, R. and Wang, L.: Thermal vibration of a single-walled carbon nanotube predicted by semiquantum molecular dynamics. Phys. Chem. Chem. Phys. 17, 5194–5201 (2015).CrossRefGoogle ScholarPubMed
Zhang, Y.Q., Liu, G.R., and Xie, X.Y.: Free transverse vibrations of double-walled carbon nanotubes using a theory of nonlocal elasticity. Phys. Rev. B 71, 195404 (2005).CrossRefGoogle Scholar
Wang, Q. and Varadan, V.K.: Vibration of carbon nanotubes studied using nonlocal continuum mechanics. Smart Mater. Struct. 15, 659 (2006).CrossRefGoogle Scholar
Wang, C.M., Tan, V.B.C., and Zhang, Y.Y.: Timoshenko beam model for vibration analysis of multi-walled carbon nanotubes. J. Sound Vib. 294, 1060–1072 (2006).CrossRefGoogle Scholar
Lu, P., Lee, H.P., Lu, C., and Zhang, P.Q.: Application of nonlocal beam models for carbon nanotubes. Int. J. Solid Struct. 44, 5289–5300 (2007).CrossRefGoogle Scholar
Ansari, R. and Sahmani, S.: Small scale effect on vibrational response of single-walled carbon nanotubes with different boundary conditions based on nonlocal beam models. Commun. Nonlinear Sci. Numer. Simulat. 17, 1965–1979 (2012).CrossRefGoogle Scholar
Yoon, J., Ru, C.Q., and Mioduchowski, A.: Vibration of an embedded multiwall carbon nanotube. Compos. Sci. Technol. 63, 1533–1542 (2003).CrossRefGoogle Scholar
Pradhan, S.C. and Murmu, T.: Small-scale effect on vibration analysis of single-walled carbon nanotubes embedded in an elastic medium using nonlocal elasticity theory. J. Appl. Phys. 105, 56 (2009).CrossRefGoogle Scholar
Mustapha, K.B. and Zhong, Z.W.: Free transverse vibration of an axially loaded non-prismatic single-walled carbon nanotube embedded in a two-parameter elastic medium. Comput. Mater. Sci. 50, 742–751 (2011).CrossRefGoogle Scholar
Benzair, A., Tounsi, A., Besseghier, A., Heireche, H., Moulay, N., and Boumia, L.: The thermal effect on vibration of single-walled carbon nanotubes using nonlocal Timoshenko beam theory. J. Phys. D: Appl. Phys. 41, 225404 (2008).CrossRefGoogle Scholar
Zhang, Y., Liu, G., and Xu, H.: Transverse vibrations of double-walled carbon nanotubes under compressive axial load. Phys. Lett. A 340, 258–266 (2005).CrossRefGoogle Scholar
Murmu, T. and Adhikari, S.: Scale-dependent vibration analysis of prestressed carbon nanotubes undergoing rotation. J. Appl. Phys. 108, 250 (2010).CrossRefGoogle Scholar
Tersoff, J.: Modeling solid-state chemistry: Interatomic potentials for multicomponent systems. Phys. Rev. B 39, 5566–5568 (1989).CrossRefGoogle ScholarPubMed
Brenner, D.W., Shenderova, O.A., Harrison, J.A., Stuart, S.J., Ni, B., and Sinnott, S.B.: A second-generation reactive empirical bond order (REBO) potential energy expression for hydrocarbons. J. Phys.: Condens. Matter 14, 783 (2002).Google Scholar
Stuart, S.J., Tutein, A.B., and Harrison, J.A.: A reactive potential for hydrocarbons with intermolecular interactions. J. Chem. Phys. 112, 6472–6486 (2000).CrossRefGoogle Scholar
Eringen, A.C.: On differential equations of nonlocal elasticity and solutions of screw dislocation and surface waves. J. Appl. Phys. 54, 4703–4710 (1998).CrossRefGoogle Scholar
Zhang, X.Q., Yao, X.H., and Sun, Y.G.: Scale effect on flexural wave propagation in embedded single-walled carbon nanotubes: Nonlocal small-size effect and surface effect. J. Comput. Theor. Nanosci. 12, 2356–2365 (2015).Google Scholar
Zhang, Y.Q., Liu, X., and Zhao, J.H.: Influence of temperature change on column buckling of multiwalled carbon nanotubes. Phys. Lett. A 372, 1676–1681 (2008).CrossRefGoogle Scholar
Arash, B. and Wang, Q.: A review on the application of nonlocal elastic models in modeling of carbon nanotubes and graphenes. Comput. Mater. Sci. 51, 303–313 (2012).CrossRefGoogle Scholar
Sun, Y.G., Yao, X.H., Liang, Y.J., and Han, Q.: Nonlocal beam model for axial buckling of carbon nanotubes with surface effect. Europhys. Lett. 99, 56007 (2012).CrossRefGoogle Scholar
Yao, X.H. and Sun, Y.G.: Combined bending stability of carbon nanotubes subjected to thermo-electro-mechanical loadings. Comput. Mater. Sci. 54, 135–144 (2012).CrossRefGoogle Scholar
Yao, X.H. and Han, Q.: Investigation of axially compressed buckling of a multi-walled carbon nanotube under temperature field. Compos. Sci. Technol. 67, 125–134 (2007).Google Scholar
Supplementary material: File

Jian et al. supplementary material

Jian et al. supplementary material 1

Download Jian et al. supplementary material(File)
File 17.6 MB