Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-28T12:53:53.923Z Has data issue: false hasContentIssue false
  • English
  • Français

The local stress state of a running shear band in amorphous solids

Published online by Cambridge University Press:  27 May 2015

Jian Luo  and
Yunfeng Shi
Jian Luo
Affiliation:
Department of Materials Science and Engineering, Rensselaer Polytechnic Institute, Troy, New York 12180, USA
Yunfeng Shi*
Affiliation:
Department of Materials Science and Engineering, Rensselaer Polytechnic Institute, Troy, New York 12180, USA
*
a)Address all correspondence to this author. e-mail: [email protected]
Get access

Abstract

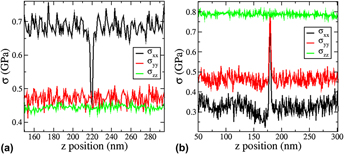
In molecular dynamics simulations, the local stress state in the shear band is examined in six different model metallic glasses and one amorphous Si system (also has been perceived as a metallic glass analog) under different loading conditions. For all but the FeP and the amorphous Si systems, the running shear band (RSB) exhibits a liquid-like hydrostatic plus shear stress state. Our results suggest that the liquid feature of a RSB is not due to temperature rise or plastic confinement but due to the disorder driven by flow, which can be offset by strong directionality in bonding, phase segregation, or aging. The knowledge of the liquid-like stress state can be conveniently utilized in experiments to infer the local stress state of the RSB from the global tensile stress for metallic glasses.

Keywords

molecular dynamicsmetallic glassesshear bandsamorphous solidsstress state
Type
Articles
Information
Journal of Materials Research , Volume 30 , Issue 12 , 28 June 2015 , pp. 1979 - 1987
Copyright
Copyright © Materials Research Society 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Schuh, C., Hufnagel, T., and Ramamurty, U.: Mechanical behavior of amorphous alloys. Acta Mater. 55, 4067 (2007).Google Scholar
Greer, A.L., Cheng, Y.Q., and Ma, E.: Shear bands in metallic glasses. Mater. Sci. Eng., R 74, 71 (2013).Google Scholar
Cao, A.J., Cheng, Y.Q., and Ma, E.: Structural processes that initiate shear localization in metallic glass. Acta Mater. 57, 5146 (2009).Google Scholar
Cheng, Y., Han, Z., Li, Y., and Ma, E.: Cold versus hot shear banding in bulk metallic glass. Phys. Rev. B 80, 134115 (2009).Google Scholar
Polk, D.E. and Turnbull, D.: Flow of melt and glass forms of metallic alloys. Acta Metall. 20, 493 (1972).Google Scholar
Pampillo, C.A.: Localized shear deformation in a glassy metal. Scr. Metall. 6, 915 (1972).Google Scholar
Pampillo, C.A. and Chen, H.S.: Comprehensive plastic deformation of a bulk metallic glass. Mater. Sci. Eng. 13, 181 (1974).Google Scholar
Lewandowski, J.J. and Greer, A.L.: Temperature rise at shear bands in metallic glasses. Nat. Mater. 5, 15 (2005).Google Scholar
Zhang, Y. and Greer, A.L.: Thickness of shear bands in metallic glasses. Appl. Phys. Lett. 89, 071907 (2006).Google Scholar
Shi, Y. and Falk, M.: Atomic-scale simulations of strain localization in three-dimensional model amorphous solids. Phys. Rev. B 73, 214201 (2006).CrossRefGoogle Scholar
Shimizu, F., Ogata, S., and Li, J.: Yield point of metallic glass. Acta Mater. 54, 4293 (2006).Google Scholar
Shi, Y. and Falk, M.L.: Stress-induced structural transformation and shear banding during simulated nanoindentation of a metallic glass. Acta Mater. 55(13), 4317 (2007).Google Scholar
Cheng, Y.Q. and Ma, E.: Atomic-level structure and structure–property relationship in metallic glasses. Prog. Mater. Sci. 56, 379 (2011).Google Scholar
Jiang, W.H., Pinkerton, F.E., and Atzmon, M.: Mechanical behavior of shear bands and the effect of their relaxation in a rolled amorphous Al-based alloy. Acta Mater. 53, 3469 (2005).Google Scholar
Li, J., Wang, Z., and Hufnagel, T.: Characterization of nanometer-scale defects in metallic glasses by quantitative high-resolution transmission electron microscopy. Phys. Rev. B 65, 144201 (2002).Google Scholar
Yang, B., Morrison, M.L., Liaw, P.K., Buchanan, R.A., Wang, G., Liu, C.T., and Denda, M.: Dynamic evolution of nanoscale shear bands in a bulk-metallic glass. Appl. Phys. Lett. 86, 141904 (2005).Google Scholar
Heggen, M., Spaepen, F., and Feuerbacher, M.: Creation and annihilation of free volume during homogeneous flow of a metallic glass. J. Appl. Phys. 97, 033506 (2005).Google Scholar
Spaepen, F.: A microscopic mechanism for steady state inhomogeneous flow in metallic glasses. Acta Metall. 25, 407 (1977).Google Scholar
Shimizu, F., Ogata, S., and Li, J.: Theory of shear banding in metallic glasses and molecular dynamics calculations. Mater. Trans. 48, 2923 (2007).Google Scholar
Klaumünzer, D., Maaß, R., and Löffler, J.F.: Stick-slip dynamics and recent insights into shear banding in metallic glasses. J. Mater. Res. 26, 1453 (2011).Google Scholar
Song, S.X. and Nieh, T.G.: Direct measurements of shear band propagation in metallic glasses—An overview. Intermetallics 19, 1968 (2011).Google Scholar
Liu, Y., Liu, C., Wang, W., Inoue, A., Sakurai, T., and Chen, M.: Thermodynamic origins of shear band formation and the universal scaling law of metallic glass strength. Phys. Rev. Lett. 103, 065504 (2009).Google Scholar
Guan, P., Chen, M., and Egami, T.: Stress-temperature scaling for steady-state flow in metallic glasses. Phys. Rev. Lett. 104, 205701 (2010).Google Scholar
Langer, J. and Manning, M.: Steady-state, effective-temperature dynamics in a glassy material. Phys. Rev. E 76, 056107 (2007).Google Scholar
Shi, Y., Katz, M., Li, H., and Falk, M.: Evaluation of the disorder temperature and free-volume formalisms via simulations of shear banding in amorphous solids. Phys. Rev. Lett. 98, 185505 (2007).Google Scholar
Spaepen, F.: Metallic glasses: Must shear bands be hot? Nat. Mater. 5, 7 (2006).Google Scholar
Murali, P., Narasimhan, R., Guo, T.F., Zhang, Y.W., and Gao, H.J.: Shear bands mediate cavitation in brittle metallic glasses. Scr. Mater. 68, 567 (2013).Google Scholar
Fujita, K., Okamoto, A., Nishiyama, N., Yokoyama, Y., Kimura, H., and Inoue, A.: Effects of loading rates, notch root radius and specimen thickness on fracture toughness in bulk metallic glasses. J. Alloys Compd. 434–435, 22 (2007).Google Scholar
Luo, J. and Shi, Y.: Tensile fracture of metallic glasses via shear band cavitation. Acta Mater. 82, 483 (2015).Google Scholar
Shi, Y., Luo, J., Yuan, F., and Huang, L.: Intrinsic ductility of glassy solids. J. Appl. Phys. 115, 043528 (2014).Google Scholar
Gao, Y.F., Wang, L., Bei, H., and Nieh, T.G.: On the shear-band direction in metallic glasses. Acta Mater. 59, 4159 (2011).Google Scholar
Nosé, S.: A unified formulation of the constant temperature molecular dynamics methods. J. Chem. Phys. 81, 511 (1984).Google Scholar
Hoover, W.: Canonical dynamics: Equilibrium phase-space distributions. Phys. Rev. A 31, 1695 (1985).Google Scholar
Argon, A.S. and Demkowicz, M.J.: What can plasticity of amorphous silicon tell us about plasticity of metallic glasses? Metall. Mater. Trans. A 39, 1762 (2008).Google Scholar
Kob, W.: Testing mode-coupling theory for a supercooled binary Lennard-Jones mixture I: The van Hove correlation function. Phys. Rev. E 51, 4626 (1995).Google Scholar
Wahnström, G.: Molecular-dynamics study of a supercooled two-component Lennard-Jones system. Phys. Rev. A 44(6), 3752 (1991).Google Scholar
Mendelev, M.I., Sordelet, D.J., and Kramer, M.J.: Using atomistic computer simulations to analyze x-ray diffraction data from metallic glasses. J. Appl. Phys. 102, 043501 (2007).Google Scholar
Cheng, Y., Ma, E., and Sheng, H.: Atomic level structure in multicomponent bulk metallic glass. Phys. Rev. Lett. 102, 245501 (2009).Google Scholar
Ackland, G.J., Mendelev, M.I., Srolovitz, D.J., Han, S., and Barashev, A.V.: Development of an interatomic potential for phosphorus impurities in -iron. J. Phys.: Condens. Matter 16, S2629 (2004).Google Scholar
Stillinger, F.H. and Weber, T.A.: Computer simulation of local order in condensed phases of silicon. Phys. Rev. B 31(8), 5262 (1985).Google Scholar
Plimpton, S.: Fast parallel algorithms for short-range molecular dynamics. J. Comput. Phys. 117, 1 (1995).Google Scholar
Demkowicz, M. and Argon, A.: High-density liquidlike component facilitates plastic flow in a model amorphous silicon system. Phys. Rev. Lett. 93, 025505 (2004).Google Scholar
Molinero, V., Sastry, S., and Angell, C.A.: Tuning of tetrahedrality in a silicon potential yields a series of monatomic (metal-like) glass formers of very high fragility. Phys. Rev. Lett. 97, 075701 (2006).Google Scholar
Varias, A.G., Suo, Z., and Shih, C.F.: Ductile failure of a constrained metal foil. J. Mech. Phys. Solids 39, 963 (1991).Google Scholar
Tvergaard, V.: Failure by ductile cavity growth at a metal-ceramic interface. Acta Metall. Mater. 39, 419 (1991).Google Scholar
He, M.Y., Evans, A.G., and Hutchinson, J.W.: Interface cracking phenomena in constrained metal layers. Acta Mater. 44, 2963 (1996).Google Scholar
Shi, Y. and Falk, M.: Strain localization and percolation of stable structure in amorphous solids. Phys. Rev. Lett. 95, 095502 (2005).Google Scholar