Article contents
Spaces of sections of Banach algebra bundles
Published online by Cambridge University Press: 04 April 2012
Abstract
Suppose that B is a G-Banach algebra over 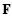 = ℝ or ℂ X is a finite dimensional compact metric space, ζ : P → X is a standard principal G-bundle, and Aζ = Γ(X,P ×GB) is the associated algebra of sections. We produce a spectral sequence which converges to π*(GLoAζ) with
= ℝ or ℂ X is a finite dimensional compact metric space, ζ : P → X is a standard principal G-bundle, and Aζ = Γ(X,P ×GB) is the associated algebra of sections. We produce a spectral sequence which converges to π*(GLoAζ) with
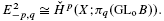
A related spectral sequence converging to K*+1(Aζ) (the real or complex topological K-theory) allows us to conclude that if B is Bott-stable, (i.e., if π*(GLoB) → K*+1(B) is an isomorphism for all * > 0) then so is Aζ.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © ISOPP 2012
References
REFERENCES
- 5
- Cited by


