No CrossRef data available.
Article contents
Uniqueness of Moore's higher reciprocity law at the prime 2 for real number fields
Published online by Cambridge University Press: 07 January 2008
Abstract
Let F be a real number field with r1 real embeddings. In this paper, we prove that the sequence
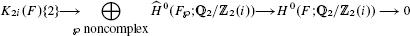
is a complex, where 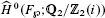 are the Tate cohomology groups. Moreover if i ≡ 0, 1, or 2 (mod 4), then it is exact; if i ≡ 3 (mod 4), then the homology group at the second term of this complex is isomorphic to
are the Tate cohomology groups. Moreover if i ≡ 0, 1, or 2 (mod 4), then it is exact; if i ≡ 3 (mod 4), then the homology group at the second term of this complex is isomorphic to 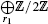 .
.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © ISOPP 2008
References
1.Banaszak, G., Generalization of the Moore exact sequence and the wild kernel for higher K-groups, Compositio Math. 86 (1993), 281–305Google Scholar
2.Kahn, B., Algebraic K-theory and twisted reciprocity laws, K-Theory 30 (2003), 211–240CrossRefGoogle Scholar
3.Levine, M., K-theory and motivic cohomology of schemes, UIUC K-Theory preprints Archives, No. 336Google Scholar
4.Milnor, J., Introduction to algebraic K-theory, Annals of Mathematics Studies 72, Princeton University Press, 1971.Google Scholar
5.Moore, C., Group extensions of p-adic and adelic linear groups, I. H. E. S. Publ. Math. No. 35 (1968), 157–222Google Scholar
6.Rognes, J. and Weibel, C., Two-primary algebraic K-theory of rings of integers in number fields, J. Amer. Math. Soc. 13 (2000) no. 1, 1–54CrossRefGoogle Scholar
7.Tate, J., Duality theorems in Galois cohomology over number fields, 1963 Proc. Internat. Congr. Mathematicians (Stockholm, 1962), pp. 288–295Google Scholar


