Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-27T21:23:11.909Z Has data issue: false hasContentIssue false
  • English
  • Français

Weakly nonlinear analysis of the viscoelastic instability in channel flow for finite and vanishing Reynolds numbers

Published online by Cambridge University Press:  08 April 2022

Gergely Buza Open the ORCID record for Gergely Buza [Opens in a new window] ,
Jacob Page Open the ORCID record for Jacob Page [Opens in a new window]  and
Rich R. Kerswell
Gergely Buza*
Affiliation:
Department of Applied Mathematics and Theoretical Physics, University of Cambridge, CB3 0WA, UK
Jacob Page*
Affiliation:
School of Mathematics, University of Edinburgh, EH9 3FD, UK
Rich R. Kerswell*
Affiliation:
Department of Applied Mathematics and Theoretical Physics, University of Cambridge, CB3 0WA, UK
*
†Email addresses for correspondence: [email protected], [email protected], [email protected]
†Email addresses for correspondence: [email protected], [email protected], [email protected]
†Email addresses for correspondence: [email protected], [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

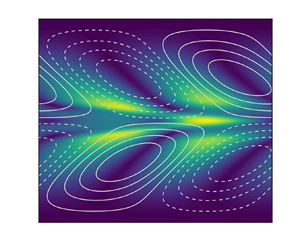
The recently discovered centre-mode instability of rectilinear viscoelastic shear flow (Garg et al., Phys. Rev. Lett., vol. 121, 2018, 024502) has offered an explanation for the origin of elasto-inertial turbulence that occurs at lower Weissenberg numbers ($Wi$). In support of this, we show using weakly nonlinear analysis that the subcriticality found in Page et al. (Phys. Rev. Lett., vol. 125, 2020, 154501) is generic across the neutral curve with the instability becoming supercritical only at low Reynolds numbers ($Re$) and high $Wi$. We demonstrate that the instability can be viewed as purely elastic in origin, even for $Re=O(10^3)$, rather than ‘elasto-inertial’, as the underlying shear does not feed the kinetic energy of the instability. It is also found that the introduction of a realistic maximum polymer extension length, $L_{max}$, in the FENE-P model moves the neutral curve closer to the inertialess $Re=0$ limit at a fixed ratio of solvent-to-solution viscosities, $\beta$. At $Re=0$ and in the dilute limit ($\beta \rightarrow 1$) with $L_{max} =O(100)$, the linear instability can be brought down to more physically relevant $Wi\gtrsim 110$ at $\beta =0.98$, compared with the threshold $Wi=O(10^3)$ at $\beta =0.994$ reported recently by Khalid et al. (Phys. Rev. Lett., vol. 127, 2021, 134502) for an Oldroyd-B fluid. Again, the instability is subcritical, implying that inertialess rectilinear viscoelastic shear flow is nonlinearly unstable – i.e. unstable to finite-amplitude disturbances – for even lower $Wi$.

JFM classification

Non-Newtonian Flows: Viscoelasticity Nonlinear Dynamical Systems: Bifurcation
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 940 , 10 June 2022 , A11
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Agarwal, A., Brandt, L. & Zaki, T.A. 2014 Linear and nonlinear evolution of a localized disturbance in polymeric channel flow. J. Fluid Mech. 760, 278–303.CrossRefGoogle Scholar
Buza, G., Beneitez, M., Page, J. & Kerswell, R.R. 2022 Finite-amplitude elastic waves in viscoelastic channel flow from large to zero Reynolds number. arXiv:2202.08047.Google Scholar
Chandra, B., Shankar, V. & Das, D. 2018 Onset of transition in the flow of polymer solutions in microtubes. J. Fluid Mech. 844, 1052–1083.CrossRefGoogle Scholar
Chaudhary, I., Garg, P., Shankar, V. & Subramanian, G. 2019 Elasto-inertial wall mode instabilities in viscoelastic plane Poiseuille flow. J. Fluid Mech. 881, 119–163.CrossRefGoogle Scholar
Chaudhary, I., Garg, P., Subramanian, G. & Shankar, V. 2021 Linear instability of viscoelastic pipe flow. J. Fluid Mech. 908, A11.CrossRefGoogle Scholar
Choueiri, G.H., Lopez, J.M. & Hof, B. 2018 Exceeding the asymptotic limit of polymer drag reduction. Phys. Rev. Lett. 120, 124501.CrossRefGoogle ScholarPubMed
Choueiri, G.H., Lopez, J.M., Varshey, A., Sankar, S. & Hof, B. 2021 Experimental observation of the origin and structure of elasto-inertial turbulence. Proc. Natl Acad. Sci. USA 118, e2102350118.CrossRefGoogle Scholar
Dijkstra, H.A., et al. 2014 Numerical bifurcation methods and their application to fluid dynamics: analysis beyond simulation. Commun. Comput. Phys. 15 (1), 1–45.CrossRefGoogle Scholar
Doering, C.R., Eckhardt, B. & Schumacher, J. 2006 Failure of energy stability in Oldroyd-B fluids at arbitrarily low Reynolds numbers. J. Non-Newtonian Fluid Mech. 135, 92–96.CrossRefGoogle Scholar
Draad, A.A., Kuiken, G.D.C. & Nieuwstadt, F.T.M. 1998 Laminar-turbulent transition in pipe flow for Newtonian and non-Newtonian fluids. J. Fluid Mech. 377, 267–312.CrossRefGoogle Scholar
Dubief, Y., Page, J., Kerswell, R.R., Terrapon, V.E. & Steinberg, V. 2020 A first coherent structure in elasto-inertial turbulence. arXiv:2006.06770.Google Scholar
Dubief, Y., Terrapon, V.E. & Soria, J. 2013 On the mechanism of elasto-inertial turbulence. Phys. Fluids 25 (11), 110817.CrossRefGoogle ScholarPubMed
Garg, P., Chaudhary, I., Khalid, M., Shankar, V. & Subramanian, G. 2018 Viscoelastic pipe flow is linearly unstable. Phys. Rev. Lett. 121, 024502.CrossRefGoogle ScholarPubMed
Goldstein, R.J., Adrian, R.J. & Kreid, D.K. 1969 Turbulent and transition pipe flow of dilute aqueous polymer solutions. Ind. Engng Chem. Fundam. 8, 498–502.CrossRefGoogle Scholar
Graham, M.D. 2014 Drag reduction and the dynamics of turbulence in simple and complex fluids. Phys. Fluids 26, 101301.CrossRefGoogle Scholar
Groisman, A. & Steinberg, V. 2000 Elastic turbulence in a polymer solution flow. Nature 405, 53–55.CrossRefGoogle Scholar
Hameduddin, I., Gayme, D.F. & Zaki, T.A. 2019 Perturbative expansions of the conformation tensor in viscoelastic flows. J. Fluid Mech. 858, 377–406.CrossRefGoogle Scholar
Hameduddin, I., Meneveau, C., Zaki, T.A. & Gayme, D.F. 2018 Geometric decomposition of the conformation tensor in viscoelastic turbulence. J. Fluid Mech. 842, 395–427.CrossRefGoogle Scholar
Hansen, R.J. & Little, R.C. 1974 Early turbulence and drag reduction phenomena in larger pipes. Nature 252, 690.CrossRefGoogle Scholar
Jones, W. & Maddock, J.L. 1966 Onset of instabilities and reduction of drag in flow of relaxing liquids through tubes and porous beds. Nature 212, 388.CrossRefGoogle Scholar
Joo, Y.L. & Shaqfeh, E.S.G. 1991 Viscoelastic Poiseuille flow through a curved channel: a new elastic instability. Phys. Fluids A: Fluid Dyn. 3 (7), 1691–1694.CrossRefGoogle Scholar
Joo, Y.L. & Shaqfeh, E.S.G. 1992 A purely elastic instability in Dean and Taylor–Dean flow. Phys. Fluids 4, 524.CrossRefGoogle Scholar
Jovanović, M.R. & Kumar, S. 2010 Transient growth without inertia. Phys. Fluids 22, 023101.CrossRefGoogle Scholar
Jovanović, M.R. & Kumar, S. 2011 Nonmodal amplification of stochastic disturbances in strongly elastic channel flows. J. Non-Newtonian Fluid Mech. 166, 755–778.CrossRefGoogle Scholar
Khalid, M., Chaudhary, I., Garg, P., Shankar, V. & Subramanian, G. 2021 a The centre-mode instability of viscoelastic plane Poiseuille flow. J. Fluid Mech. 915, A43.CrossRefGoogle Scholar
Khalid, M., Shankar, V. & Subramanian, G. 2021 b A continuous pathway between the elasto-inertial and elastic turbulent states in viscoelastic channel flow. Phys. Rev. Lett. 127, 134502.CrossRefGoogle ScholarPubMed
Larson, R.G., Shaqfeh, E.S.G. & Muller, S.J. 1990 A purely elastic instability in Taylor–Couette flow. J. Fluid Mech. 218, 573–600.CrossRefGoogle Scholar
Lopez, J.M., Choueiri, G.H. & Hof, B. 2019 Dynamics of viscoelastic pipe flow at low Reynolds numbers in the maximum drag reduction limit. J. Fluid Mech. 874, 699–719.CrossRefGoogle Scholar
Lumley, J.L. 1969 Drag reduction by additives. Annu. Rev. Fluid Mech. 656 (33), 367–384.CrossRefGoogle Scholar
Meerbergen, K., Spence, A. & Roose, D. 1994 Shift-invert and Cayley transforms for detection of rightmost eigenvalues of nonsymmetric matrices. Bit Numer. Maths 34 (3), 409–423.CrossRefGoogle Scholar
Meulenbroek, B., Storm, C., Morozov, A.N. & van Saarloos, W. 2004 Weakly nonlinear subcritical instability of visco-elastic Poiseuille flow. J. Non-Newtonian Fluid Mech. 116 (2–3), 235–268.CrossRefGoogle Scholar
Morozov, A.N. & Saarloos, W.V. 2007 An introductory essay on subcritical instabilities and the transition to turbulence in visco-elastic parallel shear flows. Phys. Rep. 447, 112–143.CrossRefGoogle Scholar
Page, J., Dubief, Y. & Kerswell, R.R. 2020 Exact traveling wave solutions in viscoelastic channel flow. Phys. Rev. Lett. 125, 154501.CrossRefGoogle ScholarPubMed
Page, J. & Zaki, T.A. 2015 The dynamics of spanwise vorticity perturbations in homogeneous viscoelastic shear flow. J. Fluid Mech. 777, 327–363.CrossRefGoogle Scholar
Pan, L., Morozov, A., Wagner, C. & Arratia, P.E. 2013 Nonlinear elastic instability in channel flows at low Reynolds numbers. Phys. Rev. Lett. 110, 174502.CrossRefGoogle ScholarPubMed
Procaccia, I., Lvov, V. & Benzi, R. 2008 Colloquium: theory of drag reduction by polymers in wall-bounded turbulence. Rev. Mod. Phys. 1, 225–247.CrossRefGoogle Scholar
Qin, B.Y., Salipante, P.F., Hudson, S.D. & Arratia, P.E. 2019 Flow resistance and structure in viscoelastic channel flows at low Re. Phys. Rev. Lett. 123, 194501.CrossRefGoogle Scholar
Ray, P.K. & Zaki, T.A. 2014 Absolute instability in viscoelastic mixing layers. Phys. Fluids 26 (1), 014103.CrossRefGoogle Scholar
Samanta, D.S., Dubief, Y., Holzner, H., Schäfer, C., Morozov, A.N., Wagner, C. & Hof, B. 2013 Elasto-inertial turbulence. Proc. Natl Acad. Sci. USA 110, 10557–10562.CrossRefGoogle ScholarPubMed
Sanchez, H.A.C., Jovanovic, M.R., Kumar, S., Morozov, A., Shankar, V., Subramanian, G. & Wilson, H.J. 2022 Understanding viscoelastic flow instabilities: Oldroyd-B and beyond. J. Non-Newtonian Fluid Mech. 302, 104742.CrossRefGoogle Scholar
Schnapp, R. & Steinberg, V. 2021 Elastic waves above elastically driven instability in weakly perturbed channel flow. arXiv:2106.01817.Google Scholar
Shaqfeh, E.S.G. 1996 Purely elastic instabilities in viscometric flows. Annu. Rev. Fluid Mech. 28, 129–185.CrossRefGoogle Scholar
Shekar, A., MucMullen, R.M., McKeon, B.J. & Graham, M.D. 2020 Self-sustained elastoinertial Tolmien–Schlichting waves. J. Fluid Mech. 897, A3.CrossRefGoogle Scholar
Shekar, A., MucMullen, R.M., Wang, S.N., McKeon, B.J. & Graham, M.D. 2018 Critical-layer structures and mechanisms in elastoinertial turbulence. Phys. Rev. Lett. 122, 124503.CrossRefGoogle Scholar
Sid, S., Terrapon, V.E. & Dubief, Y. 2018 Two-dimensional dynamics of elasto-inertial turbulence and its role in polymer drag reduction. Phys. Rev. Fluids 3, 01130(R).CrossRefGoogle Scholar
Steinberg, V. 2021 Elastic turbulence: an experimental view on inertialess random flow. Annu. Rev. Fluid Mech. 53, 27.CrossRefGoogle Scholar
Stuart, J.T. 1960 On the non-linear mechanics of wave disturbances in stable and unstable parallel flows. Part 1. The basic behaviour in plane Poiseuille flow. J. Fluid Mech. 9, 353–370.CrossRefGoogle Scholar
Tabor, M. & de Gennes, P.G. 1986 A cascade theory of drag reduction. Europhys. Lett. 2 (7), 519–522.CrossRefGoogle Scholar
Terrapon, V., Dubief, Y. & Soria, J. 2015 On the role of pressure in elasto-inertial turbulence. J. Turbul. 16, 26–43.CrossRefGoogle Scholar
Toms, B.A. 1948 Observation on the flow of linear polymer solutions through straight tubes at large Reynolds numbers. First Intern. Congr. Rheology 11, 135–141.Google Scholar
Vashney, A. & Steinberg, V. 2018 Drag enhancement and drag reduction in viscoelastic flow. Phys. Rev. Fluids 3, 103302.CrossRefGoogle Scholar
Virk, P.S. 1970 Drag reduction fundamentals. AIChE J. 21, 625–656.CrossRefGoogle Scholar
Wan, D., Sun, G. & Zhang, M. 2021 Subcritical and supercritical bifurcations in axisymmetric viscoelastic pipe flows. J. Fluid Mech. 929, A16.CrossRefGoogle Scholar
Watson, J. 1960 On the non-linear mechanics of wave disturbances in stable and unstable parallel flows. Part 2. The development of a solution for plane Poiseuille flow and for plane Couette flow. J. Fluid Mech. 9, 372–389.CrossRefGoogle Scholar
White, C.M. & Mungal, M.G. 2008 Mechanics and prediction of turbulent drag reduction with polymer additives. Annu. Rev. Fluid Mech. 40, 235–256.CrossRefGoogle Scholar
Xi, L. & Graham, M.D. 2010 Turbulent drag reduction and multistage transitions in viscoelastic minimal flow units. J. Fluid Mech. 647, 421–452.CrossRefGoogle Scholar
Xi, L. & Graham, M.D. 2012 Intermittent dynamics of turbulence hibernation in Newtonian and viscoelastic minimal channel flows. J. Fluid Mech. 693, 433–472.CrossRefGoogle Scholar
Zhang, M. 2021 Energy growth in subcritical viscoelastic pipe flows. J. Non-Newtonian Fluid Mech. 294, 104581.CrossRefGoogle Scholar
Zhang, M., Lashgari, I., Zaki, T.A. & Brandt, L. 2013 Linear stability analysis of channel flow of viscoelastic Oldroyd-B and FENE-P fluids. J. Fluid Mech. 737, 249–279.CrossRefGoogle Scholar