Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-25T05:23:01.897Z Has data issue: false hasContentIssue false
  • English
  • Français

Viscous flow in a soft valve

Published online by Cambridge University Press:  11 December 2017

K. Park ,
A. Tixier ,
A. H. Christensen ,
S. F. Arnbjerg-Nielsen ,
M. A. Zwieniecki  and
K. H. Jensen Open the ORCID record for K. H. Jensen [Opens in a new window]
K. Park
Affiliation:
Department of Physics, Technical University of Denmark, DK-2800 Kgs. Lyngby, Denmark
A. Tixier
Affiliation:
Department of Plant Sciences, University of California, Davis, CA 95616, USA
A. H. Christensen
Affiliation:
Department of Physics, Technical University of Denmark, DK-2800 Kgs. Lyngby, Denmark
S. F. Arnbjerg-Nielsen
Affiliation:
Department of Physics, Technical University of Denmark, DK-2800 Kgs. Lyngby, Denmark
M. A. Zwieniecki*
Affiliation:
Department of Plant Sciences, University of California, Davis, CA 95616, USA
K. H. Jensen*
Affiliation:
Department of Physics, Technical University of Denmark, DK-2800 Kgs. Lyngby, Denmark
*
†Email addresses for correspondence: [email protected], [email protected]
†Email addresses for correspondence: [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Fluid–structure interactions are ubiquitous in nature and technology. However, the systems are often so complex that numerical simulations or ad hoc assumptions must be used to gain insight into the details of the complex interactions between the fluid and solid mechanics. In this paper, we present experiments and theory on viscous flow in a simple bioinspired soft valve which illustrate essential features of interactions between hydrodynamic and elastic forces at low Reynolds numbers. The set-up comprises a sphere connected to a spring located inside a tapering cylindrical channel. The spring is aligned with the central axis of the channel and a pressure drop is applied across the sphere, thus forcing the liquid through the narrow gap between the sphere and the channel walls. The sphere’s equilibrium position is determined by a balance between spring and hydrodynamic forces. Since the gap thickness changes with the sphere’s position, the system has a pressure-dependent hydraulic resistance. This leads to a nonlinear relation between applied pressure and flow rate: flow initially increases with pressure, but decreases when the pressure exceeds a certain critical value as the gap closes. To rationalize these observations, we propose a mathematical model that reduced the complexity of the flow to a two-dimensional lubrication approximation. 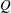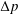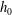A closed-form expression for the pressure drop/flow rate is obtained which reveals that the flow rate $Q$ depends on the pressure drop $\unicode[STIX]{x0394}p$, sphere radius $a$, gap thickness $h_{0}$, and viscosity 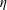$\unicode[STIX]{x1D702}$ as $Q\sim \unicode[STIX]{x1D702}^{-1}a^{1/2}h_{0}^{5/2}(1-\unicode[STIX]{x0394}p/\unicode[STIX]{x0394}p_{c})^{5/2}\unicode[STIX]{x0394}p$, where the critical pressure 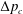$\unicode[STIX]{x0394}p_{c}$ scales with the spring constant 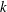$k$ as $\unicode[STIX]{x0394}p_{c}\sim kh_{0}a^{-2}$. These predictions compared favourably to the results of our experiments with no free parameters.

JFM classification

Aerodynamics: Flow-structure interactions Low-Reynolds-number flows: Lubrication theory Micro-/Nano-fluid dynamics: Microfluidics
Type
JFM Rapids
Information
Journal of Fluid Mechanics , Volume 836 , 10 February 2018 , R3
Check for updates
Copyright
© 2017 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Bearman, P. W. 1984 Vortex shedding from oscillating bluff bodies. Annu. Rev. Fluid Mech. 16 (1), 195–222.Google Scholar
Bellhouse, B. J. & Talbot, L. 1969 The fluid mechanics of the aortic valve. J. Fluid Mech. 35 (4), 721–735.Google Scholar
Capron, M., Tordjeman, P., Charru, F., Badel, E. & Cochard, H. 2014 Gas flow in plant microfluidic networks controlled by capillary valves. Phys. Rev. E 89 (3), 033019.Google Scholar
Chapman, D. C., Rand, R. H. & Cooks, J. R. 1977 A hydrodynamical model of bordered pits in conifer tracheids. J. Theor. Biol. 67 (1), 11–24.Google Scholar
Choat, B., Cobb, A. R. & Jansen, S. 2008 Structure and function of bordered pits: new discoveries and impacts on whole-plant hydraulic function. New Phytol. 177 (3), 608–626.Google Scholar
Duprat, C. & Stone, H. A. 2015 Fluid-Structure Interactions in Low-Reynolds-Number Flows. Royal Society of Chemistry.CrossRefGoogle Scholar
Gart, S., Socha, J. J., Vlachos, P. P. & Jung, S. 2015 Dogs lap using acceleration-driven open pumping. Proc. Natl Acad. Sci. USA 112 (52), 15798–15802.Google Scholar
Grotberg, J. B. & Jensen, O. E. 2004 Biofluid mechanics in flexible tubes. Annu. Rev. Fluid Mech. 36, 121–147.Google Scholar
Heil, M. & Hazel, A. L. 2011 Fluid-structure interaction in internal physiological flows. Annu. Rev. Fluid Mech. 43, 141–162.Google Scholar
Heil, M. & Hazel, A. L. 2015 Flow in flexible/collapsible tubes. In Fluid-Structure Interactions in Low-Reynolds-Number Flows (ed. Duprat, C. & Stone, H.), pp. 280–312. Royal Society of Chemistry.Google Scholar
Holmes, D. P., Tavakol, B., Froehlicher, G. & Stone, H. A. 2013 Control and manipulation of microfluidic flow via elastic deformations. Soft Matt. 9 (29), 7049–7053.Google Scholar
Jensen, K. H., Berg-Sørensen, K., Bruus, H., Holbrook, N. M., Liesche, J., Schulz, A., Zwieniecki, M. A. & Bohr, T. 2016 Sap flow and sugar transport in plants. Rev. Mod. Phys. 88 (3), 035007.Google Scholar
Kim, W. & Bush, J. W. M. 2012 Natural drinking strategies. J. Fluid Mech. 705, 7–25.Google Scholar
Lancashire, J. R. & Ennos, A. R. 2002 Modelling the hydrodynamic resistance of bordered pits. J. Expl Bot. 53 (373), 1485–1493.CrossRefGoogle ScholarPubMed
Ledesma-Alonso, R., Guzmán, J. E. V. & Zenit, R. 2014 Experimental study of a model valve with flexible leaflets in a pulsatile flow. J. Fluid Mech. 739, 338–362.Google Scholar
Luo, X. Y. & Pedley, T. J. 1996 A numerical simulation of unsteady flow in a two-dimensional collapsible channel. J. Fluid Mech. 314, 191–225.Google Scholar
Luo, X. Y. & Pedley, T. J. 2000 Multiple solutions and flow limitation in collapsible channel flows. J. Fluid Mech. 420, 301–324.CrossRefGoogle Scholar
McCulloh, K. A., Sperry, J. S. & Adler, F. R. 2003 Water transport in plants obeys Murray’s law. Nature 421 (6926), 939–942.Google Scholar
Park, K., Kim, W. & Kim, H.-Y. 2014 Optimal lamellar arrangement in fish gills. Proc. Natl Acad. Sci. USA 111 (22), 8067–8070.Google Scholar
Patil, P. P. & Tiwari, S. 2008 Effect of blockage ratio on wake transition for flow past square cylinder. Fluid Dyn. Res. 40 (11), 753–778.Google Scholar
Sahin, M. & Owens, R. G. 2004 A numerical investigation of wall effects up to high blockage ratios on two-dimensional flow past a confined circular cylinder. Phys. Fluids 16 (5), 1305–1320.Google Scholar
Schulte, P. J. 2012 Computational fluid dynamics models of conifer bordered pits show how pit structure affects flow. New Phytol. 193 (3), 721–729.Google Scholar
Smistrup, K. & Stone, H. A. 2007 A magnetically actuated ball valve applicable for small-scale fluid flows. Phys. Fluids 19 (6), 063101.Google Scholar
Sotiropoulos, F., Le, T. B. & Gilmanov, A. 2016 Fluid mechanics of heart valves and their replacements. Annu. Rev. Fluid Mech. 48, 259–283.Google Scholar
Wexler, J. S., Trinh, P. H., Berthet, H., Quennouz, N., du Roure, O., Huppert, H. E., Lindner, A. & Stone, H. A. 2013 Bending of elastic fibres in viscous flows: the influence of confinement. J. Fluid Mech. 720, 517–544.Google Scholar
Williamson, C. H. 1996 Vortex dynamics in the cylinder wake. Annu. Rev. Fluid Mech. 28 (1), 477–539.Google Scholar
Williamson, C. H. K. & Govardhan, R. 2004 Vortex-induced vibrations. Annu. Rev. Fluid Mech. 36, 413–455.CrossRefGoogle Scholar
Yang, P. J., Pham, J., Choo, J. & Hu, D. L. 2014 Duration of urination does not change with body size. Proc. Natl Acad. Sci. USA 111 (33), 11932–11937.Google Scholar

Park et al. supplementary movie 2

Experimental movie of a soft valve with water and $z_d$ = 3.6 mm. The observed oscillation frequency is $f_{ ext{obs}}$ ~ 2.7 Hz, which is significantly slower than the systems's spring-mass oscillation frequency $f_{ ext{spring}}=1/(2\pi)(k/m)^{1/2}=32$ Hz.

Download Park et al. supplementary movie 2(Video)
Video 4.8 MB

Park et al. supplementary movie 1

Experimental movie of a soft valve with water and $z_d$ = 2.9 mm

Download Park et al. supplementary movie 1(Video)
Video 1.7 MB