Hostname: page-component-78c5997874-dh8gc Total loading time: 0 Render date: 2024-11-17T14:17:55.187Z Has data issue: false hasContentIssue false
  • English
  • Français

Turbulence decay in a supersonic boundary layer subjected to a transverse sonic jet

Published online by Cambridge University Press:  21 March 2019

Mingbo Sun Open the ORCID record for Mingbo Sun [Opens in a new window] ,
Yuan Liu  and
Zhiwei Hu
Mingbo Sun*
Affiliation:
Science and Technology on Scramjet Laboratory, National University of Defense Technology, Changsha, 410073, China
Yuan Liu
Affiliation:
Science and Technology on Scramjet Laboratory, National University of Defense Technology, Changsha, 410073, China
Zhiwei Hu
Affiliation:
Aerodynamics and Flight Mechanics, Faculty of Engineering and the Environment, University of Southampton, SouthamptonSO17 1BJ, UK
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

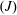The turbulence state in a supersonic boundary layer subjected to a transverse sonic jet is studied by conducting direct numerical simulations. Turbulence statistics for two jet-to-cross-flow momentum flux ratios $(J)$ of 2.3 and 5.5 based on the previous simulation (Sun & Hu, J. Fluid Mech., vol. 850, 2018, pp. 551–583) are given and compared with a flat-plate boundary layer without a jet 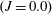$(J=0.0)$. The instantaneous and time-averaged flow features around the transverse jet in the supersonic boundary layer are analysed. It is found that, in the near-wall region, turbulence is suppressed significantly with increasing 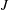$J$ in the lateral boundary layer around the jet and the turbulence decay is retained in the downstream recovery region. The local boundary-layer thickness decreases noticeably in the lateral downstream of the jet. Analysis of the cross-flow streamlines reveals a double-expansion character in the vicinity of the jet, which involves the reattachment expansion related to the flow over the jet windward separation bubble and the jet lateral expansion related to the flow around the jet barrel shock. The double expansion leads to the turbulence decay in the jet lateral boundary layer and causes a slow recovery of the outer layer in the far-field boundary layer. A preliminary experiment based on the nanoparticle laser scattering technique is conducted and confirms the existence of the turbulence decay phenomenon.

JFM classification

Compressible Flows: Compressible boundary layers Aerodynamics: High-speed flow Wakes/Jets: Jets
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 867 , 25 May 2019 , pp. 216 - 249
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Andreopoulos, Y., Agui, J. H. & Briassulis, G. 2000 Shock wave–turbulence interactions. Annu. Rev. Fluid Mech. 32 (1), 309–345.Google Scholar
Arnette, S. A., Samimy, M. O. & Elliott, G. S. 1998 The effects of expansion on the turbulence structure of compressible boundary layers. J. Fluid Mech. 367, 67–105.Google Scholar
Ben-Yakar, A., Mungal, G. M. & Hanson, R. K. 2006 Time evolution and mixing characteristics of hydrogen and ethylene transverse jets in supersonic crossflows. Phys. Fluids 18, 26–101.Google Scholar
Chai, X., Iyer, P. S. & Mahesh, K. 2015 Numerical study of high speed jets in crossflow. J. Fluid Mech. 785, 152–188.Google Scholar
Dickmann, D. A. & Lu, F. K. 2009 Shock/boundary-layer interaction effects on transverse jets in crossflow over a flat plate. J. Spacecr. Rockets 46, 1132–1141.Google Scholar
van Driest, E. R. 1956 The problem of aerodynamic heating. Aeronaut. Engng Rev. 15, 26–41.Google Scholar
Dussauge, J. P. & Gaviglio, J. 1987 The rapid expansion of a supersonic turbulent flow: role of bulk dilatation. J. Fluid Mech. 174, 81–112.Google Scholar
Everett, D. E., Woodmansee, M. A., Dutton, J. C. & Morris, M. J. 1998 Wall pressure measurements for a sonic jet injected transversely into a supersonic crossflow. J. Propul. Power 14, 861–886.Google Scholar
Gamba, M. & Mungal, M. G. 2015 Ignition, flame structure and near-wall burning in transverse hydrogen jets in supersonic crossflow. J. Fluid Mech. 780, 226–273.Google Scholar
Gao, T. Y., Liang, J. H., Sun, M. B. & Zhao, Y. H. 2017 Analysis of separation modes variation in a scramjet combustor with single-side expansion. AIAA J. 55, 1307–1317.Google Scholar
Gruber, M. R., Nejad, A. S., Chen, T. H. & Dutton, J. C. 1997 Large structure convection velocity measurements in compressible transverse injection flowfields. Exp. Fluids 22, 397–407.Google Scholar
Karagozian, A. R. 2010 Transverse jets and their control. Prog. Energy Combust. Sci. 36, 531–553.Google Scholar
Kawai, S. & Lele, S. K. 2010 Large-eddy simulation of jet mixing in supersonic crossflows. AIAA J. 48, 2063–2083.Google Scholar
Liang, C. H., Sun, M. B., Liu, Y. & Yang, Y. X. 2018 Shock wave structures in the wake of sonic transverse jet into a supersonic crossflow. Acta Astron. 148, 12–21.Google Scholar
Liu, Y., Sun, M. B., Liang, C. H., Cai, Z. & Wang, Y. N. 2018 Structures of near-wall wakes subjected to a sonic jet in a supersonic crossflow. Acta Astron. 151, 886–892.Google Scholar
Lumley, J. L. 1979 Computational modeling of turbulent flows. In Advances in Applied Mechanics (ed. Yih, C.-S.), vol. 18, pp. 123–176. Academic.Google Scholar
Mahesh, K. 2013 The interaction of jets with crossflow. Annu. Rev. Fluid Mech. 45, 379–407.Google Scholar
Martin, M. P. 2007 Direct numerical simulation of hypersonic turbulent boundary layers. Part 1. Initialization and comparison with experiments. J. Fluid Mech. 570, 347–364.Google Scholar
Portz, R. & Segal, C. 2006 Penetration of gaseous jets in supersonic flows. AIAA J. 44, 2426–2429.Google Scholar
Rana, Z. A., Thomber, B. & Drikakis, D. 2011 Transverse jet injection into a supersonic turbulent cross-flow. Phys. Fluids 23, 1–22.Google Scholar
Sandham, N. D. 2016 Effects of compressibility and shock-wave interactions on turbulent shear flows. Flow Turbul. Combust. 97, 1–25.Google Scholar
Sandham, N. D., Johnstone, R. & Jacobs, C. T. 2017 Surface-sampled simulations of turbulent flow at high Reynolds number. Int. J. Numer. Meth. Fluids 85, 1–13.Google Scholar
Sandham, N. D., Li, Q. & Yee, H. C. 2002 Entropy splitting for high-order numerical simulation of compressible turbulence. J. Comput. Phys. 178, 307–322.Google Scholar
Sandham, N. D., Schlein, E., Wagner, A., Willems, S. & Steelant, J. 2014 Transitional shock-wave/boundary-layer interactions in hypersonic flow. J. Fluid Mech. 752, 349–382.Google Scholar
Santiago, J. G. & Dutton, J. C. 1997 Velocity measurements of a jet injected into a supersonic crossflow. J. Propul. Power 13, 264–273.Google Scholar
Schetz, J. & Billig, F. S. 1966 Penetration of gaseous jets injected into a supersonic stream. J. Spacecr. Rockets 3, 1658–1665.Google Scholar
Schlatter, P. & Orlu, R. 2010 Assessment of direct numerical simulation data of turbulent boundary layers. J. Fluid Mech. 659, 116–126.Google Scholar
Sun, M. B. & Hu, Z. W. 2018a Formation of surface trailing counter-rotating vortex pairs downstream of a sonic jet in a supersonic cross-flow. J. Fluid Mech. 850, 551–583.Google Scholar
Sun, M. B. & Hu, Z. W. 2018b Generation of upper trailing counter-rotating vortices of a sonic jet in a supersonic crossflow. AIAA J. 56, 1047–1059.Google Scholar
Sun, M. B. & Hu, Z. W. 2018c Mixing in nearwall regions downstream of a sonic jet in a supersonic crossflow at Mach 2.7. Phys. Fluids 30, 106102.Google Scholar
Sun, M. B., Hu, Z. W. & Sandham, N. D. 2017 Recovery of a supersonic turbulent boundary layer after an expansion corner. Phys. Fluids 29, 076103.Google Scholar
Sun, M. B., Zhang, S. P., Zhao, Y. H., Zhao, Y. X. & Liang, J. H. 2013 Experimental investigation on transverse jet penetration into a supersonic turbulent crossflow. Sci. China Tech. Sci. 56, 1989–1998.Google Scholar
Thompson, K. W. 1987 Time dependent boundary conditions for hyperbolic systems. J. Comput. Phys. 68, 1–24.Google Scholar
Touber, E.2010 Unsteadiness in shock wave boundary layer interactions. PhD thesis, University of Southampton.Google Scholar
Touber, E. & Sandham, N. D. 2011 Low-order stochastic modelling of low-frequency motions in reflected shock-wave/boundary-layer interactions. J. Fluid Mech. 671, 417–465.Google Scholar
Wang, B., Sandham, N. D., Hu, Z. W. & Liu, W. D. 2015 Numerical study of oblique shock-wave/boundary-layer interaction considering sidewall effect. J. Fluid Mech. 767, 526–561.Google Scholar
Wang, Q. C. & Wang, Z. G. 2016 Structural characteristics of the supersonic turbulent boundary layer subjected to concave curvature. Appl. Phys. Lett. 108, 114102.Google Scholar
Won, S. H., Jeung, I. S., Parent, B. & Choi, J. Y. 2010 Numerical investigation of transverse hydrogen jet into supersonic crossflow using detached-eddy simulation. AIAA J. 48, 1047–1058.Google Scholar
Xie, Z. T. & Castro, I. P. 2008 Efficient generation of inflow conditions for large-eddy simulation of street-scale flows. Flow Turbul. Combust. 81, 449–470.Google Scholar
Young, A. D. 1989 Boundary Layers. BSP Professional.Google Scholar
Zhao, Y. X., Yi, S. H., Tian, L. F. & Cheng, Z. Y. 2009 Supersonic flow imaging via nanoparticles. Sci. China Ser. E 52, 3640–3648.Google Scholar