Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-28T10:32:45.009Z Has data issue: false hasContentIssue false
  • English
  • Français

The spatial structure of electrostatically forced Faraday waves

Published online by Cambridge University Press:  23 March 2022

S. Dehe Open the ORCID record for S. Dehe [Opens in a new window] ,
M. Hartmann Open the ORCID record for M. Hartmann [Opens in a new window] ,
A. Bandopadhyay Open the ORCID record for A. Bandopadhyay [Opens in a new window]  and
S. Hardt Open the ORCID record for S. Hardt [Opens in a new window]
S. Dehe
Affiliation:
Fachgebiet Nano- und Mikrofluidik, Fachbereich Maschinenbau, TU Darmstadt, 64287 Darmstadt, Germany
M. Hartmann
Affiliation:
Fachgebiet Nano- und Mikrofluidik, Fachbereich Maschinenbau, TU Darmstadt, 64287 Darmstadt, Germany
A. Bandopadhyay
Affiliation:
Department of Mechanical Engineering, Indian Institute of Technology Kharagpur, Kharagpur 721302, India
S. Hardt*
Affiliation:
Fachgebiet Nano- und Mikrofluidik, Fachbereich Maschinenbau, TU Darmstadt, 64287 Darmstadt, Germany
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

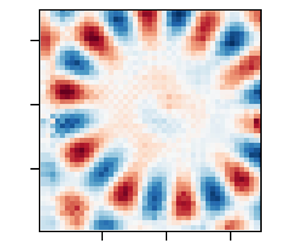
The instability of the interface between a dielectric and a conducting liquid, excited by a spatially homogeneous interface-normal time-periodic electric field, is studied based on experiments and theory. Special attention is paid to the spatial structure of the excited Faraday waves. The dominant modes of the instability are extracted using high-speed imaging in combination with an algorithm evaluating light refraction at the liquid–liquid interface. The influence of the liquid viscosities on the critical voltage corresponding to the onset of instability and on the dominant wavelength is studied. Overall, good agreement with theoretical predictions that are based on viscous fluids in an infinite domain is demonstrated. Depending on the relative influence of the domain boundary, the patterns exhibit either discrete modes corresponding to surface harmonics or boundary-independent patterns. The agreement between experiments and theory confirms that the electrostatically forced Faraday instability is sufficiently well understood, which may pave the way to control electrostatically driven instabilities. Last but not least, the analogies to classical Faraday instabilities may enable new approaches to study effects that have so far only been observed for mechanical forcing.

JFM classification

Waves/Free-surface Flows: Faraday waves Instability: Parametric instability
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 939 , 25 May 2022 , A6
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Bandopadhyay, A. & Hardt, S. 2017 Stability of horizontal viscous fluid layers in a vertical arbitrary time periodic electric field. Phys. Fluids 29 (12), 124101.CrossRefGoogle Scholar
Batson, W., Zoueshtiagh, F. & Narayanan, R. 2013 Dual role of gravity on the Faraday threshold for immiscible viscous layers. Phys. Rev. E 88 (6), 063002.CrossRefGoogle ScholarPubMed
Benjamin, T.B. & Ursell, F. 1954 The stability of the plane free surface of a liquid in vertical periodic motion. Proc. R. Soc. Lond. A 225 (1163), 505–515.Google Scholar
Besson, T., Edwards, W.S. & Tuckerman, L.S. 1996 Two-frequency parametric excitation of surface waves. Phys. Rev. E 54 (1), 507–513.CrossRefGoogle ScholarPubMed
Briskman, V.A. & Shaidurov, G.F. 1968 Parametric instability of a fluid surface in an alternating electric field. Sov. Phys. Dokl. 13 (6), 540–542.Google Scholar
Bush, J.W.M. 2015 Pilot-wave hydrodynamics. Annu. Rev. Fluid Mech. 47 (1), 269–292.CrossRefGoogle Scholar
Chen, P. & Viñals, J. 1999 Amplitude equation and pattern selection in Faraday waves. Phys. Rev. E 60 (1), 559–570.CrossRefGoogle ScholarPubMed
Chen, P. & Wu, K.-A. 2000 Subcritical bifurcations and nonlinear balloons in Faraday waves. Phys. Rev. Lett. 85 (18), 3813–3816.CrossRefGoogle ScholarPubMed
Ciliberto, S. & Gollub, J.P. 1984 Pattern competition leads to chaos. Phys. Rev. Lett. 52 (11), 922–925.CrossRefGoogle Scholar
Couder, Y., Protière, S., Fort, E. & Boudaoud, A. 2005 Walking and orbiting droplets. Nature 437 (7056), 208–208.CrossRefGoogle ScholarPubMed
Craik, A.D.D. & Armitage, J.G.M. 1995 Faraday excitation, hysteresis and wave instability in a narrow rectangular wave tank. Fluid Dyn. Res. 15 (3), 129–143.CrossRefGoogle Scholar
Cross, M.C. & Hohenberg, P.C. 1993 Pattern formation outside of equilibrium. Rev. Mod. Phys. 65 (3), 851–1112.CrossRefGoogle Scholar
Dodge, F.T., Kana, D.D. & Abramson, H.N. 1965 Liquid surface oscillations in longitudinally excited rigid cylindrical containers. AIAA J. 3 (4), 685–695.CrossRefGoogle Scholar
Douady, S. 1990 Experimental study of the Faraday instability. J. Fluid Mech. 221 (5), 383–409.CrossRefGoogle Scholar
Douady, S. & Fauve, S. 1988 Pattern selection in faraday instability. Eur. Phys. Lett. 6 (3), 221–226.CrossRefGoogle Scholar
Edwards, W.S. & Fauve, S. 1994 Patterns and quasi-patterns in the Faraday experiment. J. Fluid Mech. 278 (II), 123–148.CrossRefGoogle Scholar
Epstein, T. & Fineberg, J. 2008 Necessary conditions for mode interactions in parametrically excited waves. Phys. Rev. Lett. 100 (13), 134101.CrossRefGoogle ScholarPubMed
Faraday, M. 1831 XVII. On a peculiar class of acoustical figures; and on certain forms assumed by groups of particles upon vibrating elastic surfaces. Phil. Trans. R. Soc. Lond. 121 (August 1827), 299–340.Google Scholar
Fernández-Mateo, R. & Pérez, A.T. 2021 Faraday waves under perpendicular electric field and their application to the walking droplet phenomenon. Phys. Fluids 33 (1), 017109.CrossRefGoogle Scholar
Förstner, W. & Gülch, E. 1987 A fast operator for detection and precise location of distinct points, corners and centres of circular features. In Proc. ISPRS Intercommission Conference on Fast Processing of Photogrammetric Data, pp. 281–305. Interlaken.Google Scholar
Gambhire, P. & Thaokar, R. 2014 Electrokinetic model for electric-field-induced interfacial instabilities. Phys. Rev. E 89 (3), 032409.CrossRefGoogle ScholarPubMed
Gambhire, P. & Thaokar, R.M. 2010 Electrohydrodynamic instabilities at interfaces subjected to alternating electric field. Phys. Fluids 22 (6), 064103.CrossRefGoogle Scholar
Gambhire, P. & Thaokar, R.M. 2012 Role of conductivity in the electrohydrodynamic patterning of air-liquid interfaces. Phys. Rev. E 86 (3), 036301.CrossRefGoogle ScholarPubMed
Gluckman, B.J., Marcq, P., Bridger, J. & Gollub, J.P. 1993 Time averaging of chaotic spatiotemporal wave patterns. Phys. Rev. Lett. 71 (13), 2034–2037.CrossRefGoogle ScholarPubMed
Gollub, J.P. & Meyer, C.W. 1983 Symmetry-breaking instabilities on a fluid surface. Physica D 6 (3), 337–346.CrossRefGoogle Scholar
Iino, M., Suzuki, M. & Ikushima, A.J. 1985 Surface-wave resonance method for measuring surface tension with a very high precision. J. Phys. Colloq. 46 (C10), C10–813–C10–816.CrossRefGoogle Scholar
Jones, T.B. & Melcher, J.R. 1973 Dynamics of electromechanical flow structures. Phys. Fluids 16 (3), 393–400.CrossRefGoogle Scholar
Kahouadji, L., Périnet, N., Tuckerman, L.S., Shin, S., Chergui, J. & Juric, D. 2015 Numerical simulation of supersquare patterns in Faraday waves. J. Fluid Mech. 772, R2.CrossRefGoogle Scholar
Kityk, A.V., Embs, J., Mekhonoshin, V.V. & Wagner, C. 2005 Spatiotemporal characterization of interfacial Faraday waves by means of a light absorption technique. Phys. Rev. E 72 (3), 036209.CrossRefGoogle ScholarPubMed
Kumar, K. & Tuckerman, L.S. 1994 Parametric instability of the interface between two fluids. J. Fluid Mech. 279 (2014), 49–68.CrossRefGoogle Scholar
Matthiessen, L. 1868 Akustische versuche, die kleinsten transversalwellen der flüssigkeiten betreffend. Ann. Phys. Chem. 210 (5), 107–117.CrossRefGoogle Scholar
Matthiessen, L. 1870 Ueber die Transversalschwingungen tönender tropfbarer und elastischer Flüssigkeiten. Ann. Phys. Chem. 217 (11), 375–393.CrossRefGoogle Scholar
Melcher, J.R. 1966 Traveling-wave induced electroconvection. Phys. Fluids 9 (8), 1548–1555.CrossRefGoogle Scholar
Melcher, J.R. & Schwarz, W.J. 1968 Interfacial relaxation overstability in a tangential electric field. Phys. Fluids 11 (12), 2604–2616.CrossRefGoogle Scholar
Miles, J. 1990 Parametrically forced surface waves. Annu. Rev. Fluid Mech. 22 (1), 143–165.CrossRefGoogle Scholar
Moisy, F., Rabaud, M. & Salsac, K. 2009 A synthetic Schlieren method for the measurement of the topography of a liquid interface. Exp. Fluids 46 (6), 1021–1036.CrossRefGoogle Scholar
Müller, H.W., Friedrich, R. & Papathanassiou, D. 2011 Theoretical and experimental investigations of the Faraday instability. In Evolution of Spontaneous Structures in Dissipative Continuous Systems, vol. 44, pp. 230–265. Springer.CrossRefGoogle Scholar
Nevolin, V.G. 1984 Parametric excitation of surface waves. J. Engng Phys. 47 (6), 1482–1494.CrossRefGoogle Scholar
Perlin, M. & Schultz, W.W. 2000 Capillary effects on surface waves. Annu. Rev. Fluid Mech. 32 (1), 241–274.CrossRefGoogle Scholar
Pillai, D.S. & Narayanan, R. 2018 Nonlinear dynamics of electrostatic Faraday instability in thin films. J. Fluid Mech. 855, R4.CrossRefGoogle Scholar
Rajchenbach, J. & Clamond, D. 2015 Faraday waves: their dispersion relation, nature of bifurcation and wavenumber selection revisited. J. Fluid Mech. 777, R2.CrossRefGoogle Scholar
Rayleigh, Lord 1883 XXXIII. On maintained vibrations. Lond. Edinb. Dublin Philos. Mag. J. Science 15 (94), 229–235.CrossRefGoogle Scholar
Roberts, S.A. & Kumar, S. 2009 AC electrohydrodynamic instabilities in thin liquid films. J. Fluid Mech. 631, 255–279.CrossRefGoogle Scholar
Robinson, J.A., Bergougnou, M.A., Cairns, W.L., Castle, G.S.P. & Inculet, I.I. 2000 Breakdown of air over a water surface stressed by a perpendicular alternating electric field, in the presence of a dielectric barrier. IEEE Trans. Ind. Appl. 36 (1), 68–75.CrossRefGoogle Scholar
Robinson, J.A., Bergougnou, M.A., Castle, G.S.P. & Inculet, I.I. 2001 The electric field at a water surface stressed by an AC voltage. IEEE Trans. Ind. Applics. 37 (3), 735–742.CrossRefGoogle Scholar
Robinson, J.A., Bergougnou, M.A., Castle, G.S.P. & Inculet, I.I. 2002 A nonlinear model of AC-field-induced parametric waves on a water surface. IEEE Trans. Ind. Applics. 38 (2), 379–388.CrossRefGoogle Scholar
Serpooshan, V., et al. 2017 Bioacoustic-enabled patterning of human iPSC-derived cardiomyocytes into 3D cardiac tissue. Biomaterials 131, 47–57.CrossRefGoogle ScholarPubMed
Shao, X., Wilson, P., Saylor, J.R. & Bostwick, J.B. 2021 Surface wave pattern formation in a cylindrical container. J. Fluid Mech. 915, A19.CrossRefGoogle Scholar
Taylor, G.I. & McEwan, A.D. 1965 The stability of a horizontal fluid interface in a vertical electric field. J. Fluid Mech. 22 (1), 1–15.CrossRefGoogle Scholar
Tufillaro, N.B., Ramshankar, R. & Gollub, J.P. 1989 Order-disorder transition in capillary ripples. Phys. Rev. Lett. 62 (4), 422–425.CrossRefGoogle ScholarPubMed
Van der Walt, S., Schönberger, J.L., Nunez-Iglesias, J., Boulogne, F.çois, Warner, J.D., Yager, N., Gouillart, E. & Yu, T. 2014 scikit-image: image processing in python. PeerJ 2, e453.CrossRefGoogle ScholarPubMed
Ward, K., Matsumoto, S. & Narayanan, R. 2019 The electrostatically forced Faraday instability: theory and experiments. J. Fluid Mech. 862, 696–731.CrossRefGoogle Scholar
Yih, C.-S. 1968 Stability of a horizontal fluid interface in a periodic vertical electric field. Phys. Fluids 11 (7), 1447–1449.CrossRefGoogle Scholar
Zhao, S., Dietzel, M. & Hardt, S. 2019 Faraday instability of a liquid layer on a lubrication film. J. Fluid Mech. 879, 422–447.CrossRefGoogle Scholar