Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Gerken, Tobias
Chamecki, Marcelo
and
Fuentes, Jose D.
2017.
Air-Parcel Residence Times Within Forest Canopies.
Boundary-Layer Meteorology,
Vol. 165,
Issue. 1,
p.
29.
Chamecki, Marcelo
Dias, Nelson L.
Salesky, Scott T.
and
Pan, Ying
2017.
Scaling Laws for the Longitudinal Structure Function in the Atmospheric Surface Layer.
Journal of the Atmospheric Sciences,
Vol. 74,
Issue. 4,
p.
1127.
Liu, Hao
Yuan, Renmin
Mei, Jie
Sun, Jianning
Liu, Qi
and
Wang, Yu
2017.
Scale Properties of Anisotropic and Isotropic Turbulence in the Urban Surface Layer.
Boundary-Layer Meteorology,
Vol. 165,
Issue. 2,
p.
277.
Chen, Y.‐S.
Verlinde, J.
Clothiaux, E. E.
Ackerman, A. S.
Fridlind, A. M.
Chamecki, M.
Kollias, P.
Kirkpatrick, M. P.
Chen, B.‐C.
Yu, G.
and
Avramov, A.
2018.
On the Forward Modeling of Radar Doppler Spectrum Width From LES: Implications for Model Evaluation.
Journal of Geophysical Research: Atmospheres,
Vol. 123,
Issue. 14,
p.
7444.
Anderson, William
Yang, Jianzhi
Shrestha, Kalyan
and
Awasthi, Ankit
2018.
Turbulent secondary flows in wall turbulence: vortex forcing, scaling arguments, and similarity solution.
Environmental Fluid Mechanics,
Vol. 18,
Issue. 6,
p.
1351.
Brugger, Peter
Katul, Gabriel G.
De Roo, Frederik
Kröniger, Konstantin
Rotenberg, Eyal
Rohatyn, Shani
and
Mauder, Matthias
2018.
Scalewise invariant analysis of the anisotropic Reynolds stress tensor for atmospheric surface layer and canopy sublayer turbulent flows.
Physical Review Fluids,
Vol. 3,
Issue. 5,
Ghannam, Khaled
Katul, Gabriel G.
Bou-Zeid, Elie
Gerken, Tobias
and
Chamecki, Marcelo
2018.
Scaling and Similarity of the Anisotropic Coherent Eddies in Near-Surface Atmospheric Turbulence.
Journal of the Atmospheric Sciences,
Vol. 75,
Issue. 3,
p.
943.
Chamecki, Marcelo
Dias, Nelson L.
and
Freire, Livia S.
2018.
A TKE‐Based Framework for Studying Disturbed Atmospheric Surface Layer Flows and Application to Vertical Velocity Variance Over Canopies.
Geophysical Research Letters,
Vol. 45,
Issue. 13,
p.
6734.
Wang, C.
and
Anderson, W.
2019.
Turbulence Coherence Within Canonical and Realistic Aeolian Dune-Field Roughness Sublayers.
Boundary-Layer Meteorology,
Vol. 173,
Issue. 3,
p.
409.
Freire, Livia S.
Dias, Nelson L.
and
Chamecki, Marcelo
2019.
Effects of Path Averaging in a Sonic Anemometer on the Estimation of Turbulence-Kinetic-Energy Dissipation Rates.
Boundary-Layer Meteorology,
Vol. 173,
Issue. 1,
p.
99.
Freire, Livia S.
Chamecki, Marcelo
Bou‐Zeid, Elie
and
Dias, Nelson L.
2019.
Critical flux Richardson number for Kolmogorov turbulence enabled by TKE transport.
Quarterly Journal of the Royal Meteorological Society,
Vol. 145,
Issue. 721,
p.
1551.
Chamecki, Marcelo
Freire, Livia S.
Dias, Nelson L.
Chen, Bicheng
Dias-Junior, Cléo Quaresma
Toledo Machado, Luiz Augusto
Sörgel, Matthias
Tsokankunku, Anywhere
and
Araújo, Alessandro C. de
2020.
Effects of Vegetation and Topography on the Boundary Layer Structure above the Amazon Forest.
Journal of the Atmospheric Sciences,
Vol. 77,
Issue. 8,
p.
2941.
Berg, Jacob
Patton, Edward G.
and
Sullivan, Peter P.
2020.
Large-Eddy Simulation of Conditionally Neutral Boundary Layers: A Mesh Resolution Sensitivity Study.
Journal of the Atmospheric Sciences,
Vol. 77,
Issue. 6,
p.
1969.
Seleznov, Ivan
Popov, Anton
Kikuchi, Kazuhei
Kolosova, Elena
Kolomiiets, Bohdan
Nakata, Akio
Kaneko, Miki
and
Kiyono, Ken
2020.
Detection of oriented fractal scaling components in anisotropic two-dimensional trajectories.
Scientific Reports,
Vol. 10,
Issue. 1,
Joshi, P.
and
Anderson, W.
2022.
Surface layer response to heterogeneous tree canopy distributions: roughness regime regulates secondary flow polarity.
Journal of Fluid Mechanics,
Vol. 946,
Issue. ,
Dupont, Sylvain
and
Patton, Edward G.
2022.
On the Influence of Large-Scale Atmospheric Motions on Near-Surface Turbulence: Comparison Between Flows Over Low-Roughness and Tall Vegetation Canopies.
Boundary-Layer Meteorology,
Vol. 184,
Issue. 2,
p.
195.
Mortarini, Luca
Katul, Gabriel G.
Cava, Daniela
Dias-Junior, Cleo Quaresma
Dias, Nelson Luis
Manzi, Antonio
Sorgel, Matthias
Araújo, Alessandro
and
Chamecki, Marcelo
2023.
Adjustments to the law of the wall above an Amazon forest explained by a spectral link.
Physics of Fluids,
Vol. 35,
Issue. 2,
Chen, Bicheng
and
Chamecki, Marcelo
2023.
Turbulent Kinetic Energy Budgets over Gentle Topography Covered by Forests.
Journal of the Atmospheric Sciences,
Vol. 80,
Issue. 1,
p.
91.
Freire, Livia S.
Chamecki, Marcelo
and
Patton, Edward G.
2023.
Atmospheric Small-Scale Turbulence from Three-Dimensional Hot-film Data.
Boundary-Layer Meteorology,
Vol. 189,
Issue. 1-3,
p.
77.
Maryada, K.R.
Armfield, S.W.
MacDonald, M.
Dhopade, P.
and
Norris, S.E.
2024.
Universal relative scaling of longitudinal structure functions in shear-dominated turbulence.
Journal of Fluid Mechanics,
Vol. 984,
Issue. ,
 $\ell _{{\it\epsilon}}=u_{\star }^{3}/{\it\epsilon}$) is the appropriate scale for the shear-production range of the second-order streamwise structure function in neutrally stratified turbulent shear flows near solid boundaries, including smooth- and rough-wall boundary layers and shear layers above canopies (e.g. crops, forests and cities). These flows have two major characteristics in common: (i) a single velocity scale, i.e. the friction velocity (
$\ell _{{\it\epsilon}}=u_{\star }^{3}/{\it\epsilon}$) is the appropriate scale for the shear-production range of the second-order streamwise structure function in neutrally stratified turbulent shear flows near solid boundaries, including smooth- and rough-wall boundary layers and shear layers above canopies (e.g. crops, forests and cities). These flows have two major characteristics in common: (i) a single velocity scale, i.e. the friction velocity (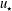 $u_{\star }$) and (ii) the presence of large eddies that scale with an external length scale much larger than the local integral length scale. No assumptions are made about the local integral scale, which is shown to be proportional to
$u_{\star }$) and (ii) the presence of large eddies that scale with an external length scale much larger than the local integral length scale. No assumptions are made about the local integral scale, which is shown to be proportional to 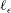 $\ell _{{\it\epsilon}}$ for the scaling analysis to be consistent with Kolmogorov’s result for the inertial subrange. Here
$\ell _{{\it\epsilon}}$ for the scaling analysis to be consistent with Kolmogorov’s result for the inertial subrange. Here  ${\it\epsilon}$ is the rate of dissipation of turbulent kinetic energy (TKE) that represents the rate of energy cascade in the inertial subrange. The scaling yields a log-law dependence of the second-order streamwise structure function on (
${\it\epsilon}$ is the rate of dissipation of turbulent kinetic energy (TKE) that represents the rate of energy cascade in the inertial subrange. The scaling yields a log-law dependence of the second-order streamwise structure function on (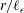 $r/\ell _{{\it\epsilon}}$), where
$r/\ell _{{\it\epsilon}}$), where  $r$ is the streamwise spatial separation. This scaling law is confirmed by large-eddy simulation (LES) results in the roughness sublayer above a model canopy, where the imbalance between local production and dissipation of TKE is much greater than in the inertial layer of wall turbulence and the local integral scale is affected by two external length scales. Parameters estimated for the log-law dependence on (
$r$ is the streamwise spatial separation. This scaling law is confirmed by large-eddy simulation (LES) results in the roughness sublayer above a model canopy, where the imbalance between local production and dissipation of TKE is much greater than in the inertial layer of wall turbulence and the local integral scale is affected by two external length scales. Parameters estimated for the log-law dependence on (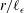 $r/\ell _{{\it\epsilon}}$) are in reasonable agreement with those reported for the inertial layer of wall turbulence. This leads to two important conclusions. Firstly, the validity of the
$r/\ell _{{\it\epsilon}}$) are in reasonable agreement with those reported for the inertial layer of wall turbulence. This leads to two important conclusions. Firstly, the validity of the  $\ell _{{\it\epsilon}}$-scaling is extended to shear flows with a much greater imbalance between production and dissipation, indicating possible universality of the shear-production range in flows near solid boundaries. Secondly, from a modelling perspective,
$\ell _{{\it\epsilon}}$-scaling is extended to shear flows with a much greater imbalance between production and dissipation, indicating possible universality of the shear-production range in flows near solid boundaries. Secondly, from a modelling perspective,  $\ell _{{\it\epsilon}}$ is the appropriate scale to characterize turbulence in shear flows with multiple externally imposed length scales.
$\ell _{{\it\epsilon}}$ is the appropriate scale to characterize turbulence in shear flows with multiple externally imposed length scales.