Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-17T14:16:41.396Z Has data issue: false hasContentIssue false
  • English
  • Français

Rotating planar gravity currents at moderate Rossby numbers: fully resolved simulations and shallow-water modelling

Published online by Cambridge University Press:  20 March 2019

Jorge S. Salinas ,
Thomas Bonometti Open the ORCID record for Thomas Bonometti [Opens in a new window] ,
Marius Ungarish Open the ORCID record for Marius Ungarish [Opens in a new window]  and
Mariano I. Cantero
Jorge S. Salinas*
Affiliation:
Comisión Nacional de Energía Atómica e Instituto Balseiro, Centro Atómico Bariloche, 8400 San Carlos de Bariloche, Argentina
Thomas Bonometti
Affiliation:
Institut de Mécanique des Fluides de Toulouse (IMFT), Université de Toulouse, CNRS-INPT-UPS, 31400 Toulouse, France
Marius Ungarish
Affiliation:
Department of Computer Science, Technion, Haifa 32000, Israel
Mariano I. Cantero
Affiliation:
Comisión Nacional de Energía Atómica e Instituto Balseiro, Centro Atómico Bariloche, 8400 San Carlos de Bariloche, Argentina Consejo Nacional de Investigaciones Científicas y Técnicas, Centro Atómico Bariloche, 8400 San Carlos de Bariloche, Argentina
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The flow of a gravity current of finite volume and density 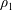$\unicode[STIX]{x1D70C}_{1}$ released from rest from a rectangular lock (of height 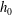$h_{0}$) into an ambient fluid of density 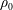$\unicode[STIX]{x1D70C}_{0}$ (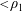${<}\unicode[STIX]{x1D70C}_{1}$) in a system rotating with 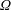$\unicode[STIX]{x1D6FA}$ about the vertical $z$ is investigated by means of fully resolved direct numerical simulations (DNS) and a theoretical model (based on shallow-water and Ekman layer spin-up theories, including mixing). The motion of the dense fluid includes several stages: propagation in the $x$-direction accompanied by Coriolis acceleration/deflection in the 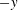$-y$-direction, which produces a quasi-steady wedge-shaped structure with significant anticyclonic velocity 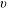$v$, followed by a spin-up reduction of 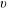$v$ accompanied by a slow $x$ drift, and oscillation. The theoretical model aims to provide useful insights and approximations concerning the formation time and shape of wedge, and the subsequent spin-up effect. The main parameter is the Coriolis number, 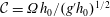${\mathcal{C}}=\unicode[STIX]{x1D6FA}h_{0}/(g^{\prime }h_{0})^{1/2}$, where 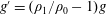$g^{\prime }=(\unicode[STIX]{x1D70C}_{1}/\unicode[STIX]{x1D70C}_{0}-1)g$ is the reduced gravity. The DNS results are focused on a range of relatively small Coriolis numbers, 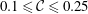$0.1\leqslant {\mathcal{C}}\leqslant 0.25$ (i.e. Rossby number 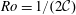$Ro=1/(2{\mathcal{C}})$ in the range 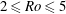$2\leqslant Ro\leqslant 5$), and a large range of Schmidt numbers 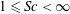$1\leqslant Sc<\infty$; the Reynolds number is large in all cases. The current spreads out in the $x$ direction until it is arrested by the Coriolis effect (in 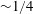${\sim}1/4$ revolution of the system). A complex motion develops about this state. First, we record oscillations on the inertial time scale 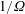$1/\unicode[STIX]{x1D6FA}$ (which are a part of the geostrophic adjustment), accompanied by vortices at the interface. Second, we note the spread of the wedge on a significantly longer time scale; this is an indirect spin-up effect – mixing and entrainment reduce the lateral (angular) velocity, which in turn decreases the Coriolis support to the 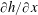$\unicode[STIX]{x2202}h/\unicode[STIX]{x2202}x$ slope of the wedge shape. Contrary to non-rotating gravity currents, the front does not remain sharp as it is subject to (i) local stretching along the streamwise direction and (ii) convective mixing due to Kelvin–Helmholtz vortices generated by shear along the spanwise direction and stemming from Coriolis effects. The theoretical model predicts that the length of the wedge scales as 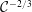${\mathcal{C}}^{-2/3}$ (in contrast to the Rossby radius 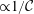$\propto 1/{\mathcal{C}}$ which is relevant for large 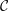${\mathcal{C}}$; and in contrast to 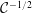${\mathcal{C}}^{-1/2}$ for the axisymmetric lens).

JFM classification

Geophysical and Geological Flows: Gravity currents Geophysical and Geological Flows: Rotating flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 867 , 25 May 2019 , pp. 114 - 145
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alahyari, A. & Longmire, E. 1996 Development and structure of a gravity current head. Exp. Fluids 20, 410–416.Google Scholar
Allen, J. 1971 Mixing at turbidity current heads, and its geological implications. J. Sedim. Petrol. 41 (1), 97–113.Google Scholar
Bonometti, T. & Balachandar, S. 2008 Effect of Schmidt number on the structure and propagation of density currents. Theor. Comput. Fluid Dyn. 22 (5), 341–361.Google Scholar
Bonometti, T., Balachandar, S. & Magnaudet, J. 2008 Wall effects in non-Boussinesq density currents. J. Fluid Mech. 616, 445–475.Google Scholar
Bonometti, T. & Magnaudet, J. 2007 An interface-capturing method for incompressible two-phase flows. Validation and application to bubble dynamics. Intl J. Multiphase Flow 33 (2), 109–133.Google Scholar
Cantero, M., Balachandar, S. & García, M. 2007a Highly resolved simulations of cylindrical density currents. J. Fluid Mech. 590, 437–469.Google Scholar
Cantero, M., Balachandar, S., García, M. & Bock, D. 2008 Turbulent structures in planar gravity currents and their influence of the flow dynamics. J. Geophys. Res. 113, C08018.Google Scholar
Cantero, M., Balachandar, S., García, M. & Ferry, J. 2006 Direct numerical simulations of planar and cyindrical density currents. Trans. ASME J. Appl. Mech. 73, 923–930.Google Scholar
Cantero, M., Lee, J. R., Balachandar, S. & García, M. 2007b On the front velocity of gravity currents. J. Fluid Mech. 586, 1–39.Google Scholar
Cantero, M. I., Cantelli, A., Pirmez, C., Balachandar, S., Mohrig, D., Hickson, T. A., Yeh, T., Naruse, H. & Parker, G. 2012 Emplacement of massive turbidities linked to extinction of turbulence in turbidity currents. Nat. Geosci. 5, 42–45.Google Scholar
Canuto, C., Hussaini, M., Quarteroni, A. & Zang, T. 1988 Spectral Methods in Fluid Dynamics. Springer, 557 pp.Google Scholar
Clarke, J. E. H. 2016 First wide-angle view of channelized turbidity currents links migrating cyclic steps to flow characteristics. Nat. Commun. 7, 11896.Google Scholar
Dai, A. & Wu, C.-S. 2016 High-resolution simulations of cylindrical gravity currents in a rotating system. J. Fluid Mech. 806, 71–101.Google Scholar
Dai, A. & Wu, C.-S. 2018 High-resolution simulations of unstable cylindrical gravity currents undergoing wandering and splitting motions in a rotating system. Phys. Fluids 30 (2), 026601.Google Scholar
Durran, D. 1999 Numerical Methods for Wave Equations in Geophysical Fluid Dynamics. Springer.Google Scholar
Fannelop, T. & Waldman, G. 1971 The dynamics of oil slicks – or ‘creeping crude’. AIAA J. 41, 1–10.Google Scholar
García, M. & Parsons, J. 1996 Mixing at the front of gravity currents. Dyn. Atmos. Oceans 24, 197–205.Google Scholar
Greenspan, H. P.1968 The theory of rotating fluids. Tech. Rep. Massachusetts Inst. of Tech. Cambridge Dept. of Mathematics.Google Scholar
Griffiths, R. W. 1986 Gravity currents in rotating systems. Annu. Rev. Fluid Mech. 18 (1), 59–89.Google Scholar
Hacker, J., Linden, P. F. & Dalziel, S. B. 1996 Mixing in lock-release gravity currents. Dyn. Atmos. Oceans 24 (1–4), 183–195.Google Scholar
Hallworth, M., Huppert, H. & Ungarish, M. 2001 Axisymmetric gravity currents in a rotating system: experimental and numerical investigations. J. Fluid Mech. 447, 1–29.Google Scholar
Härtel, C., Meiburg, E. & Necker, F. 2000 Analysis and direct numerical simulation of the flow at a gravity-current head. Part 1. Flow topology and front speed for slip and no-slip boundaries. J. Fluid Mech. 418, 189–212.Google Scholar
Hickin, E. J. 1989 Contemporary squamish river sediment flux to Howe sound, British Columbia. Can. J. Earth Sci. 26 (10), 1953–1963.Google Scholar
Hogg, A. M., Ivey, G. N. & Winters, K. B. 2001 Hydraulics and mixing in controlled exchange flows. J. Geophys. Res. 106 (C1), 959–972.Google Scholar
Hoult, D. 1972 Oil spreading in the sea. Annu. Rev. Fluid Mech. 4, 341–368.Google Scholar
Hunt, J. C. R., Pacheco, J. R., Mahalov, A. & Fernando, H. J. S. 2005 Effects of rotation and sloping terrain on the fronts of density currents. J. Fluid Mech. 537, 285–315.Google Scholar
Huppert, H. & Simpson, J. 1980 The slumping of gravity currents. J. Fluid Mech. 99, 785–799.Google Scholar
Ivey, G. N., Winters, K. B. & Koseff, J. R. 2008 Density stratification, turbulence, but how much mixing? Annu. Rev. Fluid Mech. 40, 169–184.Google Scholar
Marino, B., Thomas, L. & Linden, P. 2005 The front condition for gravity currents. J. Fluid Mech. 536, 49–78.Google Scholar
Parsons, J. & García, M. 1998 Similarity of gravity current fronts. Phys. Fluids 10 (12), 3209–3213.Google Scholar
Patterson, M., Simpson, J., Dalziel, S. & van Heijst, G. 2006 Vortical motion in the head of an axisymmetric gravity current. Phys. Fluids 18, 046601.Google Scholar
Peltier, W. R. & Caulfield, C. P. 2003 Mixing efficiency in stratified shear flows. Annu. Rev. Fluid Mech. 35, 135–167.Google Scholar
Prastowo, T., Griffiths, R. W., Hughes, G. O. & Hogg, A. M. 2008 Mixing efficiency in controlled exchange flows. J. Fluid Mech. 600, 235–244.Google Scholar
Salehipour, H. & Peltier, W. R. 2015 Diapycnal diffusivity, turbulent Prandtl number and mixing efficiency in Boussinesq stratified turbulence. J. Fluid Mech. 775, 464–500.Google Scholar
Salinas, J. S., Cantero, M. I. & Dari, E. A. 2014 Simulación directa de turbulencia en corrientes de gravedad con efecto Coriolis. RIBAGUA-Revista Iberoamericana del Agua 1 (1), 26–37.Google Scholar
Salinas, J. S., Cantero, M. I., Dari, E. A. & Bonometti, T. 2018 Turbulent structures in cylindrical density currents in a rotating frame of reference. J. Turbul. 19 (6), 463–492.Google Scholar
Shin, J., Dalziel, S. & Linden, P. 2004 Gravity currents produced by lock exchange. J. Fluid Mech. 521, 1–34.Google Scholar
Simpson, J. 1972 Effects of the lower boundary on the head of a gravity current. J. Fluid Mech. 53 (4), 759–768.Google Scholar
Simpson, J. & Britter, R. 1979 The dynamics of the head of a gravity current advancing over a horizontal surface. J. Fluid Mech. 94, 477–495.Google Scholar
Stegner, A., Bouruet-Aubertot, P. & Pichon, T. 2004 Nonlinear adjustment of density fronts. Part 1. The Rossby scenario and the experimental reality. J. Fluid Mech. 502, 335–360.Google Scholar
Stuart, G. A., Sundermeyer, M. A. & Hebert, D. 2011 On the geostrophic adjustment of an isolated lens: dependence on Burger number and initial geometry. J. Phys. Oceanogr. 41 (4), 725–741.Google Scholar
Ungarish, M. 2009 An Introduction to Gravity Currents and Intrusions. CRC Press.Google Scholar
Verzicco, R., Lalli, F. & Campana, E. 1997 Dynamics of baroclinic vortices in a rotating, stratified fluid: a numerical study. Phys. Fluids 9 (2), 419–432.Google Scholar
Zalesak, S. T. 1979 Fully multidimensional flux-corrected transport algorithms for fluids. J. Comput. Phys. 31, 335–362.Google Scholar

Salinas et al. supplementary movie 1

Effect of the Coriolis number - cases (from top to bottom) S1-C25-F, S1-C15-F, S1-C10-F - side view of spanwise averaged density (Re=4000, Sc=1, free-slip)

Download Salinas et al. supplementary movie 1(Video)
Video 16.2 MB

Salinas et al. supplementary movie 2

Case S1-C15-F - top view of bottom density distribution (C=0.15, Ro=3.3, Re=4000, Sc=1, free-slip)

Download Salinas et al. supplementary movie 2(Video)
Video 5.9 MB

Salinas et al. supplementary movie 3

Case S5-C15-N - general view of the density surface rho=0.05 (C=0.15, Ro=3.3, Re=4000, Sc=5, no-slip)

Download Salinas et al. supplementary movie 3(Video)
Video 11.4 MB

Salinas et al. supplementary movie 4

Effect of the top-bottom boundary conditions - cases (from top to bottom) S1-C15-F, S1-C15-N - side view of spanwise averaged density (C=0.15, Ro=3.3, Re=4000, Sc=1)

Download Salinas et al. supplementary movie 4(Video)
Video 14.9 MB

Salinas et al. supplementary movie 5

Case SI-C15-N - general view of the density surfaces rho=0.1 and rho=0.9 (C=0.15, Ro=3.3, Re=4000, Sc=infinite, no-slip)

Download Salinas et al. supplementary movie 5(Video)
Video 7.1 MB

Salinas et al. supplementary movie 6

Case SI-C15-N - bottom view of the density surfaces rho=0.1 and rho=0.9 (C=0.15, Ro=3.3, Re=4000, Sc=infinite, no-slip)

Download Salinas et al. supplementary movie 6(Video)
Video 11.4 MB

Salinas et al. supplementary movie 7

Effect of the Schmidt number - cases (from top to bottom) S5-C15-N, S1-C15-N - side view of spanwise averaged density (C=0.15, Ro=3.3, Re=4000, no-slip)

Download Salinas et al. supplementary movie 7(Video)
Video 13.1 MB