1. Introduction
We can broadly categorise the flow features in compressible wall-bounded flows into two different kinds: (i) subsonic features that have equivalents in incompressible flows and (ii) supersonic features that have no such equivalents. First, consider the subsonic features. Studies have confirmed the presence of the ubiquitous streaky structures of incompressible flows in compressible flows as well; structures such as the near-wall streaks in the buffer layer of the flow (Kline et al. Reference Kline, Reynolds, Schraub and Runstadler1967) and the large-scale and very-large-scale structures in the logarithmic regions of these flows (see Smits, McKeon & Marusic (Reference Smits, McKeon and Marusic2011) and references therein). There is an ongoing debate on how the length scales of these structures change with increasing Mach number and wall cooling (e.g. Smits et al. Reference Smits, Spina, Alving, Smith, Fernando and Donovan1989; Ganapathisubramani, Clemens & Dolling Reference Ganapathisubramani, Clemens and Dolling2006; Smits & Dussauge Reference Smits and Dussauge2006; Duan, Beekman & Martín Reference Duan, Beekman and Martín2010, Reference Duan, Beekman and Martín2011; Duan & Martin Reference Duan and Martin2011; Pirozzoli & Bernardini Reference Pirozzoli and Bernardini2011; Williams et al. Reference Williams, Sahoo, Baumgartner and Smits2018; Bross, Scharnowski & Kähler Reference Bross, Scharnowski and Kähler2021).
Now consider the second kind of flow features, i.e. the supersonic features that have no equivalents in incompressible flows. Here, we consider the eddy Mach waves, which are free stream pressure fluctuations, and a majority of this manuscript will focus on these structures. These pressure fluctuations cause practical difficulties within wind tunnels used to measure the transition behaviour of test vehicles. The pressure disturbances are radiated from the boundary layers formed on the walls of the wind tunnel and thereafter impact transition measurements (e.g. Laufer Reference Laufer1964; Wagner, Maddalon & Weinstein Reference Wagner, Maddalon and Weinstein1970; Stainback Reference Stainback1971; Pate Reference Pate1978; Schneider Reference Schneider2001). Studies (based on acoustic analogies) have inferred the location of the sources that radiate these Mach waves to be within the buffer layer of the boundary layer (Phillips Reference Phillips1960; Ffowcs Williams Reference Ffowcs Williams1963; Duan, Choudhari & Wu Reference Duan, Choudhari and Wu2014). With increasing Mach number, the intensity of these pressure radiations increases, and they also have larger propagation velocities and shallower orientation angles in the free stream (Laufer Reference Laufer1964; Duan, Choudhari & Zhang Reference Duan, Choudhari and Zhang2016). Wall cooling also impacts this radiation (Zhang, Duan & Choudhari Reference Zhang, Duan and Choudhari2017). Experimental measurements of these free stream radiations are notoriously challenging (e.g. Laufer Reference Laufer1961; Kendall Reference Kendall1970; Stainback Reference Stainback1971; Donaldson & Coulter Reference Donaldson and Coulter1995), and direct numerical simulation (DNS) that properly resolves these structures is expensive owing to the requirement of computational boxes with a large wall-normal extent (e.g. Hu, Morfey & Sandham Reference Hu, Morfey and Sandham2006; Duan et al. Reference Duan, Choudhari and Wu2014, Reference Duan, Choudhari and Zhang2016; Zhang et al. Reference Zhang, Duan and Choudhari2017). It is therefore crucial to obtain models that faithfully represent these structures. Empirically obtained correlations such as the Pate's correlation (Pate & Schueler Reference Pate and Schueler1969) are the typical methods by which the effects of these disturbances are currently modelled for practical purposes.
The literature described above focused on experiment-based and DNS-based investigations of compressible wall-bounded flows. The mathematical modelling of the flow is yet another method that has been employed to analyse these flows, and this will be the approach that will be pursued in the current manuscript. Models have been used to analyse the routes through which these flows transition to turbulence, and one such route is provided by the unstable eigenvalues that emerge from the compressible Navier–Stokes equations linearised around laminar mean profiles. We can categorise these unstable eigenvalues into two different kinds: (1) the first mode eigenvalues, which have an equivalent in the incompressible regime; and (2) the higher mode eigenvalues, which do not have an equivalent in the incompressible regime (e.g. Lees & Lin Reference Lees and Lin1946; Lees & Reshotko Reference Lees and Reshotko1962; Mack Reference Mack1965, Reference Mack1975, Reference Mack1984; Malik Reference Malik1990; Ma & Zhong Reference Ma and Zhong2003; Özgen & Kırcalı Reference Özgen and Kırcalı2008; Fedorov & Tumin Reference Fedorov and Tumin2011). Apart from this analysis of eigenvalues, more recent studies have focused on the non-modal mechanisms that provide an additional route to transition. These non-modal mechanisms can be studied by either computing the optimal initial perturbations that leads to maximum transient growth (e.g. Chang et al. Reference Chang, Malik, Erlebacher and Hussaini1991; Balakumar & Malik Reference Balakumar and Malik1992; Hanifi, Schmid & Henningson Reference Hanifi, Schmid and Henningson1996; Tumin & Reshotko Reference Tumin and Reshotko2001, Reference Tumin and Reshotko2003; Zuccher, Tumin & Reshotko Reference Zuccher, Tumin and Reshotko2006; Tempelmann, Hanifi & Henningson Reference Tempelmann, Hanifi and Henningson2012; Bitter & Shepherd Reference Bitter and Shepherd2014, Reference Bitter and Shepherd2015; Paredes et al. Reference Paredes, Choudhari, Li and Chang2016; Bugeat et al. Reference Bugeat, Chassaing, Robinet and Sagaut2019; Kamal et al. Reference Kamal, Rigas, Lakebrink and Colonius2020, Reference Kamal, Rigas, Lakebrink and Colonius2021) or, as will be pursued in this manuscript, by computing the optimum response of the linearised equations to a forcing (e.g. Cook et al. Reference Cook, Thome, Brock, Nichols and Candler2018; Dwivedi et al. Reference Dwivedi, Sidharth, Candler, Nichols and Jovanović2018; Bugeat et al. Reference Bugeat, Chassaing, Robinet and Sagaut2019; Dawson & McKeon Reference Dawson and McKeon2019; Dwivedi et al. Reference Dwivedi, Sidharth, Nichols, Candler and Jovanović2019). These studies showed that the lift-up mechanism that is responsible for amplifying the ubiquitous streaky structures in incompressible wall-bounded flows, also amplify streaky structures in the compressible counterparts of these flows (e.g. Balakumar & Malik Reference Balakumar and Malik1992; Hanifi et al. Reference Hanifi, Schmid and Henningson1996; Tumin & Reshotko Reference Tumin and Reshotko2001, Reference Tumin and Reshotko2003; Zuccher et al. Reference Zuccher, Tumin and Reshotko2006; Tempelmann et al. Reference Tempelmann, Hanifi and Henningson2012; Bitter & Shepherd Reference Bitter and Shepherd2014, Reference Bitter and Shepherd2015; Paredes et al. Reference Paredes, Choudhari, Li and Chang2016; Bugeat et al. Reference Bugeat, Chassaing, Robinet and Sagaut2019). (See Fedorov (Reference Fedorov2011) and references therein for a review regarding the transition of laminar compressible flows.)
Two more recent studies that consider the modelling of these flows, and that are particularly relevant to the current work, are the studies by Bugeat et al. (Reference Bugeat, Chassaing, Robinet and Sagaut2019) and Bae, Dawson & McKeon (Reference Bae, Dawson and McKeon2020b) that considered laminar and turbulent compressible boundary layers, respectively. These studies used the resolvent analysis framework, where nonlinear terms of the linearised Navier–Stokes equations are considered to be a forcing to the linear equations (e.g. Hwang & Cossu Reference Hwang and Cossu2010; McKeon & Sharma Reference McKeon and Sharma2010; Moarref et al. Reference Moarref, Sharma, Tropp and McKeon2013; Zare, Jovanović & Georgiou Reference Zare, Jovanović and Georgiou2017; Towne, Lozano-Durán & Yang Reference Towne, Lozano-Durán and Yang2020; Morra et al. Reference Morra, Nogueira, Cavalieri and Henningson2021). For compressible boundary layers, Bugeat et al. (Reference Bugeat, Chassaing, Robinet and Sagaut2019) and Bae et al. (Reference Bae, Dawson and McKeon2020b) identified two different kinds of modes that are amplified by the resolvent operator. The first among these are the subsonic modes, identified as the streaks and the first modes by Bugeat et al. (Reference Bugeat, Chassaing, Robinet and Sagaut2019), and these modes have equivalents that have been studied in the incompressible regime (e.g. Mack Reference Mack1984; Hwang & Cossu Reference Hwang and Cossu2010; McKeon & Sharma Reference McKeon and Sharma2010; Sharma & McKeon Reference Sharma and McKeon2013; Moarref et al. Reference Moarref, Jovanović, Tropp, Sharma and McKeon2014). In turbulent boundary layers, Bae et al. (Reference Bae, Dawson and McKeon2020b) found that these subsonic modes can be scaled using the semi-local scaling of compressible flows (Trettel & Larsson Reference Trettel and Larsson2016) such that they follow the trends of the incompressible modes well. The trends of these modes can therefore be predicted using tools developed for the incompressible regime (Dawson & McKeon Reference Dawson and McKeon2020). The second among the two sets of identified modes are the supersonic modes (Bugeat et al. Reference Bugeat, Chassaing, Robinet and Sagaut2019; Bae et al. Reference Bae, Dawson and McKeon2020b). Crucially, these modes are related to the higher order Mack modes (Bugeat et al. Reference Bugeat, Chassaing, Robinet and Sagaut2019; Bae et al. Reference Bae, Dawson and McKeon2020b) identified in the seminal work by Mack (Reference Mack1984), and we will explore this relationship further in the current study. Resolvent analysis predicts the increasing significance of these modes with increasing Mach number (Bae et al. Reference Bae, Dawson and McKeon2020b), consistent with DNS (Duan et al. Reference Duan, Choudhari and Zhang2016). These trends of the subsonic and supersonic modes also hold more generally for the case of boundary layers over cooled walls with a range of wall-cooling ratios (Bae, Dawson & McKeon Reference Bae, Dawson and McKeon2020a).
So far, we have seen that there are both subsonic and supersonic features in compressible boundary layer flows, and that these two features are captured by the mathematical modelling technique of resolvent flow analysis where the nonlinear terms act as a forcing (Bugeat et al. Reference Bugeat, Chassaing, Robinet and Sagaut2019; Bae et al. Reference Bae, Dawson and McKeon2020b). Here, we therefore ask the following question: can we isolate the different forcing mechanisms that generate these subsonic and supersonic modes? Recent studies have shown that understanding the forcing mechanisms, and thereby effectively modelling these mechanisms, is crucial for understanding and building practically useful linearised Navier–Stokes based models of flows (e.g. Jovanović & Bamieh Reference Jovanović and Bamieh2005; Zare et al. Reference Zare, Jovanović and Georgiou2017; Amaral et al. Reference Amaral, Cavalieri, Martini, Jordan and Towne2021; Morra et al. Reference Morra, Nogueira, Cavalieri and Henningson2021; Nogueira et al. Reference Nogueira, Morra, Martini, Cavalieri and Henningson2021; Holford, Lee & Hwang Reference Holford, Lee and Hwang2023). The technique of breaking the forcing into components and analysing the parts has provided insights into various aspects of turbulent flows. For instance, this approach has explained the increased prevalence of channel-wide structures in Couette flows when compared with Poiseuille flows (Illingworth Reference Illingworth2020); why the turbulent kinetic energy in wall-bounded flows peaks at a specific wall-normal location (Morra et al. Reference Morra, Nogueira, Cavalieri and Henningson2021; Nogueira et al. Reference Nogueira, Morra, Martini, Cavalieri and Henningson2021); how a small fraction of the full forcing, which can be empirically modelled, generates the acoustic radiations in a jet (Karban et al. Reference Karban, Bugeat, Towne, Lesshafft, Agarwal and Jordan2023) etc. Here, to analyse the forcing, we will use resolvent analysis. Different from Bugeat et al. (Reference Bugeat, Chassaing, Robinet and Sagaut2019) and Bae et al. (Reference Bae, Dawson and McKeon2020b), we concentrate on identifying the specific components of the forcing to the resolvent operator (i.e. the nonlinear terms of the linearised equations) that are responsible for amplifying the subsonic and supersonic modes, separately. The Helmholtz decomposition of the forcing to the resolvent operator proves to be an instrumental tool for this purpose. The aim is to acquire a fundamental physical understanding of the different amplification mechanisms in the flow through identifying the mechanisms associated with individual forcing components. For the majority of the manuscript, we will consider the simple case of a boundary layer flow with a time-invariant mean flow that varies only in one inhomogeneous spatial (wall-normal) direction, which makes the mathematical analysis tractable and insightful. We also show that the conclusions drawn are applicable for the more general case of a boundary layer with a two-dimensional (2-D) mean flow that varies in two inhomogeneous spatial (streamwise and wall-normal) directions.
Here, we find that the subsonic modes in the compressible flow are forced by the solenoidal component of the forcing alone. The Mach waves, however, have two routes through which they can be amplified: (i) the direct route, where the dilatational component of the forcing to the momentum equations and the forcing to the density and temperature equations are active; and (ii) the indirect route, where the solenoidal forcing excites the Mach waves. In other words, when focusing on the indirect route, the dilatational response from a solenoidal forcing is considered. We find that, while the direct route is the dominant mechanism for amplifying the Mach waves, the indirect route plays a significant role for the Mach waves that are forced by the buffer layer of the flow. While there are alternate insightful analysis techniques that have been used to probe the different mechanisms in these flows, such as the examination of the interaction of vortical and acoustic mechanisms through DNS and linear stability theory by Unnikrishnan & Gaitonde (Reference Unnikrishnan and Gaitonde2019), to the best of our knowledge, these techniques do not explain the two distinct routes of forcing the Mach waves and the different regions of the flow where these mechanisms operate.
The organisation of the rest of this paper is as follows. We will start with a description of the resolvent analysis of the linearised Navier–Stokes equations and its numerical implementation in § 2. Section 2.5 will then discuss Helmholtz decomposition, a technique that will be frequently employed in this manuscript. In § 3, the subsonic modes will be considered, and the forcing mechanisms that amplify the subsonic modes will be the topic of § 3.2. In § 4, we will then shift our focus to the supersonic resolvent modes, i.e. the resolvent Mach waves. The free stream contribution of these modes and the effect of viscosity on these modes will be discussed in §§ 4.1 and 4.2, respectively. The two routes of amplifying the Mach waves will be discussed in § 5 and the contribution of the two routes across a wide parameter regime will be considered in § 6. In § 7, we will discuss the resolvent Mach waves alongside trends of these waves that are known from DNS. Finally, in § 8, we show that the conclusions drawn are also valid for a boundary layer flow with a two-dimensional mean profile, before concluding the manuscript in § 9. Although most of the study focuses on a Mach ![]() $4$ and friction Reynolds number
$4$ and friction Reynolds number ![]() $400$ turbulent boundary layer over an adiabatic wall, the discussions are more generally applicable to laminar compressible boundary layers (Appendix C) as well as turbulent boundary layers both over adiabatic and cooled walls, for a range of Mach numbers (§ 6).
$400$ turbulent boundary layer over an adiabatic wall, the discussions are more generally applicable to laminar compressible boundary layers (Appendix C) as well as turbulent boundary layers both over adiabatic and cooled walls, for a range of Mach numbers (§ 6).
2. Methods
2.1. Linear model
We consider a compressible boundary layer with the streamwise, wall-normal and spanwise directions given by ![]() $x$,
$x$, ![]() $y$ and
$y$ and ![]() $z$, respectively. Although the development of the boundary layer in the streamwise direction is an important parameter to consider (e.g. Bertolotti, Herbert & Spalart Reference Bertolotti, Herbert and Spalart1992; Govindarajan & Narasimha Reference Govindarajan and Narasimha1995; Ma & Zhong Reference Ma and Zhong2003; Ran et al. Reference Ran, Zare, Hack and Jovanović2019; Ruan & Blanquart Reference Ruan and Blanquart2021), as a first approximation, here, we invoke the parallel flow assumption, where we assume that this streamwise development is slow and therefore neglect its effects. We briefly consider the impact of the streamwise development on the discussions here in § 8 and hope to report on this in more detail in the near future (Stroot & McKeon Reference Stroot and McKeon2022; Stroot, Madhusudanan & McKeon Reference Stroot, Madhusudanan and McKeon2023). Under this parallel flow assumption, along with the spanwise, the streamwise is also a homogeneous direction, and the mean streamwise velocity
$z$, respectively. Although the development of the boundary layer in the streamwise direction is an important parameter to consider (e.g. Bertolotti, Herbert & Spalart Reference Bertolotti, Herbert and Spalart1992; Govindarajan & Narasimha Reference Govindarajan and Narasimha1995; Ma & Zhong Reference Ma and Zhong2003; Ran et al. Reference Ran, Zare, Hack and Jovanović2019; Ruan & Blanquart Reference Ruan and Blanquart2021), as a first approximation, here, we invoke the parallel flow assumption, where we assume that this streamwise development is slow and therefore neglect its effects. We briefly consider the impact of the streamwise development on the discussions here in § 8 and hope to report on this in more detail in the near future (Stroot & McKeon Reference Stroot and McKeon2022; Stroot, Madhusudanan & McKeon Reference Stroot, Madhusudanan and McKeon2023). Under this parallel flow assumption, along with the spanwise, the streamwise is also a homogeneous direction, and the mean streamwise velocity ![]() $\bar {U}(y)$, temperature
$\bar {U}(y)$, temperature ![]() $\bar {\varTheta }(y)$, density
$\bar {\varTheta }(y)$, density ![]() $\bar {\rho }(y)$ and pressure
$\bar {\rho }(y)$ and pressure ![]() $\bar {P}(y)$ are functions of the wall-normal direction alone. Additionally, under this assumption, the mean wall-normal
$\bar {P}(y)$ are functions of the wall-normal direction alone. Additionally, under this assumption, the mean wall-normal ![]() $\bar {V}(y)$ and spanwise
$\bar {V}(y)$ and spanwise ![]() $\bar {W}(y)$ velocities are zero. Fluctuations are defined with respect to these mean quantities, where
$\bar {W}(y)$ velocities are zero. Fluctuations are defined with respect to these mean quantities, where ![]() $u$,
$u$, ![]() $v$ and
$v$ and ![]() $w$ represent the velocity fluctuations in the streamwise, wall-normal and spanwise directions, respectively, and
$w$ represent the velocity fluctuations in the streamwise, wall-normal and spanwise directions, respectively, and ![]() $\rho$,
$\rho$, ![]() $\theta$ and
$\theta$ and ![]() $p$ represent the density, temperature and pressure fluctuations, respectively. A subscript ‘
$p$ represent the density, temperature and pressure fluctuations, respectively. A subscript ‘![]() $\infty$’ denotes free stream quantities and a subscript ‘
$\infty$’ denotes free stream quantities and a subscript ‘![]() $w$’ denotes quantities at the wall. The velocities are non-dimensionalised by
$w$’ denotes quantities at the wall. The velocities are non-dimensionalised by ![]() $U_\infty$, the length scales by the boundary layer thickness
$U_\infty$, the length scales by the boundary layer thickness ![]() $\delta$ and temperature by
$\delta$ and temperature by ![]() $\varTheta _\infty$. A superscript ‘
$\varTheta _\infty$. A superscript ‘![]() $+$’ indicates normalisation of velocities and length scales by the friction velocity
$+$’ indicates normalisation of velocities and length scales by the friction velocity ![]() $u_\tau$ and the friction length scale
$u_\tau$ and the friction length scale ![]() $\mu _w/u_\tau$, respectively. Here,
$\mu _w/u_\tau$, respectively. Here, ![]() $\mu$ is the first coefficient of viscosity.
$\mu$ is the first coefficient of viscosity.
The non-dimensional numbers that define the problem are: (1) the Reynolds number, defined as ![]() $Re=\rho _\infty U_\infty \delta /\mu _\infty$; (2) the free stream Mach number, defined as
$Re=\rho _\infty U_\infty \delta /\mu _\infty$; (2) the free stream Mach number, defined as ![]() $Ma=U_\infty /(\gamma \mathcal {R}\varTheta _\infty )^{1/2}$, where
$Ma=U_\infty /(\gamma \mathcal {R}\varTheta _\infty )^{1/2}$, where ![]() $\gamma$ is the specific heat ratio and
$\gamma$ is the specific heat ratio and ![]() $\mathcal {R}$ is the universal gas constant; and (3) the Prandtl number
$\mathcal {R}$ is the universal gas constant; and (3) the Prandtl number ![]() $Pr=\mu _\infty c_p/\kappa _\infty$, defined using specific heat ratio
$Pr=\mu _\infty c_p/\kappa _\infty$, defined using specific heat ratio ![]() $c_p$ and the thermal conductivity
$c_p$ and the thermal conductivity ![]() $\kappa$. A friction Reynolds number is also defined as
$\kappa$. A friction Reynolds number is also defined as ![]() $Re_\tau =\rho _w u_\tau \delta /\mu _w$. Throughout this study,
$Re_\tau =\rho _w u_\tau \delta /\mu _w$. Throughout this study, ![]() $Pr=0.72$ and
$Pr=0.72$ and ![]() $\gamma =1.4$ are kept fixed. Flows over both adiabatic walls as well as over cooled walls are considered, and boundary layers over cooled walls are characterised by the ratio
$\gamma =1.4$ are kept fixed. Flows over both adiabatic walls as well as over cooled walls are considered, and boundary layers over cooled walls are characterised by the ratio ![]() $\varTheta _w/\varTheta _{ad}$, where
$\varTheta _w/\varTheta _{ad}$, where ![]() $\varTheta _{w}$ is the wall temperature and
$\varTheta _{w}$ is the wall temperature and ![]() $\varTheta _{ad}$ is the wall temperature in the case of the flow over adiabatic walls. For most of this work, we consider a
$\varTheta _{ad}$ is the wall temperature in the case of the flow over adiabatic walls. For most of this work, we consider a ![]() $Ma=4$,
$Ma=4$, ![]() $Re_\tau =400$ turbulent boundary layer over an adiabatic wall. However, we will show that the substance of the discussion here is applicable to turbulent boundary layers over adiabatic as well as cooled walls and over a range of Mach numbers (in § 6), as well as to laminar boundary layers (in Appendix C).
$Re_\tau =400$ turbulent boundary layer over an adiabatic wall. However, we will show that the substance of the discussion here is applicable to turbulent boundary layers over adiabatic as well as cooled walls and over a range of Mach numbers (in § 6), as well as to laminar boundary layers (in Appendix C).
We linearise the Navier–Stokes equation around the mean state ![]() $(\bar {U}(y), 0, 0, \bar {\rho }(y), \bar {\varTheta }(y))$ and obtain the equations for the fluctuations as
$(\bar {U}(y), 0, 0, \bar {\rho }(y), \bar {\varTheta }(y))$ and obtain the equations for the fluctuations as
 \begin{align} \bar{\rho}\frac{\partial u_i}{\partial t} &={-} \bar{\rho} \bar{U} \frac{\partial u_i}{\partial x} - \bar{\rho} \frac{{\rm d} \bar{U}}{{\rm d}y} v \hat{i} -\frac{1}{\gamma Ma^2} \left[ \bar{\varTheta} \frac{\partial \rho}{\partial x_i} + \frac{{\rm d}\bar{\varTheta}}{{\rm d}y} \rho \hat{j} + \bar{\rho} \frac{\partial \theta}{\partial x_i} + \frac{{\rm d}\bar{\rho}}{{\rm d}y} \theta \hat{j} \right] \nonumber\\ &\quad + \frac{1}{Re} \left[\vphantom{\left.+ \frac{\partial \bar{\lambda}}{\partial y} \frac{\partial u_k}{\partial x_k} \hat{j} + \frac{\partial \bar{\mu}}{\partial \bar{\varTheta}} \frac{{\rm d}^2 \bar{U}}{{{\rm d}y}^2} \theta \hat{i} + \bar{\mu} \frac{\partial^2 u_i}{\partial x_j\partial x_j} + (\bar{\mu}+\bar{\lambda}) \frac{\partial^2 u_j}{\partial x_j\partial x_i} \right]} \frac{\partial \bar{\mu}}{\partial \bar{\varTheta}} \frac{{\rm d}\bar{U}}{{\rm d}y} \left( \frac{\partial \theta}{\partial y} \hat{i} + \frac{\partial \theta}{\partial x} \hat{j} \right) + \frac{\partial^2 \bar{\mu}}{\partial \bar{\varTheta}^2} \frac{{\rm d}\bar{\varTheta}}{{\rm d}y} \frac{d \bar{U}}{{\rm d}y} \theta \hat{i} + \frac{\partial \bar{\mu}}{\partial y} \left( \frac{\partial u_i}{\partial y} + \frac{\partial v}{\partial x_i} \right) \right. \nonumber\\ &\quad \left.+ \frac{\partial \bar{\lambda}}{\partial y} \frac{\partial u_k}{\partial x_k} \hat{j} + \frac{\partial \bar{\mu}}{\partial \bar{\varTheta}} \frac{{\rm d}^2 \bar{U}}{{{\rm d}y}^2} \theta \hat{i} + \bar{\mu} \frac{\partial^2 u_i}{\partial x_j\partial x_j} + (\bar{\mu}+\bar{\lambda}) \frac{\partial^2 u_j}{\partial x_j\partial x_i} \right] + f_{u_i}, \end{align}
\begin{align} \bar{\rho}\frac{\partial u_i}{\partial t} &={-} \bar{\rho} \bar{U} \frac{\partial u_i}{\partial x} - \bar{\rho} \frac{{\rm d} \bar{U}}{{\rm d}y} v \hat{i} -\frac{1}{\gamma Ma^2} \left[ \bar{\varTheta} \frac{\partial \rho}{\partial x_i} + \frac{{\rm d}\bar{\varTheta}}{{\rm d}y} \rho \hat{j} + \bar{\rho} \frac{\partial \theta}{\partial x_i} + \frac{{\rm d}\bar{\rho}}{{\rm d}y} \theta \hat{j} \right] \nonumber\\ &\quad + \frac{1}{Re} \left[\vphantom{\left.+ \frac{\partial \bar{\lambda}}{\partial y} \frac{\partial u_k}{\partial x_k} \hat{j} + \frac{\partial \bar{\mu}}{\partial \bar{\varTheta}} \frac{{\rm d}^2 \bar{U}}{{{\rm d}y}^2} \theta \hat{i} + \bar{\mu} \frac{\partial^2 u_i}{\partial x_j\partial x_j} + (\bar{\mu}+\bar{\lambda}) \frac{\partial^2 u_j}{\partial x_j\partial x_i} \right]} \frac{\partial \bar{\mu}}{\partial \bar{\varTheta}} \frac{{\rm d}\bar{U}}{{\rm d}y} \left( \frac{\partial \theta}{\partial y} \hat{i} + \frac{\partial \theta}{\partial x} \hat{j} \right) + \frac{\partial^2 \bar{\mu}}{\partial \bar{\varTheta}^2} \frac{{\rm d}\bar{\varTheta}}{{\rm d}y} \frac{d \bar{U}}{{\rm d}y} \theta \hat{i} + \frac{\partial \bar{\mu}}{\partial y} \left( \frac{\partial u_i}{\partial y} + \frac{\partial v}{\partial x_i} \right) \right. \nonumber\\ &\quad \left.+ \frac{\partial \bar{\lambda}}{\partial y} \frac{\partial u_k}{\partial x_k} \hat{j} + \frac{\partial \bar{\mu}}{\partial \bar{\varTheta}} \frac{{\rm d}^2 \bar{U}}{{{\rm d}y}^2} \theta \hat{i} + \bar{\mu} \frac{\partial^2 u_i}{\partial x_j\partial x_j} + (\bar{\mu}+\bar{\lambda}) \frac{\partial^2 u_j}{\partial x_j\partial x_i} \right] + f_{u_i}, \end{align} \begin{align} \bar{\rho}\frac{\partial \theta}{\partial t} &={-} \bar{\rho} \bar{U} \frac{\partial \theta}{\partial x} - \bar{\rho} \frac{{\rm d} \bar{\varTheta}}{{\rm d}y} v - (\gamma-1) \frac{\partial u_j }{\partial x_j} \nonumber\\ &\quad + \frac{\gamma}{PrRe} \left[ 2 \frac{\partial \bar{\mu}}{\partial y} \frac{\partial \theta}{\partial y} + \frac{\partial^2 \bar{\mu}}{\partial \bar{\varTheta}^2} \left( \frac{{\rm d}\bar{\varTheta}}{{\rm d}y} \right)^2 \theta+ \bar{\mu} \frac{\partial^2 \theta}{\partial x_j \partial x_j} + \frac{\partial \bar{\mu}}{\partial \bar{\varTheta}} \frac{{\rm d}^2 \bar{\varTheta}}{{{\rm d}y}^2} \theta \right]\nonumber\\ &\quad + \frac{\gamma(\gamma-1)Ma^2}{Re} \left[ 2 \bar{\mu} \frac{{\rm d}\bar{U}}{{\rm d}y} \left( \frac{\partial u}{\partial y}+ \frac{\partial v}{\partial x} \right) + \frac{\partial \bar{\mu}}{\partial \bar{\varTheta}} \left(\frac{{\rm d}\bar{U}}{{\rm d}y}\right)^2 \theta \right]+ f_\theta. \end{align}
\begin{align} \bar{\rho}\frac{\partial \theta}{\partial t} &={-} \bar{\rho} \bar{U} \frac{\partial \theta}{\partial x} - \bar{\rho} \frac{{\rm d} \bar{\varTheta}}{{\rm d}y} v - (\gamma-1) \frac{\partial u_j }{\partial x_j} \nonumber\\ &\quad + \frac{\gamma}{PrRe} \left[ 2 \frac{\partial \bar{\mu}}{\partial y} \frac{\partial \theta}{\partial y} + \frac{\partial^2 \bar{\mu}}{\partial \bar{\varTheta}^2} \left( \frac{{\rm d}\bar{\varTheta}}{{\rm d}y} \right)^2 \theta+ \bar{\mu} \frac{\partial^2 \theta}{\partial x_j \partial x_j} + \frac{\partial \bar{\mu}}{\partial \bar{\varTheta}} \frac{{\rm d}^2 \bar{\varTheta}}{{{\rm d}y}^2} \theta \right]\nonumber\\ &\quad + \frac{\gamma(\gamma-1)Ma^2}{Re} \left[ 2 \bar{\mu} \frac{{\rm d}\bar{U}}{{\rm d}y} \left( \frac{\partial u}{\partial y}+ \frac{\partial v}{\partial x} \right) + \frac{\partial \bar{\mu}}{\partial \bar{\varTheta}} \left(\frac{{\rm d}\bar{U}}{{\rm d}y}\right)^2 \theta \right]+ f_\theta. \end{align}
Here, all the nonlinear terms of the equation are represented by ![]() $\boldsymbol {f}=(f_u,f_v,f_w,f_\rho,f_\theta )$, where
$\boldsymbol {f}=(f_u,f_v,f_w,f_\rho,f_\theta )$, where ![]() $f_u$,
$f_u$, ![]() $f_v$ and
$f_v$ and ![]() $f_w$ represent the nonlinear terms in the momentum equations, and
$f_w$ represent the nonlinear terms in the momentum equations, and ![]() $f_\rho$ and
$f_\rho$ and ![]() $f_\theta$ represent the nonlinear terms in the continuity and the energy equations, respectively. In (2.1),
$f_\theta$ represent the nonlinear terms in the continuity and the energy equations, respectively. In (2.1), ![]() $(u_1,u_2,u_3)$ represents
$(u_1,u_2,u_3)$ represents ![]() $(u,v,w)$ and
$(u,v,w)$ and ![]() $(x_1,x_2,x_3)$ represents
$(x_1,x_2,x_3)$ represents ![]() $(x,y,z)$. (It should be noted that, for this linearisation, we have not assumed the fluctuations to be small and, instead, they can assume any arbitrary value). Unit vectors along
$(x,y,z)$. (It should be noted that, for this linearisation, we have not assumed the fluctuations to be small and, instead, they can assume any arbitrary value). Unit vectors along ![]() $x$,
$x$, ![]() $y$ and
$y$ and ![]() $z$ are
$z$ are ![]() $\hat {i}$,
$\hat {i}$, ![]() $\hat {j}$ and
$\hat {j}$ and ![]() $\hat {k}$, respectively. In addition to the equations in (2.1), we also have the linearised equation of state
$\hat {k}$, respectively. In addition to the equations in (2.1), we also have the linearised equation of state ![]() $p=\bar {\rho } \theta + \bar {\varTheta } \rho$. The equations are scaled such that the mean pressure
$p=\bar {\rho } \theta + \bar {\varTheta } \rho$. The equations are scaled such that the mean pressure ![]() $\bar {P}=1$ and, therefore, the mean density is related to the mean temperature as
$\bar {P}=1$ and, therefore, the mean density is related to the mean temperature as ![]() $\bar {\rho }=1/\bar {\varTheta }$. The mean viscosity is obtained as a function of temperature using the Sutherland formula
$\bar {\rho }=1/\bar {\varTheta }$. The mean viscosity is obtained as a function of temperature using the Sutherland formula ![]() $\bar {\mu } = \bar {\varTheta }^{3/2} (1+C)/(\bar {\varTheta }+C)$, where
$\bar {\mu } = \bar {\varTheta }^{3/2} (1+C)/(\bar {\varTheta }+C)$, where ![]() $C = 110.4K/\varTheta _\infty$. The second coefficient of viscosity is given as
$C = 110.4K/\varTheta _\infty$. The second coefficient of viscosity is given as ![]() $\lambda =-2/3\mu$. The subsonic lift-up mechanism (Landahl Reference Landahl1980) and critical-layer mechanism (McKeon & Sharma Reference McKeon and Sharma2010), as well as the supersonic Mach wave generation mechanism (Mack Reference Mack1984), are easily expressed in terms of the primitive variables
$\lambda =-2/3\mu$. The subsonic lift-up mechanism (Landahl Reference Landahl1980) and critical-layer mechanism (McKeon & Sharma Reference McKeon and Sharma2010), as well as the supersonic Mach wave generation mechanism (Mack Reference Mack1984), are easily expressed in terms of the primitive variables ![]() $(u,v,w,\rho,\theta )$ used here. In the future, it would be interesting to see how the discussions here are impacted with a different choice of variables (Karban et al. Reference Karban, Bugeat, Martini, Towne, Cavalieri, Lesshafft, Agarwal, Jordan and Colonius2020).
$(u,v,w,\rho,\theta )$ used here. In the future, it would be interesting to see how the discussions here are impacted with a different choice of variables (Karban et al. Reference Karban, Bugeat, Martini, Towne, Cavalieri, Lesshafft, Agarwal, Jordan and Colonius2020).
2.2. Resolvent operator
We use the linearised equations in (2.1) to derive the resolvent operator for the flow. For this, ![]() $\boldsymbol {u}$,
$\boldsymbol {u}$, ![]() $\rho$,
$\rho$, ![]() $\theta$ and
$\theta$ and ![]() $\boldsymbol {f}$ are considered in terms of their Fourier transforms in the homogeneous streamwise and spanwise directions, as well as in time,
$\boldsymbol {f}$ are considered in terms of their Fourier transforms in the homogeneous streamwise and spanwise directions, as well as in time,
Here, ![]() $l$ represents
$l$ represents ![]() $\boldsymbol {u}$,
$\boldsymbol {u}$, ![]() $\rho$,
$\rho$, ![]() $\theta$ or
$\theta$ or ![]() $\boldsymbol {f}$, and
$\boldsymbol {f}$, and ![]() $\hat {\cdot }$ represents their Fourier transforms. (
$\hat {\cdot }$ represents their Fourier transforms. (![]() $k_x,k_z$) are the streamwise and spanwise wavenumbers, (
$k_x,k_z$) are the streamwise and spanwise wavenumbers, (![]() $\lambda _x,\lambda _z$) are the corresponding wavelengths and
$\lambda _x,\lambda _z$) are the corresponding wavelengths and ![]() $\omega$ is the temporal frequency. The wavenumbers are non-dimensionalised by
$\omega$ is the temporal frequency. The wavenumbers are non-dimensionalised by ![]() $(1/\delta )$ and the wavelengths by
$(1/\delta )$ and the wavelengths by ![]() $\delta$. The temporal frequency
$\delta$. The temporal frequency ![]() $\omega$ can be written in terms of a phase speed
$\omega$ can be written in terms of a phase speed ![]() $c$ as
$c$ as ![]() $\omega =-c k_x$. In terms of these Fourier transforms, (2.1) are written as
$\omega =-c k_x$. In terms of these Fourier transforms, (2.1) are written as
The matrix ![]() $\boldsymbol {A}$ contains the finite-dimensional discrete approximations of the linearised momentum, continuity and energy equations from (2.1) in terms of the Fourier transforms, where the derivatives
$\boldsymbol {A}$ contains the finite-dimensional discrete approximations of the linearised momentum, continuity and energy equations from (2.1) in terms of the Fourier transforms, where the derivatives ![]() $(\partial /\partial _x,\partial /\partial _y,\partial /\partial _z)$ become
$(\partial /\partial _x,\partial /\partial _y,\partial /\partial _z)$ become ![]() $({\rm i}k_x,\partial /\partial _y,{\rm i}k_z)$ (for the different terms of the matrix
$({\rm i}k_x,\partial /\partial _y,{\rm i}k_z)$ (for the different terms of the matrix ![]() $\boldsymbol {A}$, see Dawson & McKeon Reference Dawson and McKeon2019). The vector
$\boldsymbol {A}$, see Dawson & McKeon Reference Dawson and McKeon2019). The vector ![]() $\hat {\boldsymbol {q}}=(\hat {u},\hat {v},\hat {w},\hat {\rho },\hat {\theta })$ contains the state variables and
$\hat {\boldsymbol {q}}=(\hat {u},\hat {v},\hat {w},\hat {\rho },\hat {\theta })$ contains the state variables and ![]() $\hat {\boldsymbol {f}}=(\,\hat {f}_u,\hat {f}_v,\hat {f}_w,\hat {f}_\rho,\hat {f}_\theta )$ the nonlinear terms of the equations.
$\hat {\boldsymbol {f}}=(\,\hat {f}_u,\hat {f}_v,\hat {f}_w,\hat {f}_\rho,\hat {f}_\theta )$ the nonlinear terms of the equations.
To analyse (2.3), we need to choose a norm and here we employ the commonly adopted Chu norm ![]() $E$ defined as (Chu Reference Chu1965; Hanifi et al. Reference Hanifi, Schmid and Henningson1996)
$E$ defined as (Chu Reference Chu1965; Hanifi et al. Reference Hanifi, Schmid and Henningson1996)
where ![]() ${\cdot }^*$ represents a complex conjugate. The Chu norm as well as the weights corresponding to the non-uniform grid used here are incorporated within a weight matrix
${\cdot }^*$ represents a complex conjugate. The Chu norm as well as the weights corresponding to the non-uniform grid used here are incorporated within a weight matrix ![]() $\boldsymbol {W}$. The discrete inner product used becomes
$\boldsymbol {W}$. The discrete inner product used becomes ![]() $\langle \hat {\boldsymbol {q}}_1, \hat {\boldsymbol {q}}_2 \rangle = \hat {\boldsymbol {q}}_1^* \boldsymbol {W} \hat {\boldsymbol {q}}_2$. Equation (2.3) can now be re-written as
$\langle \hat {\boldsymbol {q}}_1, \hat {\boldsymbol {q}}_2 \rangle = \hat {\boldsymbol {q}}_1^* \boldsymbol {W} \hat {\boldsymbol {q}}_2$. Equation (2.3) can now be re-written as
 \begin{equation} \hat{\boldsymbol{q}} = \underbrace{\left[ \boldsymbol{W}^{1/2}\left( {\rm i}\omega \boldsymbol{I} - \boldsymbol{A}(k_x,k_z) \right)^{{-}1} \boldsymbol{W}^{{-}1/2} \right]}_{\boldsymbol{H}(k_x,k_z,\omega)} \hat{\boldsymbol{f}}, \end{equation}
\begin{equation} \hat{\boldsymbol{q}} = \underbrace{\left[ \boldsymbol{W}^{1/2}\left( {\rm i}\omega \boldsymbol{I} - \boldsymbol{A}(k_x,k_z) \right)^{{-}1} \boldsymbol{W}^{{-}1/2} \right]}_{\boldsymbol{H}(k_x,k_z,\omega)} \hat{\boldsymbol{f}}, \end{equation}
where ![]() $\boldsymbol {I}$ is the identity matrix. The transfer kernel
$\boldsymbol {I}$ is the identity matrix. The transfer kernel ![]() $\boldsymbol {H}(k_x,k_z,\omega )$ is the resolvent operator of the flow and it maps the nonlinear terms
$\boldsymbol {H}(k_x,k_z,\omega )$ is the resolvent operator of the flow and it maps the nonlinear terms ![]() $\hat {\boldsymbol {f}}$ to the state variables
$\hat {\boldsymbol {f}}$ to the state variables ![]() $\hat {\boldsymbol {q}}$.
$\hat {\boldsymbol {q}}$.
One of the benefits of using resolvent analysis is the ability to ‘mask’ the resolvent. Whereas the full resolvent admits a response and forcing in the entire spatial domain considered, the masked resolvent, when the masking is in the response, restricts the response to lie within a specific wall-normal region. If the masking is in the forcing, the forcing in the model is restricted to lie within a specific wall-normal region. For instance, we can consider the resolvent where the response lies solely in the free stream, or where the forcing lies exclusively in the buffer layer of the flow (see § 7). For this masking, matrices ![]() $\boldsymbol {B}$ and
$\boldsymbol {B}$ and ![]() $\boldsymbol {C}$ are introduced to (2.3) such that
$\boldsymbol {C}$ are introduced to (2.3) such that
If ![]() $\boldsymbol {B}$ and
$\boldsymbol {B}$ and ![]() $\boldsymbol {C}$ equal identity, we get back (2.3). To restrict the forcing or response to defined wall-normal regions, weightings, such as those introduced by Nogueira et al. (Reference Nogueira, Cavalieri, Hanifi and Henningson2020), can be incorporated in
$\boldsymbol {C}$ equal identity, we get back (2.3). To restrict the forcing or response to defined wall-normal regions, weightings, such as those introduced by Nogueira et al. (Reference Nogueira, Cavalieri, Hanifi and Henningson2020), can be incorporated in ![]() $\boldsymbol {B}$ or
$\boldsymbol {B}$ or ![]() $\boldsymbol {C}$. The masked resolvent operator
$\boldsymbol {C}$. The masked resolvent operator ![]() $\boldsymbol {H}_{mask}(k_x,k_z,\omega )$ then becomes
$\boldsymbol {H}_{mask}(k_x,k_z,\omega )$ then becomes
 \begin{equation} \hat{\boldsymbol{q}} = \underbrace{ \boldsymbol{C} \left[ \boldsymbol{W}^{1/2}\left( {\rm i}\omega \boldsymbol{I} - \boldsymbol{A}(k_x,k_z) \right)^{{-}1} \boldsymbol{W}^{{-}1/2} \right] \boldsymbol{B}}_{\boldsymbol{H}_{mask}(k_x,k_z,\omega) } \hat{\boldsymbol{f}}. \end{equation}
\begin{equation} \hat{\boldsymbol{q}} = \underbrace{ \boldsymbol{C} \left[ \boldsymbol{W}^{1/2}\left( {\rm i}\omega \boldsymbol{I} - \boldsymbol{A}(k_x,k_z) \right)^{{-}1} \boldsymbol{W}^{{-}1/2} \right] \boldsymbol{B}}_{\boldsymbol{H}_{mask}(k_x,k_z,\omega) } \hat{\boldsymbol{f}}. \end{equation}2.3. Singular value decomposition of the resolvent operator
To analyse the resolvent operator in (2.5), we perform a singular value decomposition (SVD)
 \begin{equation} \boldsymbol{H}(k_x,k_z,c) = \sum_{i=1}^{5N} \boldsymbol{\psi}_i(y) \sigma_i \boldsymbol{\phi}_i(y). \end{equation}
\begin{equation} \boldsymbol{H}(k_x,k_z,c) = \sum_{i=1}^{5N} \boldsymbol{\psi}_i(y) \sigma_i \boldsymbol{\phi}_i(y). \end{equation}
Here, ![]() $N$ represents the number of grid points used to discretise the wall-normal direction. The singular values
$N$ represents the number of grid points used to discretise the wall-normal direction. The singular values ![]() $\sigma _i$ are arranged such that
$\sigma _i$ are arranged such that ![]() $\sigma _i \geqslant \sigma _{i+1}$. The left singular vectors
$\sigma _i \geqslant \sigma _{i+1}$. The left singular vectors ![]() $\boldsymbol {\psi }_i(y)$ are the resolvent response modes and the right singular vectors
$\boldsymbol {\psi }_i(y)$ are the resolvent response modes and the right singular vectors ![]() $\boldsymbol {\phi }_i(y)$ are the resolvent forcing modes. Therefore, a forcing to the resolvent operator along
$\boldsymbol {\phi }_i(y)$ are the resolvent forcing modes. Therefore, a forcing to the resolvent operator along ![]() $\boldsymbol {\phi }_i$ will give a response along
$\boldsymbol {\phi }_i$ will give a response along ![]() $\boldsymbol {\psi }_i$ amplified by a factor of
$\boldsymbol {\psi }_i$ amplified by a factor of ![]() $\sigma _i$. The most sensitive forcing direction is
$\sigma _i$. The most sensitive forcing direction is ![]() $\boldsymbol {\phi }_1$ that is associated with the largest singular value
$\boldsymbol {\phi }_1$ that is associated with the largest singular value ![]() $\sigma _1$, and the corresponding most amplified response direction is
$\sigma _1$, and the corresponding most amplified response direction is ![]() $\boldsymbol {\psi }_1$. If we assume that the forcing
$\boldsymbol {\psi }_1$. If we assume that the forcing ![]() $\hat {\boldsymbol {f}}$ in (2.5) is unit-amplitude and broadband across
$\hat {\boldsymbol {f}}$ in (2.5) is unit-amplitude and broadband across ![]() $(k_x,k_z)$, then the regions of the wavenumber space where
$(k_x,k_z)$, then the regions of the wavenumber space where ![]() $\sigma _1$ is high represents structures that are energetic.
$\sigma _1$ is high represents structures that are energetic.
The right and left singular vectors form a complete basis. Therefore, any forcing ![]() $\hat {\boldsymbol {f}}$ and any response
$\hat {\boldsymbol {f}}$ and any response ![]() $\hat {\boldsymbol {q}}$ can be expressed in terms of these basis vectors as
$\hat {\boldsymbol {q}}$ can be expressed in terms of these basis vectors as
Let us assume the forcing is approximately stochastic and therefore does not have any preferred direction. Then, in scenarios where ![]() $\sigma _1 \gg \sigma _{i\neq 1}$, it is possible that a rank-1 model, where
$\sigma _1 \gg \sigma _{i\neq 1}$, it is possible that a rank-1 model, where ![]() $\hat {\boldsymbol {f}} \approx \chi _1 \boldsymbol {\phi }_1$ and
$\hat {\boldsymbol {f}} \approx \chi _1 \boldsymbol {\phi }_1$ and ![]() $\hat {\boldsymbol {u}} \approx \chi _1 \sigma _1 \boldsymbol {\psi }_1$ captures the flow reasonably well (Beneddine et al. Reference Beneddine, Sipp, Arnault, Dandois and Lesshafft2016; Towne, Schmidt & Colonius Reference Towne, Schmidt and Colonius2018). This is indicative of the existence of a dominant physical mechanism that is giving rise to the resolvent amplification (such as the critical layer mechanism of McKeon & Sharma Reference McKeon and Sharma2010). To analyse if such a rank-1 approximation is valid, Moarref et al. (Reference Moarref, Jovanović, Tropp, Sharma and McKeon2014) introduced the metric
$\hat {\boldsymbol {u}} \approx \chi _1 \sigma _1 \boldsymbol {\psi }_1$ captures the flow reasonably well (Beneddine et al. Reference Beneddine, Sipp, Arnault, Dandois and Lesshafft2016; Towne, Schmidt & Colonius Reference Towne, Schmidt and Colonius2018). This is indicative of the existence of a dominant physical mechanism that is giving rise to the resolvent amplification (such as the critical layer mechanism of McKeon & Sharma Reference McKeon and Sharma2010). To analyse if such a rank-1 approximation is valid, Moarref et al. (Reference Moarref, Jovanović, Tropp, Sharma and McKeon2014) introduced the metric ![]() $\mbox {LR} = \sigma _1^2/\sum _i \sigma _i^2$, which denotes the fraction of energy that is captured by the first resolvent mode alone. Here,
$\mbox {LR} = \sigma _1^2/\sum _i \sigma _i^2$, which denotes the fraction of energy that is captured by the first resolvent mode alone. Here, ![]() $\mbox {LR}$ is bounded between
$\mbox {LR}$ is bounded between ![]() $0$ and
$0$ and ![]() $1$, and the region of the
$1$, and the region of the ![]() $(\lambda _x,\lambda _z)$ space where
$(\lambda _x,\lambda _z)$ space where ![]() $\mbox {LR}$ is high indicates the region where a rank-1 approximation of the resolvent operator is valid. The resolvent operator remains low-rank in the wavenumber space where, from DNS and experiments of incompressible flows, we know most of the turbulent kinetic energy resides in the flow (e.g. Moarref et al. Reference Moarref, Jovanović, Tropp, Sharma and McKeon2014; Bae et al. Reference Bae, Dawson and McKeon2020b).
$\mbox {LR}$ is high indicates the region where a rank-1 approximation of the resolvent operator is valid. The resolvent operator remains low-rank in the wavenumber space where, from DNS and experiments of incompressible flows, we know most of the turbulent kinetic energy resides in the flow (e.g. Moarref et al. Reference Moarref, Jovanović, Tropp, Sharma and McKeon2014; Bae et al. Reference Bae, Dawson and McKeon2020b).
2.4. Numerical set-up for the resolvent operator
A summation-by-parts finite difference scheme with ![]() $N=401$ grid points is used to discretise the linear operator
$N=401$ grid points is used to discretise the linear operator ![]() $\boldsymbol {A}$ (2.3) in the wall-normal direction (Mattsson & Nordström Reference Mattsson and Nordström2004; Kamal et al. Reference Kamal, Rigas, Lakebrink and Colonius2020). To properly resolve the wall-normal direction, we employ a grid stretching technique that gives a grid that goes from
$\boldsymbol {A}$ (2.3) in the wall-normal direction (Mattsson & Nordström Reference Mattsson and Nordström2004; Kamal et al. Reference Kamal, Rigas, Lakebrink and Colonius2020). To properly resolve the wall-normal direction, we employ a grid stretching technique that gives a grid that goes from ![]() $0$ to at least
$0$ to at least ![]() $y_{{{max}}} = 4\delta$, with half the grid points used clustered below
$y_{{{max}}} = 4\delta$, with half the grid points used clustered below ![]() $y_{{{half}}} = 1\delta$ (Malik Reference Malik1990). The stretched grid
$y_{{{half}}} = 1\delta$ (Malik Reference Malik1990). The stretched grid ![]() $y$ in terms of equidistant points
$y$ in terms of equidistant points ![]() $0 \leqslant y' \leqslant 1$ is given as
$0 \leqslant y' \leqslant 1$ is given as ![]() $y = ay'/(b-y')$, with
$y = ay'/(b-y')$, with ![]() $a = y_{{{max}}}y_{{{half}}}/(y_{{{max}}}-2y_{{{half}}})$ and
$a = y_{{{max}}}y_{{{half}}}/(y_{{{max}}}-2y_{{{half}}})$ and ![]() $b = 1+a/y_{{{max}}}$ (e.g. Malik Reference Malik1990; Kamal et al. Reference Kamal, Rigas, Lakebrink and Colonius2020). Compressible boundary layer flows have pressure fluctuations that radiate into the free stream. These radiations are waves that have wall-normal wavelengths that are a function of their streamwise and spanwise wavenumbers
$b = 1+a/y_{{{max}}}$ (e.g. Malik Reference Malik1990; Kamal et al. Reference Kamal, Rigas, Lakebrink and Colonius2020). Compressible boundary layer flows have pressure fluctuations that radiate into the free stream. These radiations are waves that have wall-normal wavelengths that are a function of their streamwise and spanwise wavenumbers ![]() $k_x$,
$k_x$, ![]() $k_z$ and phase speed
$k_z$ and phase speed ![]() $c$. For the discussions in this work, it is important to properly resolve these pressure fluctuations. Therefore, it is important to consider their wall-normal wavelengths
$c$. For the discussions in this work, it is important to properly resolve these pressure fluctuations. Therefore, it is important to consider their wall-normal wavelengths ![]() $l$, and this
$l$, and this ![]() $l$ can be analytically approximated as a function of
$l$ can be analytically approximated as a function of ![]() $(k_x,k_z,c)$ (see (4.3) and (7.1)). Since there is a large range of
$(k_x,k_z,c)$ (see (4.3) and (7.1)). Since there is a large range of ![]() $l$ that exists in the flow, it would be challenging to resolve all of the waves using a fixed
$l$ that exists in the flow, it would be challenging to resolve all of the waves using a fixed ![]() $y_{{{max}}}$ and any reasonable number of wall-normal grid points
$y_{{{max}}}$ and any reasonable number of wall-normal grid points ![]() $N$. Therefore, for these modes, we use a
$N$. Therefore, for these modes, we use a ![]() $y_{{{max}}}$ that varies with
$y_{{{max}}}$ that varies with ![]() $(k_x,k_z,c)$ such that if
$(k_x,k_z,c)$ such that if ![]() $y_{{{max}}} = 4\delta$ is not sufficient to resolve at least
$y_{{{max}}} = 4\delta$ is not sufficient to resolve at least ![]() $3l$,
$3l$, ![]() $y_{{{max}}}$ is increased to be
$y_{{{max}}}$ is increased to be ![]() $3l$. (In Appendix B, we include a discussion on the grid convergence obtained.) To keep the forcing to the resolvent consistent across
$3l$. (In Appendix B, we include a discussion on the grid convergence obtained.) To keep the forcing to the resolvent consistent across ![]() $(k_x,k_z,c)$, all modes are forced only until
$(k_x,k_z,c)$, all modes are forced only until ![]() $3\delta$, with a weighting as introduced by Nogueira et al. (Reference Nogueira, Cavalieri, Hanifi and Henningson2020) used to set the forcing beyond
$3\delta$, with a weighting as introduced by Nogueira et al. (Reference Nogueira, Cavalieri, Hanifi and Henningson2020) used to set the forcing beyond ![]() $3\delta$ to zero.
$3\delta$ to zero.
Following Mack (Reference Mack1984) and Malik (Reference Malik1990), the boundary conditions enforced at the wall are ![]() $\hat {u}(0) = \hat {v}(0) = \hat {w}(0) = \hat {\theta }(0) = 0$. The wall-normal momentum equation at the wall is used to get the boundary condition on density, which, along with the temperature boundary condition, determines boundary condition for pressure. Since at the free stream we can assume that the equations are inviscid, Thompson boundary conditions derived from the inviscid equations are enforced here (Thompson Reference Thompson1987; Kamal et al. Reference Kamal, Rigas, Lakebrink and Colonius2020). Additionally, a damping layer is also required at the free stream to remove spurious numerical oscillations that arise from the finite difference operator (Appelö & Colonius Reference Appelö and Colonius2009) (see Appendix B for a discussion regarding this damping layer). Mean profiles that are required as input to the linear model in (2.1) are obtained from the DNS studies of Bernardini & Pirozzoli (Reference Bernardini and Pirozzoli2011), Duan et al. (Reference Duan, Choudhari and Wu2014, Reference Duan, Choudhari and Zhang2016) and Zhang et al. (Reference Zhang, Duan and Choudhari2017).
$\hat {u}(0) = \hat {v}(0) = \hat {w}(0) = \hat {\theta }(0) = 0$. The wall-normal momentum equation at the wall is used to get the boundary condition on density, which, along with the temperature boundary condition, determines boundary condition for pressure. Since at the free stream we can assume that the equations are inviscid, Thompson boundary conditions derived from the inviscid equations are enforced here (Thompson Reference Thompson1987; Kamal et al. Reference Kamal, Rigas, Lakebrink and Colonius2020). Additionally, a damping layer is also required at the free stream to remove spurious numerical oscillations that arise from the finite difference operator (Appelö & Colonius Reference Appelö and Colonius2009) (see Appendix B for a discussion regarding this damping layer). Mean profiles that are required as input to the linear model in (2.1) are obtained from the DNS studies of Bernardini & Pirozzoli (Reference Bernardini and Pirozzoli2011), Duan et al. (Reference Duan, Choudhari and Wu2014, Reference Duan, Choudhari and Zhang2016) and Zhang et al. (Reference Zhang, Duan and Choudhari2017).
There is some arbitrariness to the choice of wall-normal extent ![]() $y_{{{max}}}$ and the details of the damping layer used for the wall-normal grid. However, the results presented here are reasonably insensitive to variations in these choices. This has been discussed in detail in Appendix B.
$y_{{{max}}}$ and the details of the damping layer used for the wall-normal grid. However, the results presented here are reasonably insensitive to variations in these choices. This has been discussed in detail in Appendix B.
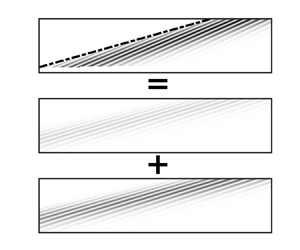
2.5. Helmholtz decomposition
As the final topic in the methods section, let us briefly look at Helmholtz decomposition, a technique that will be used frequently in this manuscript. The Helmholtz decomposition can be performed on any vector field. Consider a vector ![]() $\boldsymbol {q} = (q_x, q_y, q_z)$. The Helmholtz decomposition of
$\boldsymbol {q} = (q_x, q_y, q_z)$. The Helmholtz decomposition of ![]() $\boldsymbol {q}$ gives two components such that
$\boldsymbol {q}$ gives two components such that ![]() $\boldsymbol {q}=\boldsymbol {q}^s+\boldsymbol {q}^d$. The two components are: (i) the solenoidal component
$\boldsymbol {q}=\boldsymbol {q}^s+\boldsymbol {q}^d$. The two components are: (i) the solenoidal component ![]() $\boldsymbol {q}^s = (q^s_x, q^s_y, q^s_z)$, which is divergence-free, i.e.
$\boldsymbol {q}^s = (q^s_x, q^s_y, q^s_z)$, which is divergence-free, i.e. ![]() $\boldsymbol {\nabla }\boldsymbol {\cdot } \boldsymbol {q}^s=0$; and (ii) the dilatational component
$\boldsymbol {\nabla }\boldsymbol {\cdot } \boldsymbol {q}^s=0$; and (ii) the dilatational component ![]() $\boldsymbol {q}^d = (q^d_x, q^d_y, q^d_z)$, which is curl-free
$\boldsymbol {q}^d = (q^d_x, q^d_y, q^d_z)$, which is curl-free ![]() $\boldsymbol {\nabla }\times \boldsymbol {q}^d=0$. Helmholtz decomposition is only unique with a defined boundary condition, and the boundary conditions that are imposed here are
$\boldsymbol {\nabla }\times \boldsymbol {q}^d=0$. Helmholtz decomposition is only unique with a defined boundary condition, and the boundary conditions that are imposed here are ![]() $q^s_y(y=0)=0$ and
$q^s_y(y=0)=0$ and ![]() $q^d_x(y=0)=q^d_z(y=0)=0$ (Bhatia et al. Reference Bhatia, Norgard, Pascucci and Bremer2012). As an example, consider the velocity field
$q^d_x(y=0)=q^d_z(y=0)=0$ (Bhatia et al. Reference Bhatia, Norgard, Pascucci and Bremer2012). As an example, consider the velocity field ![]() $\boldsymbol {u}$ from an incompressible flow. Since the flow is divergence free, for this case,
$\boldsymbol {u}$ from an incompressible flow. Since the flow is divergence free, for this case, ![]() $\boldsymbol {u}=\boldsymbol {u}^s$ and
$\boldsymbol {u}=\boldsymbol {u}^s$ and ![]() $\boldsymbol {u}^d=0$.
$\boldsymbol {u}^d=0$.
In § 3.1, we will use Helmholtz decomposition of the first resolvent response mode ![]() $\boldsymbol {\psi }_1$ to find the solenoidal and dilatational components of this mode. In §§ 3.2 and 5, we will instead focus on the Helmholtz decomposition of the first resolvent forcing mode
$\boldsymbol {\psi }_1$ to find the solenoidal and dilatational components of this mode. In §§ 3.2 and 5, we will instead focus on the Helmholtz decomposition of the first resolvent forcing mode ![]() $\boldsymbol {\phi }_1$. This thereafter enables us to look at the response to the solenoidal
$\boldsymbol {\phi }_1$. This thereafter enables us to look at the response to the solenoidal ![]() $\boldsymbol {\phi }_1^s$ and the dilatational
$\boldsymbol {\phi }_1^s$ and the dilatational ![]() $\boldsymbol {\phi }_1^d$ components of this forcing mode, separately. The response to
$\boldsymbol {\phi }_1^d$ components of this forcing mode, separately. The response to ![]() $\boldsymbol {\phi }_1^s$ can be obtained as
$\boldsymbol {\phi }_1^s$ can be obtained as ![]() $\boldsymbol{\mathsf{H}}\boldsymbol {\phi }_1^s$ and to
$\boldsymbol{\mathsf{H}}\boldsymbol {\phi }_1^s$ and to ![]() $\boldsymbol {\phi }_1^d$ as
$\boldsymbol {\phi }_1^d$ as ![]() $\boldsymbol{\mathsf{H}}\boldsymbol {\phi }_1^d$.
$\boldsymbol{\mathsf{H}}\boldsymbol {\phi }_1^d$.
3. Comparing compressible and incompressible resolvent operators
In this section, we compare the resolvent norms obtained from the incompressible and the compressible resolvent operators. This comparison is similar to that by Bae et al. (Reference Bae, Dawson and McKeon2020b), however, in addition to the low-rank map that was compared by Bae et al. (Reference Bae, Dawson and McKeon2020b), here, we also look at the leading resolvent norm, which is important for the discussions. In figure 1, an ![]() $Re_\tau = 450$ incompressible resolvent operator is shown in panels (a,c) and an
$Re_\tau = 450$ incompressible resolvent operator is shown in panels (a,c) and an ![]() $Ma=4$ compressible resolvent operator at a comparable Reynolds number (
$Ma=4$ compressible resolvent operator at a comparable Reynolds number (![]() $Re_\tau = 400$) is in panels (b,d). In figure 1(a,b), the leading resolvent norm
$Re_\tau = 400$) is in panels (b,d). In figure 1(a,b), the leading resolvent norm ![]() $\sigma _1$ in (2.8) is shown with respect to the streamwise and spanwise wavelengths (
$\sigma _1$ in (2.8) is shown with respect to the streamwise and spanwise wavelengths (![]() $\lambda _x$,
$\lambda _x$, ![]() $\lambda _z$), for a fixed value of
$\lambda _z$), for a fixed value of ![]() $c=\bar {U}(y^+\approx 15)$. The colour-scale used in the figure is logarithmic. While the full Chu norm (2.4) is used for the compressible case, the kinetic energy norm is used for the incompressible case and this difference does not significantly impact the discussions here. In figure 1(
$c=\bar {U}(y^+\approx 15)$. The colour-scale used in the figure is logarithmic. While the full Chu norm (2.4) is used for the compressible case, the kinetic energy norm is used for the incompressible case and this difference does not significantly impact the discussions here. In figure 1(![]() $a$), the grey contour line indicates a third of the maximum energy of the incompressible case. The same contour line (computed from the incompressible case) is also shown in figure 1(
$a$), the grey contour line indicates a third of the maximum energy of the incompressible case. The same contour line (computed from the incompressible case) is also shown in figure 1(![]() $b$). The green contours at the top right-hand corner for the compressible case in figure 1(
$b$). The green contours at the top right-hand corner for the compressible case in figure 1(![]() $b$) indicates the region of the wavenumber space that is unstable. In figure 1(c,d), we compare the low-rank maps
$b$) indicates the region of the wavenumber space that is unstable. In figure 1(c,d), we compare the low-rank maps ![]() $\mbox {LR}=\sigma _1^2/(\sum _i \sigma _i^2)$ (see § 2.3) that shows the fraction of energy captured by the leading resolvent mode at each (
$\mbox {LR}=\sigma _1^2/(\sum _i \sigma _i^2)$ (see § 2.3) that shows the fraction of energy captured by the leading resolvent mode at each (![]() $\lambda _x,\lambda _y,c$).
$\lambda _x,\lambda _y,c$).

Figure 1. (a,b) Leading resolvent gain ![]() $\sigma _1$ as well as (c,d) fraction of energy captured by the leading resolvent mode
$\sigma _1$ as well as (c,d) fraction of energy captured by the leading resolvent mode ![]() $\mbox {LR}=\sigma _1^2/(\sum _i\sigma _i^2)$ as a function of the streamwise and spanwise wavelengths (
$\mbox {LR}=\sigma _1^2/(\sum _i\sigma _i^2)$ as a function of the streamwise and spanwise wavelengths (![]() $\lambda _x$,
$\lambda _x$,![]() $\lambda _z$) at a fixed phase speed
$\lambda _z$) at a fixed phase speed ![]() $c \approx \bar {U}(y^+ = 15)$. (a,c) An incompressible boundary layer with
$c \approx \bar {U}(y^+ = 15)$. (a,c) An incompressible boundary layer with ![]() $Re_\tau = 450$ and (b,d) a compressible boundary layer with
$Re_\tau = 450$ and (b,d) a compressible boundary layer with ![]() $Ma=4$ and
$Ma=4$ and ![]() $Re_\tau = 400$ over an adiabatic wall. The black dashed line in panels (b,d) indicates the relative Mach number equal to unity. The green contours at the top right-hand corner for the compressible case indicates the region of the wavenumber space that is unstable. The grey contour line in panel (a) indicates a third of the maximum energy in the incompressible case, and the same contour (computed from the incompressible case) also appears in panel (b) for comparison. The diamond (
$Re_\tau = 400$ over an adiabatic wall. The black dashed line in panels (b,d) indicates the relative Mach number equal to unity. The green contours at the top right-hand corner for the compressible case indicates the region of the wavenumber space that is unstable. The grey contour line in panel (a) indicates a third of the maximum energy in the incompressible case, and the same contour (computed from the incompressible case) also appears in panel (b) for comparison. The diamond (![]() $\blacklozenge$) in panels (a,b) and the square (
$\blacklozenge$) in panels (a,b) and the square (![]() $\blacksquare$) and circle (
$\blacksquare$) and circle (![]() $\bullet$) in panel (b) indicate the modes that are discussed in later figures.
$\bullet$) in panel (b) indicate the modes that are discussed in later figures.
The black dashed line in figure 1(![]() $b$) indicates the region where the free stream relative Mach number is equal to unity
$b$) indicates the region where the free stream relative Mach number is equal to unity ![]() $\overline {Ma}(y \to \infty )=1$, where
$\overline {Ma}(y \to \infty )=1$, where ![]() $\overline {Ma}(y)$ is a local Mach number at a particular wall height
$\overline {Ma}(y)$ is a local Mach number at a particular wall height ![]() $y$ defined for each (
$y$ defined for each (![]() $\lambda _x,\lambda _y,c$) as
$\lambda _x,\lambda _y,c$) as
The relative Mach number ![]() $\overline {Ma}(y)$ is the projection of the phase speed relative to the mean flow
$\overline {Ma}(y)$ is the projection of the phase speed relative to the mean flow ![]() $(\bar {U}(y)-c)$ in the direction of the streamwise wavenumber
$(\bar {U}(y)-c)$ in the direction of the streamwise wavenumber ![]() $k_x/k$ (Mack Reference Mack1984). For the flows considered here, at a particular (
$k_x/k$ (Mack Reference Mack1984). For the flows considered here, at a particular (![]() $\lambda _x,\lambda _y,c$), the maximum value of
$\lambda _x,\lambda _y,c$), the maximum value of ![]() $\overline {Ma}(y)$ is
$\overline {Ma}(y)$ is ![]() $\overline {Ma}(\infty )$. Above the black dashed line in figure 1(
$\overline {Ma}(\infty )$. Above the black dashed line in figure 1(![]() $b$),
$b$), ![]() $\overline {Ma}(\infty )>1$. Below this line,
$\overline {Ma}(\infty )>1$. Below this line, ![]() $\overline {Ma}(\infty )<1$. In § 4, we will see that the supersonic resolvent modes, which is the main subject of the discussions in the current work, can only exist when
$\overline {Ma}(\infty )<1$. In § 4, we will see that the supersonic resolvent modes, which is the main subject of the discussions in the current work, can only exist when ![]() $\overline {Ma}(\infty ) \geqslant 1$ and therefore can only exist above the black dashed line in figure 1(
$\overline {Ma}(\infty ) \geqslant 1$ and therefore can only exist above the black dashed line in figure 1(![]() $b$) (Bae et al. Reference Bae, Dawson and McKeon2020b).
$b$) (Bae et al. Reference Bae, Dawson and McKeon2020b).
Before considering these Mach waves in detail, let us briefly consider the region below the black dashed line, i.e. the subsonic modes. We note that the most amplified subsonic modes are linearly stable, i.e. do not fall within the green contour lines in figure 1(![]() $b$). To compare these subsonic modes with the incompressible case, between figures 1(
$b$). To compare these subsonic modes with the incompressible case, between figures 1(![]() $a$) and 1(
$a$) and 1(![]() $b$), we compare the regions of high amplification and between figures 1(
$b$), we compare the regions of high amplification and between figures 1(![]() $c$) and 1(
$c$) and 1(![]() $d$), we compare the regions where the resolvent operator is low-rank. Below the black dashed line, the trends of the incompressible and the compressible resolvent operators are similar. This suggests that within this region of the wavenumber space of the compressible flow, the mechanisms that are active are the same as in incompressible flows.
$d$), we compare the regions where the resolvent operator is low-rank. Below the black dashed line, the trends of the incompressible and the compressible resolvent operators are similar. This suggests that within this region of the wavenumber space of the compressible flow, the mechanisms that are active are the same as in incompressible flows.
For further comparison, consider the structure with ![]() $\lambda _x=5$,
$\lambda _x=5$, ![]() $\lambda _z=0.5$ and
$\lambda _z=0.5$ and ![]() $c=\bar {U}(y^+\approx 15)$ (indicated by diamonds
$c=\bar {U}(y^+\approx 15)$ (indicated by diamonds ![]() $({\scriptsize {\blacklozenge }})$ in figure 1a,b). The leading resolvent mode for the structure is shown in figure 2. The three components of velocity are compared in panels (a–c), with the solid coloured lines representing the compressible mode and the black dashed line representing the incompressible mode. For both compressible and incompressible cases, the modes are localised and reside within the boundary layer (the
$({\scriptsize {\blacklozenge }})$ in figure 1a,b). The leading resolvent mode for the structure is shown in figure 2. The three components of velocity are compared in panels (a–c), with the solid coloured lines representing the compressible mode and the black dashed line representing the incompressible mode. For both compressible and incompressible cases, the modes are localised and reside within the boundary layer (the ![]() $y$-axis terminates at
$y$-axis terminates at ![]() $0.5\delta$), and there are no significant differences between the incompressible and compressible structures. This similarity between the compressible and incompressible resolvent operators was explored by Bae et al. (Reference Bae, Dawson and McKeon2020b), where they showed that when the compressible modes are scaled using the semi-local scaling of compressible flows (Trettel & Larsson Reference Trettel and Larsson2016), they collapse well onto the modes from the incompressible flow. Of course, in the case of the compressible flow, there are the additional components of temperature and density, and these are shown in figures 2(
$0.5\delta$), and there are no significant differences between the incompressible and compressible structures. This similarity between the compressible and incompressible resolvent operators was explored by Bae et al. (Reference Bae, Dawson and McKeon2020b), where they showed that when the compressible modes are scaled using the semi-local scaling of compressible flows (Trettel & Larsson Reference Trettel and Larsson2016), they collapse well onto the modes from the incompressible flow. Of course, in the case of the compressible flow, there are the additional components of temperature and density, and these are shown in figures 2(![]() $d$) and 2(
$d$) and 2(![]() $e$). Temperature and density also show localised profiles within the boundary layer.
$e$). Temperature and density also show localised profiles within the boundary layer.

Figure 2. Leading resolvent response for the modes indicated by the diamonds (![]() $\blacklozenge$) in figure 1(a,b). The mode corresponds to
$\blacklozenge$) in figure 1(a,b). The mode corresponds to ![]() $\lambda _x=5$,
$\lambda _x=5$, ![]() $\lambda _z=0.5$ and
$\lambda _z=0.5$ and ![]() $c=\bar {U}(y^+\approx 15)$. The wall-normal profile of the (a) streamwise, (b) wall-normal and (c) spanwise velocities. The solid lines in panels (a–c) represent the mode from a compressible boundary layer with
$c=\bar {U}(y^+\approx 15)$. The wall-normal profile of the (a) streamwise, (b) wall-normal and (c) spanwise velocities. The solid lines in panels (a–c) represent the mode from a compressible boundary layer with ![]() $Ma=4$ and
$Ma=4$ and ![]() $Re_\tau = 400$ and the black dashed lines represent the mode from an incompressible boundary layer with
$Re_\tau = 400$ and the black dashed lines represent the mode from an incompressible boundary layer with ![]() $Re_\tau = 450$. The profiles of (d) density and (e) temperature for the compressible case.
$Re_\tau = 450$. The profiles of (d) density and (e) temperature for the compressible case.
3.1. Helmholtz decomposition of the resolvent response
We can attempt to isolate these incompressible-like subsonic modes from the compressible effects in the flow by doing a Helmholtz decomposition of the velocity components ![]() $\hat {\boldsymbol {u}} = (\hat {u}, \hat {v}, \hat {w})$ of the leading resolvent mode
$\hat {\boldsymbol {u}} = (\hat {u}, \hat {v}, \hat {w})$ of the leading resolvent mode ![]() $\boldsymbol {\psi }_1$. As described in § 2.5, the Helmholtz decomposition gives: (i) a solenoidal component
$\boldsymbol {\psi }_1$. As described in § 2.5, the Helmholtz decomposition gives: (i) a solenoidal component ![]() $\hat {\boldsymbol {u}}^s$ and (ii) a dilatational component
$\hat {\boldsymbol {u}}^s$ and (ii) a dilatational component ![]() $\hat {\boldsymbol {u}}^d$ (in an incompressible flow,
$\hat {\boldsymbol {u}}^d$ (in an incompressible flow, ![]() $\hat {\boldsymbol {u}}^d=0$). In figure 3, the full kinetic energy in figure 3(
$\hat {\boldsymbol {u}}^d=0$). In figure 3, the full kinetic energy in figure 3(![]() $a$) is compared with the kinetic energy of
$a$) is compared with the kinetic energy of ![]() $\hat {\boldsymbol {u}}^s$ in figure 3(
$\hat {\boldsymbol {u}}^s$ in figure 3(![]() $b$) and of
$b$) and of ![]() $\hat {\boldsymbol {u}}^d$ in figure 3(
$\hat {\boldsymbol {u}}^d$ in figure 3(![]() $c$). The grey contour in figure 3(
$c$). The grey contour in figure 3(![]() $b$) is the same as that shown in figure 1(
$b$) is the same as that shown in figure 1(![]() $a$) and indicates the region of the wavenumber space where the incompressible resolvent is reasonably amplified.
$a$) and indicates the region of the wavenumber space where the incompressible resolvent is reasonably amplified.

Figure 3. Kinetic energy of (a) the leading resolvent response ![]() $\boldsymbol {\psi }_1$ and of (b) the solenoidal and (c) the dilatational component of the velocity from
$\boldsymbol {\psi }_1$ and of (b) the solenoidal and (c) the dilatational component of the velocity from ![]() $\boldsymbol {\psi }_1$ as a function of the streamwise and spanwise wavelengths (
$\boldsymbol {\psi }_1$ as a function of the streamwise and spanwise wavelengths (![]() $\lambda _x, \lambda _z$) and a fixed phase speed
$\lambda _x, \lambda _z$) and a fixed phase speed ![]() $c = \bar {U}(y^+\approx 15)$. The grey contour line in panel (b) is the same as that shown in figure 1(
$c = \bar {U}(y^+\approx 15)$. The grey contour line in panel (b) is the same as that shown in figure 1(![]() $a$) and shows the kinetic energy for the incompressible case at a third of the maximum.
$a$) and shows the kinetic energy for the incompressible case at a third of the maximum.
The solenoidal response in figure 3(![]() $b$) looks similar to the response of the incompressible flow in figure 1(
$b$) looks similar to the response of the incompressible flow in figure 1(![]() $a$). Subsonic modes, i.e. the modes below the
$a$). Subsonic modes, i.e. the modes below the ![]() $\overline {Ma}(\infty )=1$ line, have solenoidal velocity, consistent with observations from DNS (Yu, Xu & Pirozzoli Reference Yu, Xu and Pirozzoli2019). Notably, in figure 3(
$\overline {Ma}(\infty )=1$ line, have solenoidal velocity, consistent with observations from DNS (Yu, Xu & Pirozzoli Reference Yu, Xu and Pirozzoli2019). Notably, in figure 3(![]() $b$),
$b$), ![]() $\hat {\boldsymbol {u}}^s$ captures some of the energy in modes above the
$\hat {\boldsymbol {u}}^s$ captures some of the energy in modes above the ![]() $\overline {Ma}(\infty )=1$ line where supersonic mechanisms are active. The grey contour line indicates that this occurs in the region where subsonic mechanisms are also active. These modes therefore have both the subsonic as well as supersonic mechanisms active. The co-existence of two amplification mechanisms provides an explanation for the observed decrease in the low-rank behaviour of these modes in figure 1(
$\overline {Ma}(\infty )=1$ line where supersonic mechanisms are active. The grey contour line indicates that this occurs in the region where subsonic mechanisms are also active. These modes therefore have both the subsonic as well as supersonic mechanisms active. The co-existence of two amplification mechanisms provides an explanation for the observed decrease in the low-rank behaviour of these modes in figure 1(![]() $d$). However, the most interesting consequence of this co-existence of mechanisms is that it provides an additional route for amplifying the supersonic resolvent modes. This amplification route will be explained in § 5.2 and its potential importance to the real flow will be discussed in § 7.
$d$). However, the most interesting consequence of this co-existence of mechanisms is that it provides an additional route for amplifying the supersonic resolvent modes. This amplification route will be explained in § 5.2 and its potential importance to the real flow will be discussed in § 7.
3.2. Forcing to the subsonic modes: Helmholtz decomposition of resolvent forcing
In this section, we take the comparison between the compressible and incompressible operators one step further. For incompressible resolvent operators, if we consider Helmholtz decomposition of the forcing to the momentum equations ![]() $\hat {\boldsymbol {f}}_{\!\!\boldsymbol{u}}$, it is known that only the solenoidal component of the forcing
$\hat {\boldsymbol {f}}_{\!\!\boldsymbol{u}}$, it is known that only the solenoidal component of the forcing ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ has any active influence in amplifying resolvent modes (Rosenberg & McKeon Reference Rosenberg and McKeon2019; Morra et al. Reference Morra, Nogueira, Cavalieri and Henningson2021). Although
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ has any active influence in amplifying resolvent modes (Rosenberg & McKeon Reference Rosenberg and McKeon2019; Morra et al. Reference Morra, Nogueira, Cavalieri and Henningson2021). Although ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d$ is not zero in the real flow (i.e. the divergence of the full forcing
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d$ is not zero in the real flow (i.e. the divergence of the full forcing ![]() $\boldsymbol {\nabla }\boldsymbol {\cdot } \hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}} \neq 0$), this component cannot directly excite a response in velocity (Rosenberg & McKeon Reference Rosenberg and McKeon2019; Morra et al. Reference Morra, Nogueira, Cavalieri and Henningson2021). Here, we ask if this property of incompressible flows carries over to the subsonic modes of compressible flows. We will take
$\boldsymbol {\nabla }\boldsymbol {\cdot } \hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}} \neq 0$), this component cannot directly excite a response in velocity (Rosenberg & McKeon Reference Rosenberg and McKeon2019; Morra et al. Reference Morra, Nogueira, Cavalieri and Henningson2021). Here, we ask if this property of incompressible flows carries over to the subsonic modes of compressible flows. We will take ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}$ from the leading resolvent forcing mode
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}$ from the leading resolvent forcing mode ![]() $\boldsymbol {\phi }_1$ (the suboptimal modes are considered in Appendix A). A Helmholtz decomposition of this resolvent forcing mode gives the solenoidal component
$\boldsymbol {\phi }_1$ (the suboptimal modes are considered in Appendix A). A Helmholtz decomposition of this resolvent forcing mode gives the solenoidal component ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s = (\,\hat{f}^s_u, \hat {f}^s_v, \hat {f}^s_w)$ and the dilatational component
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s = (\,\hat{f}^s_u, \hat {f}^s_v, \hat {f}^s_w)$ and the dilatational component ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d= (\,\hat {f}^d_u, \hat {f}^d_v, \hat {f}^d_w)$. We will consider the response to the solenoidal component alone through
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d= (\,\hat {f}^d_u, \hat {f}^d_v, \hat {f}^d_w)$. We will consider the response to the solenoidal component alone through ![]() $\hat {\boldsymbol {f}}_{\!\!1}=(\,\hat {f}^s_u, \hat {f}^s_v, \hat {f}^s_w, 0, 0)$, and the response to the dilatational component along with the forcing to the density
$\hat {\boldsymbol {f}}_{\!\!1}=(\,\hat {f}^s_u, \hat {f}^s_v, \hat {f}^s_w, 0, 0)$, and the response to the dilatational component along with the forcing to the density ![]() $\hat {f}_\rho$ and temperature
$\hat {f}_\rho$ and temperature ![]() $\hat {f}_\theta$ equations through
$\hat {f}_\theta$ equations through ![]() $\hat {\boldsymbol {f}}_{\!\!2}=(\,\hat {f}^d_u, \hat {f}^d_v, \hat {f}^d_w, \hat {f}_\rho, \hat {f}_\theta )$. The response to
$\hat {\boldsymbol {f}}_{\!\!2}=(\,\hat {f}^d_u, \hat {f}^d_v, \hat {f}^d_w, \hat {f}_\rho, \hat {f}_\theta )$. The response to ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ is obtained as
$\hat {\boldsymbol {f}}_{\!\!1}$ is obtained as ![]() $\boldsymbol{\mathsf{H}} \hat {\boldsymbol {f}}_{\!\!1}$ and to
$\boldsymbol{\mathsf{H}} \hat {\boldsymbol {f}}_{\!\!1}$ and to ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ as
$\hat {\boldsymbol {f}}_{\!\!2}$ as ![]() $\boldsymbol{\mathsf{H}} \hat {\boldsymbol {f}}_{\!\!2}$.
$\boldsymbol{\mathsf{H}} \hat {\boldsymbol {f}}_{\!\!2}$.
In figures 4(![]() $a$)–4(
$a$)–4(![]() $e$), for the same structure as in figure 2, the five components of the leading resolvent response mode in black is compared with the response to
$e$), for the same structure as in figure 2, the five components of the leading resolvent response mode in black is compared with the response to ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ in blue and to
$\hat {\boldsymbol {f}}_{\!\!1}$ in blue and to ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ in red. We see that the response is almost entirely captured by the solenoidal component of the forcing. To see that this is more generally true, in figure 4(f–j), the same comparison is shown for an
$\hat {\boldsymbol {f}}_{\!\!2}$ in red. We see that the response is almost entirely captured by the solenoidal component of the forcing. To see that this is more generally true, in figure 4(f–j), the same comparison is shown for an ![]() $Re_\tau =450$ boundary layer with a higher Mach number of
$Re_\tau =450$ boundary layer with a higher Mach number of ![]() $Ma=6$, over a cooled wall with wall-cooling ratio
$Ma=6$, over a cooled wall with wall-cooling ratio ![]() $\varTheta _w/\varTheta _{ad}=0.25$. Here again, we see that the solenoidal component of the forcing captures a majority of the response. Therefore, like in the incompressible case, the subsonic resolvent modes are actively forced only by the solenoidal component of the forcing; and this is true both for flows over adiabatic and cooled walls.
$\varTheta _w/\varTheta _{ad}=0.25$. Here again, we see that the solenoidal component of the forcing captures a majority of the response. Therefore, like in the incompressible case, the subsonic resolvent modes are actively forced only by the solenoidal component of the forcing; and this is true both for flows over adiabatic and cooled walls.

Figure 4. Response of the resolvent operator to the full leading resolvent forcing mode ![]() $\boldsymbol {\phi }_1$ (black) as well as the response to the two components of the forcing
$\boldsymbol {\phi }_1$ (black) as well as the response to the two components of the forcing ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ (blue) and
$\hat {\boldsymbol {f}}_{\!\!1}$ (blue) and ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ (red). Subsonic modes with
$\hat {\boldsymbol {f}}_{\!\!2}$ (red). Subsonic modes with ![]() $(\lambda _x,\lambda _z,c)=(5,0.5,\bar {U}(y^+\approx 15))$ for two compressible boundary layers: (a–e)
$(\lambda _x,\lambda _z,c)=(5,0.5,\bar {U}(y^+\approx 15))$ for two compressible boundary layers: (a–e) ![]() $Ma=4$,
$Ma=4$, ![]() $Re_\tau =400$ over an adiabatic wall (the mode indicated by the (
$Re_\tau =400$ over an adiabatic wall (the mode indicated by the (![]() $\blacklozenge$) in figure 1
$\blacklozenge$) in figure 1![]() $b$) and (f–j)
$b$) and (f–j) ![]() $Ma=6$,
$Ma=6$, ![]() $Re_\tau =450$ with
$Re_\tau =450$ with ![]() $\varTheta _w/\varTheta _{ad}=0.25$. (a,f) Streamwise, (b,g) wall-normal and (c,h) spanwise velocities as well as the (d,i) density and (e,j) temperature.
$\varTheta _w/\varTheta _{ad}=0.25$. (a,f) Streamwise, (b,g) wall-normal and (c,h) spanwise velocities as well as the (d,i) density and (e,j) temperature.
4. Mach wave radiation from the resolvent operator
We will now focus on the region of the wavenumber space that falls above the ![]() $\overline {Ma}(\infty )=1$ line in figure 1(
$\overline {Ma}(\infty )=1$ line in figure 1(![]() $b$). The amplification in this region is largely due to supersonic resolvent modes. In this section, the connection between these resolvent modes and the relative Mach number defined in (3.1) is first established. We will then focus on the contribution of these modes to the free stream fluctuations of the boundary layer in § 4.1 and the effect of viscosity on these modes in § 4.2. Thereafter, from § 5 onward, we will concentrate on the forcing mechanisms that amplify these modes.
$b$). The amplification in this region is largely due to supersonic resolvent modes. In this section, the connection between these resolvent modes and the relative Mach number defined in (3.1) is first established. We will then focus on the contribution of these modes to the free stream fluctuations of the boundary layer in § 4.1 and the effect of viscosity on these modes in § 4.2. Thereafter, from § 5 onward, we will concentrate on the forcing mechanisms that amplify these modes.
These supersonic modes above the ![]() $\overline {Ma}(\infty )=1$ line are pressure fluctuations that radiate into the free stream and they occur due to the Mach wave radiation mechanism as explained by Mack (Reference Mack1984). We will briefly follow the derivation of the Mach wave radiation by Mack (Reference Mack1984), with the difference of keeping the nonlinear terms as a forcing
$\overline {Ma}(\infty )=1$ line are pressure fluctuations that radiate into the free stream and they occur due to the Mach wave radiation mechanism as explained by Mack (Reference Mack1984). We will briefly follow the derivation of the Mach wave radiation by Mack (Reference Mack1984), with the difference of keeping the nonlinear terms as a forcing ![]() $\boldsymbol {f}$, rather than setting them to zero as done by Mack (Reference Mack1984). This will enable us to later isolate the two routes by which the Mach waves can cause high resolvent amplification: (i) the direct route (§ 5.1) and (ii) the indirect route (§ 5.2).
$\boldsymbol {f}$, rather than setting them to zero as done by Mack (Reference Mack1984). This will enable us to later isolate the two routes by which the Mach waves can cause high resolvent amplification: (i) the direct route (§ 5.1) and (ii) the indirect route (§ 5.2).
As done by Mack (Reference Mack1984), let us for simplicity consider the inviscid form of the governing equations Fourier transformed in ![]() $(x,y)$. As in (2.1), the nonlinear terms in the equations and any external disturbances to the flow are denoted as the unknown forcing
$(x,y)$. As in (2.1), the nonlinear terms in the equations and any external disturbances to the flow are denoted as the unknown forcing ![]() $\hat {\boldsymbol {f}}$, therefore giving
$\hat {\boldsymbol {f}}$, therefore giving
Here, ![]() $\boldsymbol {u}=(u_1,u_2,u_3)=(u,v,w)$ and we have used
$\boldsymbol {u}=(u_1,u_2,u_3)=(u,v,w)$ and we have used ![]() $\bar {\rho }\bar {\varTheta }=1$ to write
$\bar {\rho }\bar {\varTheta }=1$ to write ![]() $- \bar {\rho } \partial \bar {\varTheta }/\partial y = \bar {\varTheta } \partial \bar {\rho }/\partial y$. Pressure fluctuation
$- \bar {\rho } \partial \bar {\varTheta }/\partial y = \bar {\varTheta } \partial \bar {\rho }/\partial y$. Pressure fluctuation ![]() $\hat {p}$ can be written using the linearised equation of state as
$\hat {p}$ can be written using the linearised equation of state as ![]() $\hat {p} = \bar {\varTheta } \hat {\rho } + \bar {\rho } \hat {\theta }$. Using (4.1b) and (4.1c), and computing
$\hat {p} = \bar {\varTheta } \hat {\rho } + \bar {\rho } \hat {\theta }$. Using (4.1b) and (4.1c), and computing ![]() $\boldsymbol {\nabla }\boldsymbol {\cdot } \boldsymbol {u}$ from (4.1a), we rewrite this equation for
$\boldsymbol {\nabla }\boldsymbol {\cdot } \boldsymbol {u}$ from (4.1a), we rewrite this equation for ![]() $\hat {p}$ as
$\hat {p}$ as
 \begin{align} -\frac{\partial^2 \hat{p}}{\partial y^2} + k^2(1-\overline{Ma}^2)\hat{p} & = {\rm i}\gamma Ma^2 \left[2 k_x \bar{\rho} \frac{{\rm d}\bar{U}}{{\rm d} y} + \frac{{\rm d}\bar{\rho}}{{\rm d}y} (k_x \bar{U}-\omega) \right] \hat{v} \nonumber\\ &\quad - \gamma Ma^2 \boldsymbol{\nabla}\boldsymbol{\cdot} \hat{\boldsymbol{f}}_{\!\!\boldsymbol{u}} + {\rm i}Ma^2 \bar{\rho} (k_x \bar{U}-\omega) \left( \hat{f}_\theta + \hat{f}_\rho \right) . \end{align}
\begin{align} -\frac{\partial^2 \hat{p}}{\partial y^2} + k^2(1-\overline{Ma}^2)\hat{p} & = {\rm i}\gamma Ma^2 \left[2 k_x \bar{\rho} \frac{{\rm d}\bar{U}}{{\rm d} y} + \frac{{\rm d}\bar{\rho}}{{\rm d}y} (k_x \bar{U}-\omega) \right] \hat{v} \nonumber\\ &\quad - \gamma Ma^2 \boldsymbol{\nabla}\boldsymbol{\cdot} \hat{\boldsymbol{f}}_{\!\!\boldsymbol{u}} + {\rm i}Ma^2 \bar{\rho} (k_x \bar{U}-\omega) \left( \hat{f}_\theta + \hat{f}_\rho \right) . \end{align}
Here, ![]() $k^2=k_x^2+k_z^2$ and
$k^2=k_x^2+k_z^2$ and ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}} = (\,\hat {f}_u, \hat {f}_v, \hat {f}_w)$. (Please see Mack (Reference Mack1984) for a more complete derivation.) Note that in (4.2),
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}} = (\,\hat {f}_u, \hat {f}_v, \hat {f}_w)$. (Please see Mack (Reference Mack1984) for a more complete derivation.) Note that in (4.2), ![]() $Ma$ denotes the free stream Mach number and
$Ma$ denotes the free stream Mach number and ![]() $\overline {Ma}$ denotes the relative Mach number
$\overline {Ma}$ denotes the relative Mach number ![]() $\overline {Ma}(y)$ defined in (3.1). Following Mack (Reference Mack1984), let us consider the unforced equation within the free stream. In this case, the first term on the right-hand side of (4.2) is zero since the terms within the square brackets are zero in the free stream. The second and third terms are zero since we are considering the unforced equations. Therefore, the right-hand side of (4.2) goes to zero. For this equation, when
$\overline {Ma}(y)$ defined in (3.1). Following Mack (Reference Mack1984), let us consider the unforced equation within the free stream. In this case, the first term on the right-hand side of (4.2) is zero since the terms within the square brackets are zero in the free stream. The second and third terms are zero since we are considering the unforced equations. Therefore, the right-hand side of (4.2) goes to zero. For this equation, when ![]() $\overline {Ma}(y)>1$, we obtain a wave equation with solutions of the form
$\overline {Ma}(y)>1$, we obtain a wave equation with solutions of the form
These are the Mach waves of the flow derived by Mack (Reference Mack1984) as solutions of the inviscid unforced free stream equations when ![]() $\overline {Ma}(\infty )>1$. From (4.3), we get both upstream and downstream inclining waves, but only the downstream inclining waves satisfy the boundary conditions and therefore become valid solutions (Mack Reference Mack1984).
$\overline {Ma}(\infty )>1$. From (4.3), we get both upstream and downstream inclining waves, but only the downstream inclining waves satisfy the boundary conditions and therefore become valid solutions (Mack Reference Mack1984).
Going back to figure 1(![]() $b$), the supersonic resolvent modes are amplified only when
$b$), the supersonic resolvent modes are amplified only when ![]() $\overline {Ma}(\infty )\geqslant 1$ suggesting that these resolvent modes are Mach waves (Bae et al. Reference Bae, Dawson and McKeon2020b). To show that this is indeed the case, let us compare resolvent modes with (4.3). We pick the
$\overline {Ma}(\infty )\geqslant 1$ suggesting that these resolvent modes are Mach waves (Bae et al. Reference Bae, Dawson and McKeon2020b). To show that this is indeed the case, let us compare resolvent modes with (4.3). We pick the ![]() $(\lambda _x,\lambda _y)$ marked by a square (
$(\lambda _x,\lambda _y)$ marked by a square (![]() $\blacksquare$) in figure 1(
$\blacksquare$) in figure 1(![]() $b$) and the pressure
$b$) and the pressure ![]() $(\psi _1)_p$ from the leading resolvent mode of this structure is computed using the density
$(\psi _1)_p$ from the leading resolvent mode of this structure is computed using the density ![]() $(\psi _1)_\rho$ and the temperature
$(\psi _1)_\rho$ and the temperature ![]() $(\psi _1)_\theta$ components as
$(\psi _1)_\theta$ components as ![]() $(\psi _1)_p = \bar {\varTheta }(\psi _1)_\rho + \bar {\rho }(\psi _1)_\theta$. The real part of
$(\psi _1)_p = \bar {\varTheta }(\psi _1)_\rho + \bar {\rho }(\psi _1)_\theta$. The real part of ![]() $(\psi _1)_p$ is shown as the red solid line in figure 5(
$(\psi _1)_p$ is shown as the red solid line in figure 5(![]() $a$). In the same figure, the real part of the Mach wave obtained using (4.3) is also shown in black (the amplitude and phase of the Mach wave is fixed to be the same as the resolvent mode at some arbitrary wall height, here
$a$). In the same figure, the real part of the Mach wave obtained using (4.3) is also shown in black (the amplitude and phase of the Mach wave is fixed to be the same as the resolvent mode at some arbitrary wall height, here ![]() $y=5$). To further compare, figure 5(
$y=5$). To further compare, figure 5(![]() $b$) shows the negative (blue) and the positive (red) pressure fluctuations from the resolvent mode
$b$) shows the negative (blue) and the positive (red) pressure fluctuations from the resolvent mode ![]() $(\psi _1)_p$ in a streamwise wall-normal (
$(\psi _1)_p$ in a streamwise wall-normal (![]() $x$–
$x$–![]() $y$) plane at a fixed spanwise location (here
$y$) plane at a fixed spanwise location (here ![]() $z=0$). The black line-contours represent the Mach wave from (4.3) (the contours represent
$z=0$). The black line-contours represent the Mach wave from (4.3) (the contours represent ![]() $\pm 0.5$ of the maximum value). From these figures, first we observe that, far from the wall, these resolvent modes are outgoing waves that radiate into the free stream. Additionally, we also note that, within the free stream, the resolvent modes closely follow the behaviour of the inviscid Mach wave. This further suggests that the Mach waves are responsible for the high resolvent amplification in the wavenumber region where the free stream relative Mach number is greater than
$\pm 0.5$ of the maximum value). From these figures, first we observe that, far from the wall, these resolvent modes are outgoing waves that radiate into the free stream. Additionally, we also note that, within the free stream, the resolvent modes closely follow the behaviour of the inviscid Mach wave. This further suggests that the Mach waves are responsible for the high resolvent amplification in the wavenumber region where the free stream relative Mach number is greater than ![]() $1$, i.e.
$1$, i.e. ![]() $\overline {Ma}(\infty )\geqslant 1$. For the sake of completeness, the velocity, density and temperature components of this mode are also shown in figure 5(
$\overline {Ma}(\infty )\geqslant 1$. For the sake of completeness, the velocity, density and temperature components of this mode are also shown in figure 5(![]() $c$), and we see that these components also oscillate in the free stream.
$c$), and we see that these components also oscillate in the free stream.

Figure 5. Leading resolvent response for the mode indicated by the square (![]() $\blacksquare$) in figure 1(b). The mode corresponds to
$\blacksquare$) in figure 1(b). The mode corresponds to ![]() $\lambda _x=5$,
$\lambda _x=5$, ![]() $\lambda _z=3.5$ and
$\lambda _z=3.5$ and ![]() $c=\bar {U}(y^+\approx 15)$ for a compressible boundary layer with
$c=\bar {U}(y^+\approx 15)$ for a compressible boundary layer with ![]() $Ma=4$ and
$Ma=4$ and ![]() $Re_\tau = 400$ over an adiabatic wall. (a) Wall-normal profile of the real part of the pressure from the resolvent mode (red) compared with the pressure fluctuations from the inviscid Mach wave (black dashed) given by (4.3). (b) Pressure fluctuations in an
$Re_\tau = 400$ over an adiabatic wall. (a) Wall-normal profile of the real part of the pressure from the resolvent mode (red) compared with the pressure fluctuations from the inviscid Mach wave (black dashed) given by (4.3). (b) Pressure fluctuations in an ![]() $x\unicode{x2013}y$ plane with red (positive) and blue (negative) contours showing the real part of the pressure fluctuation from the resolvent mode and the black contour lines indicating the pressure fluctuations from the inviscid Mach wave at
$x\unicode{x2013}y$ plane with red (positive) and blue (negative) contours showing the real part of the pressure fluctuation from the resolvent mode and the black contour lines indicating the pressure fluctuations from the inviscid Mach wave at ![]() $\pm 0.5$ of the maximum. (c) Wall-normal profile of the streamwise (blue), wall-normal (green) and spanwise (orange) velocities, as well as the density (purple) and temperature (brown). The grey shaded regions indicate the boundary layer thickness.
$\pm 0.5$ of the maximum. (c) Wall-normal profile of the streamwise (blue), wall-normal (green) and spanwise (orange) velocities, as well as the density (purple) and temperature (brown). The grey shaded regions indicate the boundary layer thickness.
So far, ![]() $\overline {Ma}(y)\geq 1$ just provides a condition for which we get modes that radiate into the free stream. The mechanism by which these modes cause high resolvent amplification is not obvious, and this will be discussed in § 5. Before that, we will briefly consider: (i) the contribution of these modes to the free stream disturbances of the boundary layer in § 4.1 and (ii) the impact of viscosity on these resolvent modes in § 4.2.
$\overline {Ma}(y)\geq 1$ just provides a condition for which we get modes that radiate into the free stream. The mechanism by which these modes cause high resolvent amplification is not obvious, and this will be discussed in § 5. Before that, we will briefly consider: (i) the contribution of these modes to the free stream disturbances of the boundary layer in § 4.1 and (ii) the impact of viscosity on these resolvent modes in § 4.2.
4.1. Contribution to the boundary layer and free stream
A crucial detail about these Mach waves is that, within the wavelength range considered here, these resolvent Mach waves are the the only modes that contribute to the response in the free stream. To illustrate this, in figure 6, the response to the masked resolvent is considered. The masking is done by introducing a weighting to the resolvent operator, as done by Nogueira et al. (Reference Nogueira, Cavalieri, Hanifi and Henningson2020) (see § 2.2). To obtain figure 6(![]() $a$), the resolvent is masked such that it gives a response only within the boundary layer, i.e. in the region
$a$), the resolvent is masked such that it gives a response only within the boundary layer, i.e. in the region ![]() $y \leqslant \delta _{99}$, and for figure 6(
$y \leqslant \delta _{99}$, and for figure 6(![]() $b$), the response is restricted to the free stream, i.e.
$b$), the response is restricted to the free stream, i.e. ![]() $y > \delta _{99}$. First, from figure 6(
$y > \delta _{99}$. First, from figure 6(![]() $a$), we observe that the subsonic modes lie within the boundary layer, consistent with the observations in § 3. The contribution to the free stream in figure 6(
$a$), we observe that the subsonic modes lie within the boundary layer, consistent with the observations in § 3. The contribution to the free stream in figure 6(![]() $b$) then comes solely from the Mach wave resolvent modes. Therefore, the resolvent Mach waves are the only contributors to the free stream fluctuations from the resolvent, at least within the wavenumber space considered here.
$b$) then comes solely from the Mach wave resolvent modes. Therefore, the resolvent Mach waves are the only contributors to the free stream fluctuations from the resolvent, at least within the wavenumber space considered here.

Figure 6. Masking of the resolvent operator to give a response (a) within the boundary layer (i.e. ![]() $y\leqslant d_{99}$) and (b) within the free stream (i.e.
$y\leqslant d_{99}$) and (b) within the free stream (i.e. ![]() $y>d_{99}$), separately. The leading resolvent gain
$y>d_{99}$), separately. The leading resolvent gain ![]() $\sigma _1$ from the masked operators is shown as a function of the streamwise and spanwise wavelengths
$\sigma _1$ from the masked operators is shown as a function of the streamwise and spanwise wavelengths ![]() $\lambda _x$ and
$\lambda _x$ and ![]() $\lambda _z$ and for a fixed phase speed
$\lambda _z$ and for a fixed phase speed ![]() $c = \bar {U}(y^+\approx 15)$. The dashed black line represents the relative Mach equal to unity line.
$c = \bar {U}(y^+\approx 15)$. The dashed black line represents the relative Mach equal to unity line.
4.2. Effect of viscosity on resolvent Mach wave amplification
From figure 1(![]() $b$), we see that the most linearly amplified supersonic modes are those that lie close to the
$b$), we see that the most linearly amplified supersonic modes are those that lie close to the ![]() $\overline {Ma}(\infty )=1$ line, and here we explore why this is the case. For the flows considered here, the maximum
$\overline {Ma}(\infty )=1$ line, and here we explore why this is the case. For the flows considered here, the maximum ![]() $\overline {Ma}(y)$ for the modes at the
$\overline {Ma}(y)$ for the modes at the ![]() $\overline {Ma}(\infty )=1$ line is
$\overline {Ma}(\infty )=1$ line is ![]() $1$ at
$1$ at ![]() $y=\delta _{99}$. Therefore, these modes have
$y=\delta _{99}$. Therefore, these modes have ![]() $\overline {Ma}(y)<1$ within the boundary layer, i.e.
$\overline {Ma}(y)<1$ within the boundary layer, i.e. ![]() $\overline {Ma}(y<\delta _{99})<1$. Mach waves only start radiating from the wall height where
$\overline {Ma}(y<\delta _{99})<1$. Mach waves only start radiating from the wall height where ![]() $\overline {Ma}(y) \geqslant 1$. Hence, these modes near the
$\overline {Ma}(y) \geqslant 1$. Hence, these modes near the ![]() $\overline {Ma}(\infty )=1$ line only start radiating at or near
$\overline {Ma}(\infty )=1$ line only start radiating at or near ![]() $y=\delta _{99}$, and they therefore do not have a presence within the boundary layer. These modes then exist in a region of the flow where the effect of viscosity is negligible. In contrast, supersonic modes that have
$y=\delta _{99}$, and they therefore do not have a presence within the boundary layer. These modes then exist in a region of the flow where the effect of viscosity is negligible. In contrast, supersonic modes that have ![]() $\overline {Ma}(\infty )>1$, i.e. fall much above the
$\overline {Ma}(\infty )>1$, i.e. fall much above the ![]() $\overline {Ma}(\infty )=1$ line, start radiating from within the boundary layer. These modes therefore experience the dampening effects of viscosity. This difference could explain why the most amplified supersonic modes lie close to the
$\overline {Ma}(\infty )=1$ line, start radiating from within the boundary layer. These modes therefore experience the dampening effects of viscosity. This difference could explain why the most amplified supersonic modes lie close to the ![]() $\overline {Ma}(\infty )=1$ line.
$\overline {Ma}(\infty )=1$ line.
To illustrate this, in figure 7, we compare the responses from the regular resolvent operator (black solid line) to fictional resolvent operators with viscosity artificially decreased to ![]() $1/10$ (red dash-dotted line) and
$1/10$ (red dash-dotted line) and ![]() $1/100$ (blue dash-dotted line) times the original value (note that these operators are not physical and are used here only for illustrative purposes). We saw in § 4.1 that only the Mach waves contribute to the free stream response from the resolvent. Therefore, to focus solely on the Mach waves, here, we ‘mask’ the resolvent such that the response from the resolvent is restricted to lie only in the free stream (see § 2.2). The vertical black dashed line represents
$1/100$ (blue dash-dotted line) times the original value (note that these operators are not physical and are used here only for illustrative purposes). We saw in § 4.1 that only the Mach waves contribute to the free stream response from the resolvent. Therefore, to focus solely on the Mach waves, here, we ‘mask’ the resolvent such that the response from the resolvent is restricted to lie only in the free stream (see § 2.2). The vertical black dashed line represents ![]() $\overline {Ma}(\infty )=1$ and we are only interested in the region to the right of this line. First, let us address the jagged response from the regular resolvent. Although mathematically we can have Mach waves at a continuous range of temporal frequencies (4.3), because of our discretised operators, we only resolve a discrete set of these frequencies in our linear operator. The jagged response therefore comes about because of the proximity (or remoteness) of the selected
$\overline {Ma}(\infty )=1$ and we are only interested in the region to the right of this line. First, let us address the jagged response from the regular resolvent. Although mathematically we can have Mach waves at a continuous range of temporal frequencies (4.3), because of our discretised operators, we only resolve a discrete set of these frequencies in our linear operator. The jagged response therefore comes about because of the proximity (or remoteness) of the selected ![]() $\omega$ to the nearest resolved frequency in the linear operator (Bae et al. Reference Bae, Dawson and McKeon2020b).
$\omega$ to the nearest resolved frequency in the linear operator (Bae et al. Reference Bae, Dawson and McKeon2020b).

Figure 7. Leading resolvent amplification for modes with ![]() $\lambda _x=1$ and
$\lambda _x=1$ and ![]() $c \approx \bar {U}(y^+ = 15)$ as a function of
$c \approx \bar {U}(y^+ = 15)$ as a function of ![]() $\lambda _z$. The solid line is obtained from the regular resolvent operator masked to give a response only within the free stream. The dashed lines are obtained from the fictional resolvent operators with viscosity artificially decreased to
$\lambda _z$. The solid line is obtained from the regular resolvent operator masked to give a response only within the free stream. The dashed lines are obtained from the fictional resolvent operators with viscosity artificially decreased to ![]() $1/10$ (red line) and
$1/10$ (red line) and ![]() $1/100$ (blue line) times the original value. The inset represents a zoomed-in version of the boxed region in the plot. The dashed line represents
$1/100$ (blue line) times the original value. The inset represents a zoomed-in version of the boxed region in the plot. The dashed line represents ![]() $\overline {Ma}(\infty )=1$.
$\overline {Ma}(\infty )=1$.
From this figure, we see that the amplification of the mode at the ![]() $\overline {Ma}(\infty )=1$ line (see inset of the figure which zooms in on this mode) is not significantly affected by the reduction in viscosity. However, when
$\overline {Ma}(\infty )=1$ line (see inset of the figure which zooms in on this mode) is not significantly affected by the reduction in viscosity. However, when ![]() $\overline {Ma}(\infty ) > 1$, reducing the dampening effect of viscosity results in more amplified modes. This suggests that the negligible effect of viscosity on the supersonic modes that lie at or close to the
$\overline {Ma}(\infty ) > 1$, reducing the dampening effect of viscosity results in more amplified modes. This suggests that the negligible effect of viscosity on the supersonic modes that lie at or close to the ![]() $\overline {Ma}(\infty )=1$ line is the reason why these modes are the most linearly amplified. These observations also suggests that, if in the future, the linearised equations augmented with an eddy-viscosity profile is used to study these free stream fluctuations (e.g. Del Álamo & Jiménez Reference Del Álamo and Jiménez2006; Pujals et al. Reference Pujals, García-Villalba, Cossu and Depardon2009; Hwang & Cossu Reference Hwang and Cossu2010), the amplification of modes with
$\overline {Ma}(\infty )=1$ line is the reason why these modes are the most linearly amplified. These observations also suggests that, if in the future, the linearised equations augmented with an eddy-viscosity profile is used to study these free stream fluctuations (e.g. Del Álamo & Jiménez Reference Del Álamo and Jiménez2006; Pujals et al. Reference Pujals, García-Villalba, Cossu and Depardon2009; Hwang & Cossu Reference Hwang and Cossu2010), the amplification of modes with ![]() $\overline {Ma}(\infty ) > 1$ could potentially be impacted, although their mode shapes within the free stream will likely not be affected (since eddy viscosity in the free stream will be zero). However, analysing the eddy viscosity based operator is beyond the scope of the current manuscript and is left for the future.
$\overline {Ma}(\infty ) > 1$ could potentially be impacted, although their mode shapes within the free stream will likely not be affected (since eddy viscosity in the free stream will be zero). However, analysing the eddy viscosity based operator is beyond the scope of the current manuscript and is left for the future.
5. Two routes of forcing resolvent Mach wave radiation
The ![]() $\overline {Ma}(y)\geq 1$ just provides a condition for which we get modes that radiate into the free stream. The mechanism by which these modes cause high resolvent amplification is not obvious. There are two routes, (i) direct and (ii) indirect, through which these Mach waves can cause high resolvent amplification, and here we will consider these two routes separately. In this section, two different supersonic resolvent modes from an
$\overline {Ma}(y)\geq 1$ just provides a condition for which we get modes that radiate into the free stream. The mechanism by which these modes cause high resolvent amplification is not obvious. There are two routes, (i) direct and (ii) indirect, through which these Mach waves can cause high resolvent amplification, and here we will consider these two routes separately. In this section, two different supersonic resolvent modes from an ![]() $Ma=4$,
$Ma=4$, ![]() $Re_\tau \approx 400$ turbulent boundary layer over an adiabatic wall are taken as examples: (i) ‘MW1 (Mach wave 1)’ with
$Re_\tau \approx 400$ turbulent boundary layer over an adiabatic wall are taken as examples: (i) ‘MW1 (Mach wave 1)’ with ![]() $\lambda _y=3.5$ in § 5.1 (the square
$\lambda _y=3.5$ in § 5.1 (the square ![]() $\blacksquare$ in figure 1
$\blacksquare$ in figure 1![]() $b$) and (ii) ‘MW2’ with
$b$) and (ii) ‘MW2’ with ![]() $\lambda _y=10$ in § 5.2 (the circle (
$\lambda _y=10$ in § 5.2 (the circle (![]() $\bullet$) in figure 1
$\bullet$) in figure 1![]() $b$). Both the modes have the same
$b$). Both the modes have the same ![]() $\lambda _x=5$ and
$\lambda _x=5$ and ![]() $c \approx \overline{U}(y^+=15)$. MW1 is chosen such that it falls close to the
$c \approx \overline{U}(y^+=15)$. MW1 is chosen such that it falls close to the ![]() $\overline {Ma}(\infty )=1$ line, while MW2 is taken such that it lies further away from the
$\overline {Ma}(\infty )=1$ line, while MW2 is taken such that it lies further away from the ![]() $\overline {Ma}(\infty )=1$ line. This choice has two significant consequences. First, modes that lie close to the
$\overline {Ma}(\infty )=1$ line. This choice has two significant consequences. First, modes that lie close to the ![]() $\overline {Ma}(\infty )=1$ line have higher amplifications relative to other supersonic modes (see figure 1
$\overline {Ma}(\infty )=1$ line have higher amplifications relative to other supersonic modes (see figure 1![]() $b$ and § 4.2). Therefore, MW1 has a higher resolvent amplification when compared with MW2. Second, the Mach wave radiates only when
$b$ and § 4.2). Therefore, MW1 has a higher resolvent amplification when compared with MW2. Second, the Mach wave radiates only when ![]() $\overline {Ma}(y) \geqslant 1$. For MW1, the free stream relative Mach number
$\overline {Ma}(y) \geqslant 1$. For MW1, the free stream relative Mach number ![]() $\overline {Ma}(\infty )$ (i.e. the maximum value of
$\overline {Ma}(\infty )$ (i.e. the maximum value of ![]() $\overline {Ma}(y)$) is close to
$\overline {Ma}(y)$) is close to ![]() $1$ and, therefore, the mode starts radiating from near
$1$ and, therefore, the mode starts radiating from near ![]() $y=\delta _{99}$ (see § 4.2). However, MW2 has
$y=\delta _{99}$ (see § 4.2). However, MW2 has ![]() $\overline {Ma}(\infty )$ greater than
$\overline {Ma}(\infty )$ greater than ![]() $1$ and, therefore, the mode starts radiating from well within the boundary layer (see § 4.2). We will see that this difference impacts the forcing mechanisms active for these modes. In this section: (1) the ‘direct route’ of forcing the Mach waves will be discussed in § 5.1 using MW1 and (2) the ‘indirect route’ in § 5.2 using MW2. In § 6, we will look at the relative contributions of these two forcing routes across
$1$ and, therefore, the mode starts radiating from well within the boundary layer (see § 4.2). We will see that this difference impacts the forcing mechanisms active for these modes. In this section: (1) the ‘direct route’ of forcing the Mach waves will be discussed in § 5.1 using MW1 and (2) the ‘indirect route’ in § 5.2 using MW2. In § 6, we will look at the relative contributions of these two forcing routes across ![]() $(\lambda _x,\lambda _y,c)$.
$(\lambda _x,\lambda _y,c)$.
5.1. Direct route of forcing resolvent Mach wave radiation
To probe the direct forcing route, consider the equations for the Mach waves (4.2) in the free stream. Consider the inverse of the resolvent operator ![]() $\boldsymbol{\mathsf{L}}=\boldsymbol{\mathsf{H}}^{-1}$, where
$\boldsymbol{\mathsf{L}}=\boldsymbol{\mathsf{H}}^{-1}$, where ![]() $\boldsymbol{\mathsf{L}}\hat {\boldsymbol {q}}=\hat {\boldsymbol {f}}$, (2.5). The SVD of this operator gives
$\boldsymbol{\mathsf{L}}\hat {\boldsymbol {q}}=\hat {\boldsymbol {f}}$, (2.5). The SVD of this operator gives ![]() $\boldsymbol{\mathsf{L}} = \sum _{i=1}^{5N} \boldsymbol{\phi}^{\boldsymbol{\prime}}_i \sigma '_i \boldsymbol {\psi}^{\boldsymbol{\prime}}_i$. For the inviscid case, solutions from (4.3)
$\boldsymbol{\mathsf{L}} = \sum _{i=1}^{5N} \boldsymbol{\phi}^{\boldsymbol{\prime}}_i \sigma '_i \boldsymbol {\psi}^{\boldsymbol{\prime}}_i$. For the inviscid case, solutions from (4.3) ![]() $\hat {p}_M(y)$ are solutions of
$\hat {p}_M(y)$ are solutions of ![]() $\boldsymbol{\mathsf{L}}$. Another way of saying this is that a singular vector of
$\boldsymbol{\mathsf{L}}$. Another way of saying this is that a singular vector of ![]() $\boldsymbol{\mathsf{L}}$, with a corresponding singular value of
$\boldsymbol{\mathsf{L}}$, with a corresponding singular value of ![]() $\sigma '_i=0$, has pressure equal to
$\sigma '_i=0$, has pressure equal to ![]() $\hat {p}_M(y)$.
$\hat {p}_M(y)$.
For the case of finite Reynolds numbers considered here, viscosity will damp these modes. Therefore, singular vectors with pressure ![]() $\hat {p}_M(y)$ will have singular values
$\hat {p}_M(y)$ will have singular values ![]() $\sigma '_i$ close to
$\sigma '_i$ close to ![]() $0$, but not
$0$, but not ![]() $0$. Let us now consider the SVD of the resolvent operator
$0$. Let us now consider the SVD of the resolvent operator ![]() $\boldsymbol{\mathsf{H}}$ in terms of the SVD of
$\boldsymbol{\mathsf{H}}$ in terms of the SVD of ![]() $\boldsymbol{\mathsf{L}}=\boldsymbol{\mathsf{H}}^{-1}$. This gives
$\boldsymbol{\mathsf{L}}=\boldsymbol{\mathsf{H}}^{-1}$. This gives ![]() $\boldsymbol{\mathsf{H}} = \sum _{i=1}^{5N} \boldsymbol {\psi}^{\boldsymbol{\prime}}_i (1/\sigma '_i) \boldsymbol {\phi}^{\boldsymbol{\prime}}_i$. There is
$\boldsymbol{\mathsf{H}} = \sum _{i=1}^{5N} \boldsymbol {\psi}^{\boldsymbol{\prime}}_i (1/\sigma '_i) \boldsymbol {\phi}^{\boldsymbol{\prime}}_i$. There is ![]() $1/\sigma '_i$ that appears in the SVD of
$1/\sigma '_i$ that appears in the SVD of ![]() $\boldsymbol{\mathsf{H}}$. Therefore, singular vectors of
$\boldsymbol{\mathsf{H}}$. Therefore, singular vectors of ![]() $\boldsymbol{\mathsf{L}}$ with pressure
$\boldsymbol{\mathsf{L}}$ with pressure ![]() $\hat {p}_M(y)$, which have
$\hat {p}_M(y)$, which have ![]() $\sigma '_i$ close to
$\sigma '_i$ close to ![]() $0$, will now appear as highly amplified singular vectors of
$0$, will now appear as highly amplified singular vectors of ![]() $\boldsymbol{\mathsf{H}}$. This is how the Mach waves
$\boldsymbol{\mathsf{H}}$. This is how the Mach waves ![]() $\hat {p}_M(y)$ cause high resolvent amplification. Note that this mechanism can amplify
$\hat {p}_M(y)$ cause high resolvent amplification. Note that this mechanism can amplify ![]() $(\lambda _x,\lambda _y)$ modes that are linearly stable. In figure 1(
$(\lambda _x,\lambda _y)$ modes that are linearly stable. In figure 1(![]() $b$), the green contour lines show the region of the
$b$), the green contour lines show the region of the ![]() $(\lambda _x,\lambda _y)$ space that is linearly unstable and we observe that there are supersonic modes outside of this region, i.e. that are linearly stable, but still have high resolvent amplification.
$(\lambda _x,\lambda _y)$ space that is linearly unstable and we observe that there are supersonic modes outside of this region, i.e. that are linearly stable, but still have high resolvent amplification.
From (4.2), we see that, within the free stream, the pressure cannot be forced by ![]() $\hat {v}$ (since all the terms within the square brackets are zero in the free stream). Therefore, only
$\hat {v}$ (since all the terms within the square brackets are zero in the free stream). Therefore, only ![]() $\boldsymbol {\nabla }\boldsymbol {\cdot }\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}$,
$\boldsymbol {\nabla }\boldsymbol {\cdot }\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}$, ![]() $\hat {f}_\theta$ or
$\hat {f}_\theta$ or ![]() $\hat {f}_\rho$ can excite the waves. Now consider the Helmholtz decomposition of
$\hat {f}_\rho$ can excite the waves. Now consider the Helmholtz decomposition of ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}$ that gives the solenoidal
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}$ that gives the solenoidal ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ and dilatational
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ and dilatational ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d$ components. Since, by the definition of the Helmholtz decomposition,
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d$ components. Since, by the definition of the Helmholtz decomposition, ![]() $\boldsymbol {\nabla }\boldsymbol {\cdot } \hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s = 0$, only the dilatational component
$\boldsymbol {\nabla }\boldsymbol {\cdot } \hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s = 0$, only the dilatational component ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^{d}$ can force these modes. To excite the Mach waves in the free stream, we therefore need a forcing in
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^{d}$ can force these modes. To excite the Mach waves in the free stream, we therefore need a forcing in ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^{d}$,
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^{d}$, ![]() $\hat {f}_\theta$ or
$\hat {f}_\theta$ or ![]() $\hat {f}_\rho$.
$\hat {f}_\rho$.
To illustrate this, in figures 8(![]() $a$)–8(
$a$)–8(![]() $e$), we consider the supersonic mode MW1 (modes MW1 and MW2 are defined at the beginning of § 5). The five components of the full leading resolvent response
$e$), we consider the supersonic mode MW1 (modes MW1 and MW2 are defined at the beginning of § 5). The five components of the full leading resolvent response ![]() $\boldsymbol {\psi }_1$ are shown in black (suboptimal modes are considered in Appendix A). The forcing
$\boldsymbol {\psi }_1$ are shown in black (suboptimal modes are considered in Appendix A). The forcing ![]() $\hat {\boldsymbol {f}}$ to this resolvent mode is the leading resolvent forcing mode
$\hat {\boldsymbol {f}}$ to this resolvent mode is the leading resolvent forcing mode ![]() $\boldsymbol {\phi }_1$. In red is the response to
$\boldsymbol {\phi }_1$. In red is the response to ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d$,
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d$, ![]() $\hat {f}_\rho$ and
$\hat {f}_\rho$ and ![]() $\hat {f}_\theta$, i.e. the forcing to the resolvent is
$\hat {f}_\theta$, i.e. the forcing to the resolvent is ![]() $\hat {\boldsymbol {f}}_{\!\!2}=(\,\hat {f}^d_u, \hat {f}^d_v, \hat {f}^d_w, \hat {f}_\rho, \hat {f}_\theta )$. In blue is the response to the remaining components of the forcing
$\hat {\boldsymbol {f}}_{\!\!2}=(\,\hat {f}^d_u, \hat {f}^d_v, \hat {f}^d_w, \hat {f}_\rho, \hat {f}_\theta )$. In blue is the response to the remaining components of the forcing ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$, i.e. the resolvent forcing is
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$, i.e. the resolvent forcing is ![]() $\hat {\boldsymbol {f}}_{\!\!1}=(\,\hat {f}^s_u, \hat {f}^s_v, \hat {f}^s_w, 0, 0)$. From figures 8(
$\hat {\boldsymbol {f}}_{\!\!1}=(\,\hat {f}^s_u, \hat {f}^s_v, \hat {f}^s_w, 0, 0)$. From figures 8(![]() $a$)–8(
$a$)–8(![]() $e$), we see that
$e$), we see that ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ is responsible for capturing the majority of the energy, and
$\hat {\boldsymbol {f}}_{\!\!2}$ is responsible for capturing the majority of the energy, and ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ plays an insignificant role for these modes. (The reason for why the contribution of
$\hat {\boldsymbol {f}}_{\!\!1}$ plays an insignificant role for these modes. (The reason for why the contribution of ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ in figures 8
$\hat {\boldsymbol {f}}_{\!\!1}$ in figures 8![]() $a$–8
$a$–8![]() $e$, although small, is still non-zero, is explained in § 5.2.) Further, the contribution of
$e$, although small, is still non-zero, is explained in § 5.2.) Further, the contribution of ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d$ alone is shown in a lighter shade of green and that of (
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d$ alone is shown in a lighter shade of green and that of (![]() $f_\rho,f_\theta$) is shown in a darker shade of green. For the mode MW1, we note that both these components contribute almost equally to the response.
$f_\rho,f_\theta$) is shown in a darker shade of green. For the mode MW1, we note that both these components contribute almost equally to the response.

Figure 8. Response of the resolvent operator to the full leading resolvent forcing mode ![]() $\hat {\boldsymbol {f}}=\boldsymbol {\phi }_1$ (black) as well as the response to the two components of the forcing
$\hat {\boldsymbol {f}}=\boldsymbol {\phi }_1$ (black) as well as the response to the two components of the forcing ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ (blue) and
$\hat {\boldsymbol {f}}_{\!\!1}$ (blue) and ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ (red). Further, the contribution of
$\hat {\boldsymbol {f}}_{\!\!2}$ (red). Further, the contribution of ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d$ alone is shown in a lighter shade of green and that of (
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d$ alone is shown in a lighter shade of green and that of (![]() $f_\rho$,
$f_\rho$,![]() $f_\theta$) is shown in a darker shade of green. Two compressible boundary layers are considered: (a–e)
$f_\theta$) is shown in a darker shade of green. Two compressible boundary layers are considered: (a–e) ![]() $Ma=4$,
$Ma=4$, ![]() $Re_\tau =400$ over an adiabatic wall and (f–j)
$Re_\tau =400$ over an adiabatic wall and (f–j) ![]() $Ma=6$,
$Ma=6$, ![]() $Re_\tau =450$ with
$Re_\tau =450$ with ![]() $\varTheta _w/\varTheta _{ad}=0.25$. Modes that fall close to the relative Mach equal to unity lines are shown: mode
$\varTheta _w/\varTheta _{ad}=0.25$. Modes that fall close to the relative Mach equal to unity lines are shown: mode ![]() $(\lambda _x,\lambda _z,c)=(5,3.5,\bar {U}(y^+\approx 15))$ for the
$(\lambda _x,\lambda _z,c)=(5,3.5,\bar {U}(y^+\approx 15))$ for the ![]() $Ma=4$ case (the mode indicated by the square (
$Ma=4$ case (the mode indicated by the square (![]() $\blacksquare$) in figure 1
$\blacksquare$) in figure 1![]() $b$) and mode
$b$) and mode ![]() $(\lambda _x,\lambda _z,c)=(5,1.6,\bar {U}(y^+\approx 15))$ for the
$(\lambda _x,\lambda _z,c)=(5,1.6,\bar {U}(y^+\approx 15))$ for the ![]() $Ma=6$ case. (a,f) Streamwise, (b,g) wall-normal and (c,h) spanwise velocities as well as the (d,i) density and (e,j) temperature.
$Ma=6$ case. (a,f) Streamwise, (b,g) wall-normal and (c,h) spanwise velocities as well as the (d,i) density and (e,j) temperature.
To access the more general applicability of the discussions here, in figures 8(![]() $f$)–8(
$f$)–8(![]() $j$), we also look at a supersonic mode for an
$j$), we also look at a supersonic mode for an ![]() $Re_\tau =450$ boundary layer flow at a higher Mach number of
$Re_\tau =450$ boundary layer flow at a higher Mach number of ![]() $Ma=6$ and over a cooled wall with
$Ma=6$ and over a cooled wall with ![]() $\varTheta _w/\varTheta _{ad}=0.25$. Here again, we choose a mode that falls close to the relative Mach equal to unity line which for
$\varTheta _w/\varTheta _{ad}=0.25$. Here again, we choose a mode that falls close to the relative Mach equal to unity line which for ![]() $c \approx \overline{U}(y^+=15)$ and
$c \approx \overline{U}(y^+=15)$ and ![]() $\lambda _x=5$ corresponds to
$\lambda _x=5$ corresponds to ![]() $\lambda _z=1.6$. We observe similar trends as in figures 8(
$\lambda _z=1.6$. We observe similar trends as in figures 8(![]() $a$)–8(
$a$)–8(![]() $e$), where
$e$), where ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ amplifies the mode. Therefore, wall cooling does not affect the trends discussed here.
$\hat {\boldsymbol {f}}_{\!\!2}$ amplifies the mode. Therefore, wall cooling does not affect the trends discussed here.
5.2. Indirect route of forcing resolvent Mach wave radiation
So far, we considered mode MW1 and found that mainly the forcing components ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^{d}$,
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^{d}$, ![]() $\hat {f}_\theta$ or
$\hat {f}_\theta$ or ![]() $\hat {f}_\rho$ excite the mode. Let us now consider mode MW2 where this is not the case. Figure 9 is the equivalent of figures 8(
$\hat {f}_\rho$ excite the mode. Let us now consider mode MW2 where this is not the case. Figure 9 is the equivalent of figures 8(![]() $a$)–8(
$a$)–8(![]() $e$), but for mode MW2. The five components of the full leading resolvent mode of MW2 is shown in black, along with the response to
$e$), but for mode MW2. The five components of the full leading resolvent mode of MW2 is shown in black, along with the response to ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ in blue and
$\hat {\boldsymbol {f}}_{\!\!1}$ in blue and ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ in red (the definitions of
$\hat {\boldsymbol {f}}_{\!\!2}$ in red (the definitions of ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ and
$\hat {\boldsymbol {f}}_{\!\!1}$ and ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ are the same as in § 5.1). Unlike for MW1, for MW2, we find that
$\hat {\boldsymbol {f}}_{\!\!2}$ are the same as in § 5.1). Unlike for MW1, for MW2, we find that ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ (blue line), and therefore the solenoidal component of the forcing to the momentum equations,
$\hat {\boldsymbol {f}}_{\!\!1}$ (blue line), and therefore the solenoidal component of the forcing to the momentum equations, ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$, plays a significant role in capturing the mode. The question then is, why does
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$, plays a significant role in capturing the mode. The question then is, why does ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ excite MW2, but not MW1?
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ excite MW2, but not MW1?

Figure 9. Response of the resolvent operator to the full leading resolvent forcing mode ![]() $\hat {\boldsymbol {f}}=\boldsymbol {\phi }_1$ (black) as well as the response to the two components of the forcing
$\hat {\boldsymbol {f}}=\boldsymbol {\phi }_1$ (black) as well as the response to the two components of the forcing ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ (blue) and
$\hat {\boldsymbol {f}}_{\!\!1}$ (blue) and ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ (red). Further, the contribution of
$\hat {\boldsymbol {f}}_{\!\!2}$ (red). Further, the contribution of ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d$ alone is shown in a lighter shade of green and that of (
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d$ alone is shown in a lighter shade of green and that of (![]() $f_\rho,f_\theta$) is shown in a darker shade of green. A supersonic mode with
$f_\rho,f_\theta$) is shown in a darker shade of green. A supersonic mode with ![]() $(\lambda _x,\lambda _z,c)=(5,10,\bar {U}(y^+\approx 15))$ for a compressible boundary layer over an adiabatic wall with
$(\lambda _x,\lambda _z,c)=(5,10,\bar {U}(y^+\approx 15))$ for a compressible boundary layer over an adiabatic wall with ![]() $Ma=4$,
$Ma=4$, ![]() $Re_\tau =400$ is considered (the mode indicated by the circle (
$Re_\tau =400$ is considered (the mode indicated by the circle (![]() $\bullet$) in figure 1
$\bullet$) in figure 1![]() $b$). (a) Streamwise, (b) wall-normal and (c) spanwise velocities as well as the (d) density and (e) temperature.
$b$). (a) Streamwise, (b) wall-normal and (c) spanwise velocities as well as the (d) density and (e) temperature.
To understand this, first, from (4.2), we note that wall-normal velocity ![]() $\hat {v}$ can excite the resolvent Mach waves. Second, from figure 1(
$\hat {v}$ can excite the resolvent Mach waves. Second, from figure 1(![]() $b$), we note that both MW1 and MW2 lie within the grey contour line, which represents the region where incompressible mechanisms are reasonably active. Therefore, a possible route through which
$b$), we note that both MW1 and MW2 lie within the grey contour line, which represents the region where incompressible mechanisms are reasonably active. Therefore, a possible route through which ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ can excite Mach waves is as follows: incompressible-like mechanisms cause
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ can excite Mach waves is as follows: incompressible-like mechanisms cause ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ to excite a response in
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ to excite a response in ![]() $\hat {v}$, which in turn excites the Mach waves. Here, we will call this the indirect route of forcing the Mach waves. However, this mechanism still does not explain the differences between the trends in the forcing to MW1 and MW2, specifically, why the indirect route is prominent for MW2 and not for MW1.
$\hat {v}$, which in turn excites the Mach waves. Here, we will call this the indirect route of forcing the Mach waves. However, this mechanism still does not explain the differences between the trends in the forcing to MW1 and MW2, specifically, why the indirect route is prominent for MW2 and not for MW1.
To probe this, in figure 10(![]() $a$), we consider MW1 and in figure 10(
$a$), we consider MW1 and in figure 10(![]() $b$) we consider MW2. Two separate profiles are plotted in these figures. The first profile,
$b$) we consider MW2. Two separate profiles are plotted in these figures. The first profile, ![]() $\hat {p}_M(y)$, is obtained from the analytic expression for the Mach wave radiation (4.3), and indicates the wall-normal region where these Mach waves exist. The red dashed line represents the wall height above which the relative Mach number
$\hat {p}_M(y)$, is obtained from the analytic expression for the Mach wave radiation (4.3), and indicates the wall-normal region where these Mach waves exist. The red dashed line represents the wall height above which the relative Mach number ![]() $\overline {Ma}(y)\geqslant 1$ and, therefore,
$\overline {Ma}(y)\geqslant 1$ and, therefore, ![]() $\hat {p}_M(y)$ starts radiating only from this wall height. To compute the second profile,
$\hat {p}_M(y)$ starts radiating only from this wall height. To compute the second profile, ![]() $(\psi ^{in}_1)_{v}(y)$, we consider a fictional incompressible resolvent operator
$(\psi ^{in}_1)_{v}(y)$, we consider a fictional incompressible resolvent operator ![]() $\boldsymbol{\mathsf{H}}^{in}$ (i.e. the resolvent operator at
$\boldsymbol{\mathsf{H}}^{in}$ (i.e. the resolvent operator at ![]() $Ma=0$), but with the mean velocity profile
$Ma=0$), but with the mean velocity profile ![]() $\bar {U}$ from the compressible case. In effect,
$\bar {U}$ from the compressible case. In effect, ![]() $\boldsymbol{\mathsf{H}}^{in}$ captures the mechanisms that would hypothetically be active in an
$\boldsymbol{\mathsf{H}}^{in}$ captures the mechanisms that would hypothetically be active in an ![]() $Ma=0$ flow that maintains the compressible mean velocity profile (
$Ma=0$ flow that maintains the compressible mean velocity profile (![]() $\boldsymbol{\mathsf{H}}^{in}$ is not physically relevant and is used here only for illustration). In other words,
$\boldsymbol{\mathsf{H}}^{in}$ is not physically relevant and is used here only for illustration). In other words, ![]() $\boldsymbol{\mathsf{H}}^{in}$ should be able to approximately capture the incompressible mechanisms in this flow. The profile
$\boldsymbol{\mathsf{H}}^{in}$ should be able to approximately capture the incompressible mechanisms in this flow. The profile ![]() $(\psi ^{in}_1)_{v}(y)$ is the wall-normal velocity component of the leading resolvent mode obtained from
$(\psi ^{in}_1)_{v}(y)$ is the wall-normal velocity component of the leading resolvent mode obtained from ![]() $\boldsymbol{\mathsf{H}}^{in}$. Finally, the red shaded region represents the overlap between
$\boldsymbol{\mathsf{H}}^{in}$. Finally, the red shaded region represents the overlap between ![]() $\hat {p}_M(y)$ and
$\hat {p}_M(y)$ and ![]() $(\psi ^{in}_1)_{v}(y)$ (in this region,
$(\psi ^{in}_1)_{v}(y)$ (in this region, ![]() $\overline {Ma}(y) \geqslant 1$ and, therefore,
$\overline {Ma}(y) \geqslant 1$ and, therefore, ![]() $\hat {p}_M(y)$ exists, and
$\hat {p}_M(y)$ exists, and ![]() $(\psi ^{in}_1)_{v}(y)$ is at least
$(\psi ^{in}_1)_{v}(y)$ is at least ![]() $10\,\%$ of its maximum value). We see that, since MW2 starts radiating from well within the boundary layer, this region of overlap is significantly higher for the mode. The
$10\,\%$ of its maximum value). We see that, since MW2 starts radiating from well within the boundary layer, this region of overlap is significantly higher for the mode. The ![]() $\hat {v}$ generated through incompressible-like mechanisms forced by
$\hat {v}$ generated through incompressible-like mechanisms forced by ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ can therefore much more efficiently drive the Mach waves in MW2, in comparison with MW1. This provides a possible explanation for why
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ can therefore much more efficiently drive the Mach waves in MW2, in comparison with MW1. This provides a possible explanation for why ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ plays a significant role in exciting MW2. The relatively small overlap observed in the case of MW1 also explains why the solenoidal component gives a small but non-zero response (blue line) in figures 8(
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ plays a significant role in exciting MW2. The relatively small overlap observed in the case of MW1 also explains why the solenoidal component gives a small but non-zero response (blue line) in figures 8(![]() $a$)–8(
$a$)–8(![]() $e$).
$e$).

Figure 10. Analytical Mach wave ![]() $\hat {p}_M(y)$ in (4.2) and
$\hat {p}_M(y)$ in (4.2) and ![]() $(\psi ^{in}_1)_{v}(y)$ the wall-normal velocity obtained from the incompressible resolvent with the compressible mean
$(\psi ^{in}_1)_{v}(y)$ the wall-normal velocity obtained from the incompressible resolvent with the compressible mean ![]() $\boldsymbol{\mathsf{H}}^{in}$ for the modes (a) MW1 and (b) MW2. The grey shaded region represents the region within the boundary layer. The red shaded region represents the overlap between
$\boldsymbol{\mathsf{H}}^{in}$ for the modes (a) MW1 and (b) MW2. The grey shaded region represents the region within the boundary layer. The red shaded region represents the overlap between ![]() $\hat {p}_M(y)$ and
$\hat {p}_M(y)$ and ![]() $(\psi ^{in}_1)_{v}(y)$, where
$(\psi ^{in}_1)_{v}(y)$, where ![]() $\overline {Ma}(y) \geqslant 1$ and therefore
$\overline {Ma}(y) \geqslant 1$ and therefore ![]() $\hat {p}_M(y)$ exists, and
$\hat {p}_M(y)$ exists, and ![]() $(\psi ^{in}_1)_{v}(y)$ is at least
$(\psi ^{in}_1)_{v}(y)$ is at least ![]() $10\,\%$ of its maximum value.
$10\,\%$ of its maximum value.
From this, we see that there are two factors that are responsible for this indirect forcing. (1) The modes should have active incompressible-like mechanisms. This is true for both MW1 and MW2, and can be measured by the leading singular value of ![]() $\boldsymbol{\mathsf{H}}^{in}$,
$\boldsymbol{\mathsf{H}}^{in}$, ![]() $\sigma ^{in}_{1}(\lambda _x,\lambda _y,c)$. (2) There should be an overlap between
$\sigma ^{in}_{1}(\lambda _x,\lambda _y,c)$. (2) There should be an overlap between ![]() $\hat {p}_M(y)$ and
$\hat {p}_M(y)$ and ![]() $(\psi ^{in}_1)_{v}(y)$. This is more significant for MW2 and can be measured using a projection of
$(\psi ^{in}_1)_{v}(y)$. This is more significant for MW2 and can be measured using a projection of ![]() $(\psi ^{in}_1)_{v}$ onto
$(\psi ^{in}_1)_{v}$ onto ![]() $\hat {p}_M(y)$ defined as
$\hat {p}_M(y)$ defined as
 \begin{equation} \eta(\lambda_x,\lambda_y,c) = \frac{ \left( \displaystyle\int\nolimits_0^{y_{f}}\hat{p}_M(y) (\psi^{in}_1)_{v}^*(y) \right)^2}{ \displaystyle\int\nolimits_0^{y_{f}} \hat{p}_M(y) \hat{p}_M^*(y) \displaystyle\int\nolimits_0^{y_{f}} (\psi^{in}_1)_{v}(y) (\psi^{in}_1)_{v}^*(y) }. \end{equation}
\begin{equation} \eta(\lambda_x,\lambda_y,c) = \frac{ \left( \displaystyle\int\nolimits_0^{y_{f}}\hat{p}_M(y) (\psi^{in}_1)_{v}^*(y) \right)^2}{ \displaystyle\int\nolimits_0^{y_{f}} \hat{p}_M(y) \hat{p}_M^*(y) \displaystyle\int\nolimits_0^{y_{f}} (\psi^{in}_1)_{v}(y) (\psi^{in}_1)_{v}^*(y) }. \end{equation}
Therefore, if this mechanism is indeed responsible for amplification, then the mode should have a higher value of ![]() $(\sigma ^{in}_{1})^2 \eta$. The wall height
$(\sigma ^{in}_{1})^2 \eta$. The wall height ![]() $y_f$ is the height until which we force the resolvent and here,
$y_f$ is the height until which we force the resolvent and here, ![]() $y_f=3$ (see § 2.4). The value of
$y_f=3$ (see § 2.4). The value of ![]() $(\sigma ^{in}_{1})^2 \eta$ is approximately
$(\sigma ^{in}_{1})^2 \eta$ is approximately ![]() $102$ for MW1 and
$102$ for MW1 and ![]() $202$ for MW2, showing that the indirect route is more active for MW2 (although computing this number shows that this route is not completely absent for MW1, but just less significant). We will use this metric
$202$ for MW2, showing that the indirect route is more active for MW2 (although computing this number shows that this route is not completely absent for MW1, but just less significant). We will use this metric ![]() $(\sigma ^{in}_{1})^2 \eta$ in § 6.
$(\sigma ^{in}_{1})^2 \eta$ in § 6.
6. Contribution of the direct and indirect forcing mechanisms
So far, we have considered a decomposition of the forcing to the resolvent into two components: (1) ![]() $\hat {\boldsymbol {f}}_{\!\!1}=(\,\hat {f}^s_u, \hat {f}^s_v, \hat {f}^s_w, 0, 0)$, which contains the solenoidal component of the forcing to the momentum equations; and (2)
$\hat {\boldsymbol {f}}_{\!\!1}=(\,\hat {f}^s_u, \hat {f}^s_v, \hat {f}^s_w, 0, 0)$, which contains the solenoidal component of the forcing to the momentum equations; and (2) ![]() $\hat {\boldsymbol {f}}_{\!\!2}=(\,\hat {f}^d_u, \hat {f}^d_v, \hat {f}^d_w, \hat {f}_\rho, \hat {f}_\theta )$, which contains the dilatational component of the forcing to the momentum equations as well as the forcing to the density and temperature equations. Subsonic modes are forced by
$\hat {\boldsymbol {f}}_{\!\!2}=(\,\hat {f}^d_u, \hat {f}^d_v, \hat {f}^d_w, \hat {f}_\rho, \hat {f}_\theta )$, which contains the dilatational component of the forcing to the momentum equations as well as the forcing to the density and temperature equations. Subsonic modes are forced by ![]() $\hat {\boldsymbol {f}}_{\!\!1}$. However, there are two routes through which the supersonic modes can be forced: the direct route, where
$\hat {\boldsymbol {f}}_{\!\!1}$. However, there are two routes through which the supersonic modes can be forced: the direct route, where ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ is active, and the indirect route, where
$\hat {\boldsymbol {f}}_{\!\!2}$ is active, and the indirect route, where ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ plays a significant role. So far, we have only looked at individual
$\hat {\boldsymbol {f}}_{\!\!1}$ plays a significant role. So far, we have only looked at individual ![]() $(\lambda _x,\lambda _y,c)$ modes and the purpose of this section is to analyse the relative importance of
$(\lambda _x,\lambda _y,c)$ modes and the purpose of this section is to analyse the relative importance of ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ and
$\hat {\boldsymbol {f}}_{\!\!1}$ and ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ over a range of
$\hat {\boldsymbol {f}}_{\!\!2}$ over a range of ![]() $(\lambda _x, \lambda _y,c)$.
$(\lambda _x, \lambda _y,c)$.
In figure 11, for the case of a turbulent boundary layer at ![]() $Ma=4$ and
$Ma=4$ and ![]() $Re_\tau =400$, the full resolvent response in figure 11(a,d) is compared with responses to
$Re_\tau =400$, the full resolvent response in figure 11(a,d) is compared with responses to ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ in figure 11(b,e) and
$\hat {\boldsymbol {f}}_{\!\!1}$ in figure 11(b,e) and ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ in figure 11(c,f). Figure 11(a–c) shows the responses as a function of (
$\hat {\boldsymbol {f}}_{\!\!2}$ in figure 11(c,f). Figure 11(a–c) shows the responses as a function of (![]() $\lambda _x,\lambda _z$) at a fixed phase speed of
$\lambda _x,\lambda _z$) at a fixed phase speed of ![]() $c=\overline{U}(y^+\approx 15)$. To consider a range of
$c=\overline{U}(y^+\approx 15)$. To consider a range of ![]() $c$, figure 11(d–f) shows the responses as a function of
$c$, figure 11(d–f) shows the responses as a function of ![]() $c$ and
$c$ and ![]() $\lambda _z$, at a fixed value of
$\lambda _z$, at a fixed value of ![]() $\lambda _x=5$. (The values of
$\lambda _x=5$. (The values of ![]() $c$ in figures 11(
$c$ in figures 11(![]() $d$)–11(
$d$)–11(![]() $f$) are taken only until
$f$) are taken only until ![]() $0.75$, since the amplification of the subsonic streaks with
$0.75$, since the amplification of the subsonic streaks with ![]() $c>0.75$ is too high to be clearly depicted along with the lesser amplified supersonic modes.) The black contour lines represent
$c>0.75$ is too high to be clearly depicted along with the lesser amplified supersonic modes.) The black contour lines represent ![]() $-1:1:2$ contours of
$-1:1:2$ contours of ![]() $\log _{10}((\sigma ^{in}_{1})^2 \eta )$ which, as seen in § 5.2, indicate the region where (i) incompressible-like mechanisms are active and (ii)
$\log _{10}((\sigma ^{in}_{1})^2 \eta )$ which, as seen in § 5.2, indicate the region where (i) incompressible-like mechanisms are active and (ii) ![]() $(\psi ^{in}_1)_{v}$ projects onto
$(\psi ^{in}_1)_{v}$ projects onto ![]() $\hat {p}_M(y)$ (see § 5.2). The black dashed lines in the figures (or curves in the case of figure 11d–f) indicates relative Mach number equal to unity
$\hat {p}_M(y)$ (see § 5.2). The black dashed lines in the figures (or curves in the case of figure 11d–f) indicates relative Mach number equal to unity ![]() $\overline {Ma}(\infty )=1$. Note that the colour scales in this figure are logarithmic.
$\overline {Ma}(\infty )=1$. Note that the colour scales in this figure are logarithmic.

Figure 11. Chu norm of the response of the resolvent operator to (a,d) the full leading resolvent forcing mode ![]() $\boldsymbol {\phi }_1$ as well as the two components of the forcing (b,e)
$\boldsymbol {\phi }_1$ as well as the two components of the forcing (b,e) ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ and (c,f)
$\hat {\boldsymbol {f}}_{\!\!1}$ and (c,f) ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ in two ways: (a–c) as a function of the streamwise and spanwise wavelengths
$\hat {\boldsymbol {f}}_{\!\!2}$ in two ways: (a–c) as a function of the streamwise and spanwise wavelengths ![]() $(\lambda _x,\lambda _z)$ at a fixed phase speed
$(\lambda _x,\lambda _z)$ at a fixed phase speed ![]() $c=\bar {U}(y^+\approx 15)$ and (d–f) as a function of phase speeds and spanwise wavelengths (
$c=\bar {U}(y^+\approx 15)$ and (d–f) as a function of phase speeds and spanwise wavelengths (![]() $c,\lambda _z$) at a fixed streamwise wavelength
$c,\lambda _z$) at a fixed streamwise wavelength ![]() $\lambda _x=5$. The vertical dash-dotted line in panel (a) indicates
$\lambda _x=5$. The vertical dash-dotted line in panel (a) indicates ![]() $\lambda _x=5$ and in panel (d), indicates
$\lambda _x=5$ and in panel (d), indicates ![]() $c=\bar {U}(y^+\approx 15)$. The solid black contours in panel (b) indicate
$c=\bar {U}(y^+\approx 15)$. The solid black contours in panel (b) indicate ![]() $-1:1:2$ contours of
$-1:1:2$ contours of ![]() $\log _{10}((\sigma ^{in}_{1})^2 \eta )$. The dashed black contour lines indicate the relative Mach equal to unity.
$\log _{10}((\sigma ^{in}_{1})^2 \eta )$. The dashed black contour lines indicate the relative Mach equal to unity.
From figures 11(![]() $b$) and 11(
$b$) and 11(![]() $e$), we observe that
$e$), we observe that ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ captures the responses in the subsonic modes, i.e. the responses in the region below the
$\hat {\boldsymbol {f}}_{\!\!1}$ captures the responses in the subsonic modes, i.e. the responses in the region below the ![]() $\overline {Ma}(\infty )=1$ line. Figures 11(
$\overline {Ma}(\infty )=1$ line. Figures 11(![]() $c$) and 11(
$c$) and 11(![]() $f$) show that
$f$) show that ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ captures the majority of the response in the supersonic modes, i.e. the responses in the region above the
$\hat {\boldsymbol {f}}_{\!\!2}$ captures the majority of the response in the supersonic modes, i.e. the responses in the region above the ![]() $\overline {Ma}(\infty )=1$ line. This is especially true when considering the most amplified supersonic modes that are near the
$\overline {Ma}(\infty )=1$ line. This is especially true when considering the most amplified supersonic modes that are near the ![]() $\overline {Ma}(\infty )=1$ line, consistent with observations in § 5. Also in line with § 5, from figures 11(
$\overline {Ma}(\infty )=1$ line, consistent with observations in § 5. Also in line with § 5, from figures 11(![]() $b$) and 11(
$b$) and 11(![]() $e$), we note that there is a small set of supersonic modes where the contribution of
$e$), we note that there is a small set of supersonic modes where the contribution of ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ is not zero. The black contour lines in figure 11(
$\hat {\boldsymbol {f}}_{\!\!1}$ is not zero. The black contour lines in figure 11(![]() $b$) follow the trends of these modes, which suggests that an incompressible-like mechanism is active, and this mechanism can force the Mach waves. Therefore, the indirect route of forcing (§ 5.2) is active for these modes.
$b$) follow the trends of these modes, which suggests that an incompressible-like mechanism is active, and this mechanism can force the Mach waves. Therefore, the indirect route of forcing (§ 5.2) is active for these modes.
In figure 12, we consider an ![]() $Ma=6$,
$Ma=6$, ![]() $Re_\tau =450$ turbulent boundary layer over a cooled wall with wall-cooling ratio
$Re_\tau =450$ turbulent boundary layer over a cooled wall with wall-cooling ratio ![]() $\varTheta _w/\varTheta _{ad}=0.25$, with figures 12(
$\varTheta _w/\varTheta _{ad}=0.25$, with figures 12(![]() $a$), 12(
$a$), 12(![]() $b$) and 12(
$b$) and 12(![]() $c$) showing responses to the full forcing, to
$c$) showing responses to the full forcing, to ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ and to
$\hat {\boldsymbol {f}}_{\!\!1}$ and to ![]() $\hat {\boldsymbol {f}}_{\!\!2}$, respectively. We observe that the same trends as observed for the
$\hat {\boldsymbol {f}}_{\!\!2}$, respectively. We observe that the same trends as observed for the ![]() $Ma=4$ boundary layer is valid here, indicating that wall cooling does not impact the trends discussed. These discussions are also equally valid for laminar compressible boundary layers, and Appendix C briefly discusses this case. Another point to note is that we have so far only discussed the most amplified first resolvent mode, and Appendix A contains a brief discussion on the effect of the different components of the forcing on the sub-optimal resolvent modes.
$Ma=4$ boundary layer is valid here, indicating that wall cooling does not impact the trends discussed. These discussions are also equally valid for laminar compressible boundary layers, and Appendix C briefly discusses this case. Another point to note is that we have so far only discussed the most amplified first resolvent mode, and Appendix A contains a brief discussion on the effect of the different components of the forcing on the sub-optimal resolvent modes.

Figure 12. For a turbulent boundary layer with ![]() $Ma=6$,
$Ma=6$, ![]() $Re_\tau =450$ and
$Re_\tau =450$ and ![]() $\varTheta _w/\varTheta _{ad}=0.25$, the Chu norm of the response of the resolvent operator to (a) the full leading resolvent forcing mode
$\varTheta _w/\varTheta _{ad}=0.25$, the Chu norm of the response of the resolvent operator to (a) the full leading resolvent forcing mode ![]() $\boldsymbol {\phi }_1$ as well as the two components of the forcing (b)
$\boldsymbol {\phi }_1$ as well as the two components of the forcing (b) ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ and (c)
$\hat {\boldsymbol {f}}_{\!\!1}$ and (c) ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ as a function of the streamwise (
$\hat {\boldsymbol {f}}_{\!\!2}$ as a function of the streamwise (![]() $\lambda _x$) and spanwise (
$\lambda _x$) and spanwise (![]() $\lambda _z$) wavelengths for a fixed value of phase speed
$\lambda _z$) wavelengths for a fixed value of phase speed ![]() $c=\bar {U}(y^+\approx 15)$. The dashed lines indicate the relative Mach number equal to unity.
$c=\bar {U}(y^+\approx 15)$. The dashed lines indicate the relative Mach number equal to unity.
From these observations, we see that within the majority of the wavenumber space above the ![]() $\overline {Ma}(\infty )=1$ line, the contribution of
$\overline {Ma}(\infty )=1$ line, the contribution of ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ is orders of magnitude higher than the contribution of
$\hat {\boldsymbol {f}}_{\!\!2}$ is orders of magnitude higher than the contribution of ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ (given that the colour scale is logarithmic). This is especially true for the most amplified resolvent modes that lie close to the
$\hat {\boldsymbol {f}}_{\!\!1}$ (given that the colour scale is logarithmic). This is especially true for the most amplified resolvent modes that lie close to the ![]() $\overline {Ma}(\infty )=1$ line. However, this does not mean that the contribution of
$\overline {Ma}(\infty )=1$ line. However, this does not mean that the contribution of ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ is not significant for supersonic modes. In the next section, we will discuss a comparison with DNS, where
$\hat {\boldsymbol {f}}_{\!\!1}$ is not significant for supersonic modes. In the next section, we will discuss a comparison with DNS, where ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ can be seen to play a dominant role in exciting the supersonic modes of the flow.
$\hat {\boldsymbol {f}}_{\!\!1}$ can be seen to play a dominant role in exciting the supersonic modes of the flow.
7. Resolvent Mach waves and Mach waves from DNS: a discussion
In this section, we will discuss the resolvent Mach waves alongside trends of these waves that are known from DNS (e.g. Duan et al. Reference Duan, Choudhari and Wu2014, Reference Duan, Choudhari and Zhang2016; Zhang et al. Reference Zhang, Duan and Choudhari2017). The turbulence within the boundary layer forces Mach wave radiation. DNS studies show that with increasing ![]() $Ma$, the free stream inclination angles of these Mach waves decrease and their propagation velocities increase (Duan et al. Reference Duan, Choudhari and Zhang2016). However, increasing wall cooling does not seem to impact propagation speeds of the waves, but the inclination angles are reported to be slightly steeper (Zhang et al. Reference Zhang, Duan and Choudhari2017). Crucially, these studies employed acoustic analogy based arguments, and identified the sources of these waves to lie within the buffer layer of the flow.
$Ma$, the free stream inclination angles of these Mach waves decrease and their propagation velocities increase (Duan et al. Reference Duan, Choudhari and Zhang2016). However, increasing wall cooling does not seem to impact propagation speeds of the waves, but the inclination angles are reported to be slightly steeper (Zhang et al. Reference Zhang, Duan and Choudhari2017). Crucially, these studies employed acoustic analogy based arguments, and identified the sources of these waves to lie within the buffer layer of the flow.
To analyse these Mach waves that are radiated by the buffer layer, we have to move away from using resolvent analysis over the entire wall-normal domain, as has been done in previous works (e.g. Bugeat et al. Reference Bugeat, Chassaing, Robinet and Sagaut2019; Bae et al. Reference Bae, Dawson and McKeon2020b). We will instead use the ‘masked resolvent’, where the forcing and the response to the resolvent is masked, i.e. restricted to lie in particular wall-normal regions of the flow. First, considering the response, Mach wave responses alone are of interest here and we have observed that these Mach waves are the sole contributors to the free stream resolvent response (see § 4.1). Therefore, the resolvent response can be masked such that it lies within the free stream alone. Next, considering the forcing, DNS studies have shown that the sources to these waves lie within the buffer layer of the flow (e.g. Duan et al. Reference Duan, Choudhari and Wu2014, Reference Duan, Choudhari and Zhang2016; Zhang et al. Reference Zhang, Duan and Choudhari2017). Therefore, the resolvent forcing can be masked such that it is localised within the buffer layer. So we are here considering the resolvent operator such that its forcing can only lie within ![]() $y^+<30$ and the response within
$y^+<30$ and the response within ![]() $y>\delta _{99}$. The weighting function introduced by Nogueira et al. (Reference Nogueira, Cavalieri, Hanifi and Henningson2020) is used to mask the resolvent (see § 2.2).
$y>\delta _{99}$. The weighting function introduced by Nogueira et al. (Reference Nogueira, Cavalieri, Hanifi and Henningson2020) is used to mask the resolvent (see § 2.2).
Figure 13 shows the response to such a masked resolvent, with figures 13(![]() $a$), 13(
$a$), 13(![]() $b$) and 13(
$b$) and 13(![]() $c$) showing the response to the full forcing, to
$c$) showing the response to the full forcing, to ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ and to
$\hat {\boldsymbol {f}}_{\!\!1}$ and to ![]() $\hat {\boldsymbol {f}}_{\!\!2}$, respectively. Here,
$\hat {\boldsymbol {f}}_{\!\!2}$, respectively. Here, ![]() $c=\bar {U}(y^+\approx 15)$ is taken from within the buffer layer. Comparing figure 13(
$c=\bar {U}(y^+\approx 15)$ is taken from within the buffer layer. Comparing figure 13(![]() $a$) with figure 11(
$a$) with figure 11(![]() $b$), the response of the masked resolvent is concentrated in the region where we found that the indirect route of forcing is significant. Additionally, from figures 13(
$b$), the response of the masked resolvent is concentrated in the region where we found that the indirect route of forcing is significant. Additionally, from figures 13(![]() $b$) and 13(
$b$) and 13(![]() $c$), we see that
$c$), we see that ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ captures almost all of the response. Therefore, based on the discussions in § 6, we can postulate that the indirect route of forcing amplifies these modes. Purely from a resolvent perspective, we can therefore hypothesise that the Mach wave radiation due to the turbulence within the buffer layer of the boundary layer is generated due to the indirect route of forcing as described in § 5.2.
$\hat {\boldsymbol {f}}_{\!\!1}$ captures almost all of the response. Therefore, based on the discussions in § 6, we can postulate that the indirect route of forcing amplifies these modes. Purely from a resolvent perspective, we can therefore hypothesise that the Mach wave radiation due to the turbulence within the buffer layer of the boundary layer is generated due to the indirect route of forcing as described in § 5.2.

Figure 13. Response of the masked resolvent; the response is masked to lie solely within the free stream and the forcing to lie within ![]() $y^+<30$. The Chu norm of the response of the resolvent operator to (a) the full leading resolvent forcing mode
$y^+<30$. The Chu norm of the response of the resolvent operator to (a) the full leading resolvent forcing mode ![]() $\boldsymbol {\phi }_1$ as well as the two components of the forcing (b)
$\boldsymbol {\phi }_1$ as well as the two components of the forcing (b) ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ and (c)
$\hat {\boldsymbol {f}}_{\!\!1}$ and (c) ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ as a function of the streamwise (
$\hat {\boldsymbol {f}}_{\!\!2}$ as a function of the streamwise (![]() $\lambda _x$) and spanwise (
$\lambda _x$) and spanwise (![]() $\lambda _z$) wavelengths for a fixed value of phase speed
$\lambda _z$) wavelengths for a fixed value of phase speed ![]() $c \approx \bar {U}(y^+=15)$. The
$c \approx \bar {U}(y^+=15)$. The ![]() $Ma=4$,
$Ma=4$, ![]() $Re_\tau =400$ turbulent boundary layer over an adiabatic wall is considered. The dashed black line indicates the relative Mach number equal to unity line.
$Re_\tau =400$ turbulent boundary layer over an adiabatic wall is considered. The dashed black line indicates the relative Mach number equal to unity line.
Presuming the accuracy of this hypothesis, both the indirect route of Mach wave forcing and the subsonic modes are forced by the solenoidal component of the forcing. This could potentially reduce the problem of modelling the full high-rank nonlinear forcing in these flows to the simpler problem of modelling only the lower-rank solenoidal component of the forcing.
7.1. Inclination angle of the supersonic resolvent modes
DNS studies have investigated the free stream inclination angles of the Mach wave radiation generated from the boundary layer (e.g. Duan et al. Reference Duan, Choudhari and Wu2014; Zhang et al. Reference Zhang, Duan and Choudhari2017). Although DNS datasets show some degree of variability in the free stream inclination angles observed, these studies consistently emphasise the existence of a statistically dominant angle. When considering the Mach waves from the resolvent model, we can use the fact that these waves are pressure oscillations of the form given by (4.3) and compute the free stream inclination angle of these Mach waves as
 \begin{equation} \angle_{FS} = \tan^{{-}1} \left( \frac{-1j}{\sqrt{1+(\lambda_x/\lambda_z)^2-{Ma}^2 (1-c)^2}} \right). \end{equation}
\begin{equation} \angle_{FS} = \tan^{{-}1} \left( \frac{-1j}{\sqrt{1+(\lambda_x/\lambda_z)^2-{Ma}^2 (1-c)^2}} \right). \end{equation}
At a fixed Mach number, we see that the inclination angle depends on the aspect ratio ![]() $\lambda _x/\lambda _z$ and the phase speed
$\lambda _x/\lambda _z$ and the phase speed ![]() $c$ of the mode. From the resolvent, we therefore have a range of inclination angles. The mechanism that picks out the statistically dominant inclination angles found in DNS is still not clear. To probe into this mechanism, in the future, there is a requirement of comparing DNS data with the resolvent model. More specifically, constructing a resolvent-based low-order model of the free stream radiations using DNS data from the buffer layer alone will potentially elucidate the mechanism that is responsible for the selection of a predominant angle in DNS studies. This detailed comparison with DNS, however, is beyond the scope of the current manuscript, and is left as an important future direction of work.
$c$ of the mode. From the resolvent, we therefore have a range of inclination angles. The mechanism that picks out the statistically dominant inclination angles found in DNS is still not clear. To probe into this mechanism, in the future, there is a requirement of comparing DNS data with the resolvent model. More specifically, constructing a resolvent-based low-order model of the free stream radiations using DNS data from the buffer layer alone will potentially elucidate the mechanism that is responsible for the selection of a predominant angle in DNS studies. This detailed comparison with DNS, however, is beyond the scope of the current manuscript, and is left as an important future direction of work.
Although we cannot do a one-to-one comparison with DNS, we can still compare some of the trends observed in DNS with that from the resolvent model. For this, in figure 14, the solid lines show the inclination angles of the resolvent free stream radiation computed from (7.1) as a function of Mach number for a range of aspect ratios and phase speeds. The phase speed increases in the direction of the arrow in figure 14 and, for each phase speed, lighter coloured lines represent larger aspect ratios. First, we observe that phase speeds have a more prominent impact on the free stream inclination angles, when compared with aspect ratios. Second, from (7.1), we observe that wall cooling does not have a direct impact on the inclination angles of the structures. It only has an indirect effect through a control on the values of ![]() $c$ that can be admitted for these waves (through the differences in mean profiles due to wall cooling). Finally, we note that, in general, the inclination angles obtained from the model decrease with increasing Mach number, and this trend is consistent with DNS (Duan et al. Reference Duan, Choudhari and Wu2014).
$c$ that can be admitted for these waves (through the differences in mean profiles due to wall cooling). Finally, we note that, in general, the inclination angles obtained from the model decrease with increasing Mach number, and this trend is consistent with DNS (Duan et al. Reference Duan, Choudhari and Wu2014).

Figure 14. Inclination angle of the inviscid Mach waves given by (7.1) with respect to Mach number for a range of aspect ratios and phase speeds. Three different phase speeds of ![]() $c=0.3$,
$c=0.3$, ![]() $0.5$ and
$0.5$ and ![]() $0.7$ increasing in the direction of the arrow and three different values of aspect ratios
$0.7$ increasing in the direction of the arrow and three different values of aspect ratios ![]() $\lambda _x/\lambda _z=0$,
$\lambda _x/\lambda _z=0$, ![]() $0.5$ and
$0.5$ and ![]() $1.0$ are considered. The markers indicate the average inclination angles of the Mach waves from DNS reported in the literature (Duan et al. Reference Duan, Choudhari and Wu2014; Zhang et al. Reference Zhang, Duan and Choudhari2017). The DNS data at
$1.0$ are considered. The markers indicate the average inclination angles of the Mach waves from DNS reported in the literature (Duan et al. Reference Duan, Choudhari and Wu2014; Zhang et al. Reference Zhang, Duan and Choudhari2017). The DNS data at ![]() $Ma=6$ are for the case of cooled walls, and the two markers indicate two different ratios of
$Ma=6$ are for the case of cooled walls, and the two markers indicate two different ratios of ![]() $\varTheta _w/\varTheta _{ad}=0.76$ (blue) and
$\varTheta _w/\varTheta _{ad}=0.76$ (blue) and ![]() $0.25$ (red). The intervals represent the range of inclination angles predicted by the resolvent model under two assumptions: (1)
$0.25$ (red). The intervals represent the range of inclination angles predicted by the resolvent model under two assumptions: (1) ![]() $k_z=0$ and (2)
$k_z=0$ and (2) ![]() $\bar {U}(y^+ = 10) \leqslant c \leqslant \bar {U}(y^+ = 20)$.
$\bar {U}(y^+ = 10) \leqslant c \leqslant \bar {U}(y^+ = 20)$.
The markers show the values of the average free stream inclination angles that are obtained from DNS (Duan et al. Reference Duan, Choudhari and Wu2014; Zhang et al. Reference Zhang, Duan and Choudhari2017) from an ![]() $Ma=2.5$ flow over an adiabatic wall, and an
$Ma=2.5$ flow over an adiabatic wall, and an ![]() $Ma=6$ flow with two wall-cooling ratios of
$Ma=6$ flow with two wall-cooling ratios of ![]() $\varTheta _w/\varTheta _{ad}=0.76$ and
$\varTheta _w/\varTheta _{ad}=0.76$ and ![]() $0.25$ (indicated by the two markers at
$0.25$ (indicated by the two markers at ![]() $Ma=6$). The intervals plotted show the range of inclination angles predicted by the resolvent under two assumptions: (1) the phase speed lies within the buffer layer
$Ma=6$). The intervals plotted show the range of inclination angles predicted by the resolvent under two assumptions: (1) the phase speed lies within the buffer layer ![]() $\bar {U}(y^+ \approx 10) \leqslant c \leqslant \bar {U}(y^+ \approx 20)$ and (2) the aspect ratio is zero, since from figure 13, the most amplified resolvent modes that can be forced from the buffer layer tend to have large
$\bar {U}(y^+ \approx 10) \leqslant c \leqslant \bar {U}(y^+ \approx 20)$ and (2) the aspect ratio is zero, since from figure 13, the most amplified resolvent modes that can be forced from the buffer layer tend to have large ![]() $\lambda _z$ (also see Bugeat et al. Reference Bugeat, Chassaing, Robinet and Sagaut2019). It could be argued that when the effect of wall cooling is not significant (green and blue markers), the average inclination angles obtained from DNS fall roughly within the range of inclination angles that are observed from the model. However, there are major discrepancies. First, the range of inclination angles predicted for
$\lambda _z$ (also see Bugeat et al. Reference Bugeat, Chassaing, Robinet and Sagaut2019). It could be argued that when the effect of wall cooling is not significant (green and blue markers), the average inclination angles obtained from DNS fall roughly within the range of inclination angles that are observed from the model. However, there are major discrepancies. First, the range of inclination angles predicted for ![]() $Ma=2.5$ is too large for any meaningful comparison to be made. Second, DNS predicts an increase in the inclination angle with increasing wall cooling, while resolvent predicts the opposite trend, i.e. a decrease in the inclination angle.
$Ma=2.5$ is too large for any meaningful comparison to be made. Second, DNS predicts an increase in the inclination angle with increasing wall cooling, while resolvent predicts the opposite trend, i.e. a decrease in the inclination angle.
These differences could be due to several reasons and some of them are: (1) the assumption of phase speeds and aspect ratios that we have considered for plotting the intervals are oversimplified; or (2) the rank-1 resolvent model that we have used here is not sufficient. However, the most obvious restriction in comparing the inclination angles, as done here, is the assumption that all ![]() $(k_x,k_z,c)$ modes are forced equally. This cannot be true, since the dominant turbulent structures within the boundary layer will determine the extent to which these modes are forced. These turbulent structures can also be impacted by wall cooling, thereby influencing the dominant angles of these radiations. To address this, there is again the need to construct resolvent-based low-rank models of the free stream, and this is an important future direction of work.
$(k_x,k_z,c)$ modes are forced equally. This cannot be true, since the dominant turbulent structures within the boundary layer will determine the extent to which these modes are forced. These turbulent structures can also be impacted by wall cooling, thereby influencing the dominant angles of these radiations. To address this, there is again the need to construct resolvent-based low-rank models of the free stream, and this is an important future direction of work.
8. Mach wave radiation from a streamwise developing boundary layer
The aim of this section is to briefly show that the mechanisms generating the Mach wave radiations, as discussed here, can be generalised to a streamwise developing boundary layer. In this case, the mean velocity is a function of the streamwise and wall-normal directions ![]() $\bar {U}(x,y)$. Unlike in the case of the one-dimensional (1-D) boundary layer, for the growing boundary layer, the wall-normal mean velocity
$\bar {U}(x,y)$. Unlike in the case of the one-dimensional (1-D) boundary layer, for the growing boundary layer, the wall-normal mean velocity ![]() $\bar {V}(x,y)$ is not zero. Without the simplifying assumptions for the 1-D boundary layer, the inviscid equations for pressure in (4.2) will now become
$\bar {V}(x,y)$ is not zero. Without the simplifying assumptions for the 1-D boundary layer, the inviscid equations for pressure in (4.2) will now become
 \begin{align} &\omega^2p - 2\omega\bar{U}_i \frac{\partial p}{\partial x_i} + \bar{U}_i\bar{U}_j \frac{\partial p}{\partial x_i \partial x_j} + \bar{U}_i \frac{\partial \bar{U}_j }{\partial x_i}\frac{\partial p}{\partial x_j} + \gamma \omega \frac{\partial \bar{U}_j }{\partial x_j} p +\gamma \bar{U}_i \frac{\partial \bar{U}_j }{\partial x_j} \frac{\partial p}{\partial x_i} \nonumber\\ &\quad - \gamma \frac{\partial \bar{U}_i }{\partial x_j}\frac{\partial \bar{U}_j }{\partial x_i} p - \frac{1}{M^2} \frac{\partial \bar{\varTheta} }{\partial x_j} \frac{\partial p}{\partial x_j} - \frac{\bar{\varTheta}}{M^2} \frac{\partial^2 p}{\partial x_j^2}= \gamma \left[ 2\frac{\partial \bar{U}_i}{\partial x_j} \frac{\partial }{\partial x_i} +\frac{\partial^2 \bar{U}_i }{\partial x_i\partial x_j} \right] u_j\nonumber\\ &\quad + \left[ \omega +\bar{U}_i \frac{\partial }{\partial x_i}\right] \left( \bar{\varTheta}f_\rho+f_\theta \right) - \gamma \frac{\partial f_{u_i}}{\partial x_i}. \end{align}
\begin{align} &\omega^2p - 2\omega\bar{U}_i \frac{\partial p}{\partial x_i} + \bar{U}_i\bar{U}_j \frac{\partial p}{\partial x_i \partial x_j} + \bar{U}_i \frac{\partial \bar{U}_j }{\partial x_i}\frac{\partial p}{\partial x_j} + \gamma \omega \frac{\partial \bar{U}_j }{\partial x_j} p +\gamma \bar{U}_i \frac{\partial \bar{U}_j }{\partial x_j} \frac{\partial p}{\partial x_i} \nonumber\\ &\quad - \gamma \frac{\partial \bar{U}_i }{\partial x_j}\frac{\partial \bar{U}_j }{\partial x_i} p - \frac{1}{M^2} \frac{\partial \bar{\varTheta} }{\partial x_j} \frac{\partial p}{\partial x_j} - \frac{\bar{\varTheta}}{M^2} \frac{\partial^2 p}{\partial x_j^2}= \gamma \left[ 2\frac{\partial \bar{U}_i}{\partial x_j} \frac{\partial }{\partial x_i} +\frac{\partial^2 \bar{U}_i }{\partial x_i\partial x_j} \right] u_j\nonumber\\ &\quad + \left[ \omega +\bar{U}_i \frac{\partial }{\partial x_i}\right] \left( \bar{\varTheta}f_\rho+f_\theta \right) - \gamma \frac{\partial f_{u_i}}{\partial x_i}. \end{align}
Here, ![]() $(x_1,x_2,x_3)=(x,y,z)$,
$(x_1,x_2,x_3)=(x,y,z)$, ![]() $(u_1,u_2,u_3)=(u,v,w)$,
$(u_1,u_2,u_3)=(u,v,w)$, ![]() $(\bar {U}_1,\bar {U}_2,\bar {U}_3)=(\bar {U},\bar {V},0)$ and
$(\bar {U}_1,\bar {U}_2,\bar {U}_3)=(\bar {U},\bar {V},0)$ and ![]() $(f_{u_1},f_{u_2},f_{u_3})=(f_x,f_y,f_z)$. A Fourier transform is taken in the homogeneous spanwise direction
$(f_{u_1},f_{u_2},f_{u_3})=(f_x,f_y,f_z)$. A Fourier transform is taken in the homogeneous spanwise direction ![]() $z$. Within the free stream, the left-hand side of (8.1) can be solved using separation of variables to obtain solutions of the form (Mack Reference Mack1990)
$z$. Within the free stream, the left-hand side of (8.1) can be solved using separation of variables to obtain solutions of the form (Mack Reference Mack1990)
Here, ![]() $A$ is a constant at
$A$ is a constant at ![]() $(k_z,\omega )$, and
$(k_z,\omega )$, and ![]() $r_x$ and
$r_x$ and ![]() $r_y$ can be obtained by solving the ordinary differential equations obtained from the separation of variables. These wavenumbers follow one of these two sets of quadratic equations: (i)
$r_y$ can be obtained by solving the ordinary differential equations obtained from the separation of variables. These wavenumbers follow one of these two sets of quadratic equations: (i) ![]() $c_1 r_x^2 - c_3r_x - l_1 = 0$ and
$c_1 r_x^2 - c_3r_x - l_1 = 0$ and ![]() $c_2 r_y^2 + (c_5 r_x - c_4) r_y + (l_1 + c_6) = 0$ or (ii)
$c_2 r_y^2 + (c_5 r_x - c_4) r_y + (l_1 + c_6) = 0$ or (ii) ![]() $c_2 r_y^2 - c_4r_y - l_2 = 0$ and
$c_2 r_y^2 - c_4r_y - l_2 = 0$ and ![]() $c_1 r_x^2 + (c_5 r_y - c_3) r_x + (l_2 + c_6) = 0$. The constants depend on the mean free stream quantities alone as
$c_1 r_x^2 + (c_5 r_y - c_3) r_x + (l_2 + c_6) = 0$. The constants depend on the mean free stream quantities alone as ![]() $c_1 = (1 - 1/M^2)$,
$c_1 = (1 - 1/M^2)$, ![]() $c_2 = (\bar {V}_\infty ^2 - 1/M^2)$,
$c_2 = (\bar {V}_\infty ^2 - 1/M^2)$, ![]() $c_3 = 2\omega$,
$c_3 = 2\omega$, ![]() $c_4 = 2\omega \bar {V}_\infty$,
$c_4 = 2\omega \bar {V}_\infty$, ![]() $c_5 = 2\bar {V}_\infty$ and
$c_5 = 2\bar {V}_\infty$ and ![]() $c_6 = (\omega ^2 - k_z^2/M^2 )$, where
$c_6 = (\omega ^2 - k_z^2/M^2 )$, where ![]() $\bar {V}_\infty$ represents the free stream wall-normal velocity normalised by
$\bar {V}_\infty$ represents the free stream wall-normal velocity normalised by ![]() $U_\infty$. By varying the constant
$U_\infty$. By varying the constant ![]() $l_1$ (or
$l_1$ (or ![]() $l_2$), we can get a family of solutions at a range of
$l_2$), we can get a family of solutions at a range of ![]() $(r_x,r_y)$. Therefore, for the streamwise developing boundary layer, we can obtain wave-like solutions within the free stream, consistent with the observations for the 1-D case in the rest of the manuscript (Mack Reference Mack1990).
$(r_x,r_y)$. Therefore, for the streamwise developing boundary layer, we can obtain wave-like solutions within the free stream, consistent with the observations for the 1-D case in the rest of the manuscript (Mack Reference Mack1990).
Now considering the right-hand side of (8.1) and comparing it with the 1-D boundary layer (4.2), we can obtain similar observations. There are two routes of forcing. First, the dilatational part of the forcing to the momentum equations ![]() $\hat {\boldsymbol {f}}_d$ (obtained through
$\hat {\boldsymbol {f}}_d$ (obtained through ![]() $\partial f_{u_i}/\partial x_i$), along with the forcing to the continuity
$\partial f_{u_i}/\partial x_i$), along with the forcing to the continuity ![]() $f_\rho$ and energy
$f_\rho$ and energy ![]() $f_\theta$ equations, can directly force the pressure waves. This gives the direct route of forcing as in § 5.1. Second, the solenoidal component of the forcing can generate a response in velocity
$f_\theta$ equations, can directly force the pressure waves. This gives the direct route of forcing as in § 5.1. Second, the solenoidal component of the forcing can generate a response in velocity ![]() $u_j$, which in turn can force the pressure equations within the boundary layer. (Although
$u_j$, which in turn can force the pressure equations within the boundary layer. (Although ![]() $u_j$ cannot be assumed to be zero in the free stream, the terms within the square brackets that multiply with
$u_j$ cannot be assumed to be zero in the free stream, the terms within the square brackets that multiply with ![]() $u_j$ can be assumed to be zero in this region.) This gives us the indirect route of forcing as in § 5.2. Consistent with prior observations from the 1-D case, the indirect route of forcing involves the mean shear profiles and can therefore be expected to be dominant in the buffer layer where this mean shear is high.
$u_j$ can be assumed to be zero in this region.) This gives us the indirect route of forcing as in § 5.2. Consistent with prior observations from the 1-D case, the indirect route of forcing involves the mean shear profiles and can therefore be expected to be dominant in the buffer layer where this mean shear is high.
As done in § 4, to confirm if these conclusions derived from the inviscid solutions do indeed capture features of the resolvent at a finite Reynolds number, figure 15 shows a 2-D resolvent mode. To obtain the 2-D resolvent mode, the linearised discrete Navier–Stokes equations contained in the matrix ![]() $\boldsymbol {A}$ in (2.3) are now constructed using the 2-D mean profiles
$\boldsymbol {A}$ in (2.3) are now constructed using the 2-D mean profiles ![]() $\bar {U}(x,y)$ and
$\bar {U}(x,y)$ and ![]() $\bar {V}(x,y)$ obtained from Duan et al. (Reference Duan, Choudhari and Zhang2016). The derivatives are now
$\bar {V}(x,y)$ obtained from Duan et al. (Reference Duan, Choudhari and Zhang2016). The derivatives are now ![]() $(\partial /\partial x,\partial /\partial y,\partial /\partial z)=(\partial /\partial x,\partial /\partial y, i k_z)$. The boundary conditions in the free stream are the same as for the 1-D resolvent (see § 2.4). The inlet and outlet boundary conditions are the non-reflecting Navier–Stokes characteristic boundary conditions (Poinsot & Lele Reference Poinsot and Lele1992) with damping sponges to prevent any reflections (Freund Reference Freund1997). For the 2-D resolvent, at a particular value of
$(\partial /\partial x,\partial /\partial y,\partial /\partial z)=(\partial /\partial x,\partial /\partial y, i k_z)$. The boundary conditions in the free stream are the same as for the 1-D resolvent (see § 2.4). The inlet and outlet boundary conditions are the non-reflecting Navier–Stokes characteristic boundary conditions (Poinsot & Lele Reference Poinsot and Lele1992) with damping sponges to prevent any reflections (Freund Reference Freund1997). For the 2-D resolvent, at a particular value of ![]() $(k_z,\omega )$, we obtain a range of modes at different
$(k_z,\omega )$, we obtain a range of modes at different ![]() $k_x$ that includes both subsonic and supersonic modes. To obtain just the Mach wave radiation that is of interest here, we will therefore consider the resolvent with the response masked such that it lies only in the free stream.
$k_x$ that includes both subsonic and supersonic modes. To obtain just the Mach wave radiation that is of interest here, we will therefore consider the resolvent with the response masked such that it lies only in the free stream.

Figure 15. Leading resolvent response mode obtained from the 2-D resolvent analysis for spanwise wavenumber ![]() $k_z=12.62$ and temporal frequency
$k_z=12.62$ and temporal frequency ![]() $\omega =3.15$. (a,d) Full response, with the resolvent masked such that the response is solely in the free stream. Also shown are the responses to the forcing (b,e)
$\omega =3.15$. (a,d) Full response, with the resolvent masked such that the response is solely in the free stream. Also shown are the responses to the forcing (b,e) ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ and (c,f)
$\hat {\boldsymbol {f}}_{\!\!1}$ and (c,f) ![]() $\hat {\boldsymbol {f}}_{\!\!2}$. The modes obtained from two different masking for the forcing are shown: (a–c) the forcing lies throughout the boundary layer and part of the free stream, here until
$\hat {\boldsymbol {f}}_{\!\!2}$. The modes obtained from two different masking for the forcing are shown: (a–c) the forcing lies throughout the boundary layer and part of the free stream, here until ![]() $y\leq 3$, and (d–f) the forcing lies within the buffer layer,
$y\leq 3$, and (d–f) the forcing lies within the buffer layer, ![]() $y^+\leq 30$. Note, for clarity, only the free stream and a subset of the streamwise domain used to compute the resolvent is shown here. The approximate streamwise wavenumber
$y^+\leq 30$. Note, for clarity, only the free stream and a subset of the streamwise domain used to compute the resolvent is shown here. The approximate streamwise wavenumber ![]() $k_x$ and phase speed
$k_x$ and phase speed ![]() $c$ obtained using a Fourier transform of the mode, are also shown. The contour lines in panels (a,d) show the analytical solution obtained by solving the inviscid pressure equations in the free stream, with red and blue contour lines representing positive and negative pressure fluctuations, respectively.
$c$ obtained using a Fourier transform of the mode, are also shown. The contour lines in panels (a,d) show the analytical solution obtained by solving the inviscid pressure equations in the free stream, with red and blue contour lines representing positive and negative pressure fluctuations, respectively.
Figures 15(![]() $a$) and 15(
$a$) and 15(![]() $d$) show the pressure obtained from the 2-D resolvent mode as the filled contours. Note, for clarity, only the free stream and a subset of the streamwise domain used to compute the resolvent is shown in the figure. The line contours represent the solution to the inviscid free stream pressure equation (8.2). The constant for the quadratic equation
$d$) show the pressure obtained from the 2-D resolvent mode as the filled contours. Note, for clarity, only the free stream and a subset of the streamwise domain used to compute the resolvent is shown in the figure. The line contours represent the solution to the inviscid free stream pressure equation (8.2). The constant for the quadratic equation ![]() $l_1$ (or
$l_1$ (or ![]() $l_2$) is fixed such that
$l_2$) is fixed such that ![]() $r_x$ (or
$r_x$ (or ![]() $r_y$) matches the streamwise (or wall-normal) wavenumber of the resolvent mode in the free stream, which is obtained from a Fourier transform. Here, red and blue represent positive and negative fluctuations, respectively. An
$r_y$) matches the streamwise (or wall-normal) wavenumber of the resolvent mode in the free stream, which is obtained from a Fourier transform. Here, red and blue represent positive and negative fluctuations, respectively. An ![]() $Ma=5.86$,
$Ma=5.86$, ![]() $T_w/T_{ad}\approx 0.76, T_\infty = 55\ {\rm K}$ boundary layer is considered here. A temporal frequency
$T_w/T_{ad}\approx 0.76, T_\infty = 55\ {\rm K}$ boundary layer is considered here. A temporal frequency ![]() $\omega =3.15$ is chosen, since, from DNS, we know that this frequency is energetic in the free stream (Duan et al. Reference Duan, Choudhari and Zhang2016), and a spanwise wavenumber of
$\omega =3.15$ is chosen, since, from DNS, we know that this frequency is energetic in the free stream (Duan et al. Reference Duan, Choudhari and Zhang2016), and a spanwise wavenumber of ![]() $k_z=12.62$ is chosen. The dash-dotted line represents the Mach angle computed as
$k_z=12.62$ is chosen. The dash-dotted line represents the Mach angle computed as ![]() $\sin ^{-1}{(1/Ma)}$. Unlike in the 1-D resolvent, due to the finite nature of the domain, the forcing is restricted to a finite streamwise extent and, therefore, the radiation from the resulting response modes will be restricted by the Mach angle (note, as seen in the figure, the inclination angle of the mode can be different from this Mach angle). The forcing and response are not masked in the streamwise direction. Two options for masking the forcing in the wall-normal direction are used: (i) in figures 15(
$\sin ^{-1}{(1/Ma)}$. Unlike in the 1-D resolvent, due to the finite nature of the domain, the forcing is restricted to a finite streamwise extent and, therefore, the radiation from the resulting response modes will be restricted by the Mach angle (note, as seen in the figure, the inclination angle of the mode can be different from this Mach angle). The forcing and response are not masked in the streamwise direction. Two options for masking the forcing in the wall-normal direction are used: (i) in figures 15(![]() $a$)–15(
$a$)–15(![]() $c$), the forcing is allowed to lie anywhere within the boundary layer or part of the free stream, i.e. at
$c$), the forcing is allowed to lie anywhere within the boundary layer or part of the free stream, i.e. at ![]() $y\leq 3$; and (ii) in figures 15(
$y\leq 3$; and (ii) in figures 15(![]() $d$)–15(
$d$)–15(![]() $f$), the forcing lies exclusively within the buffer layer of the flow, i.e.
$f$), the forcing lies exclusively within the buffer layer of the flow, i.e. ![]() $y^+\leqslant 30$. The response to the forcing
$y^+\leqslant 30$. The response to the forcing ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ is shown in figures 15(
$\hat {\boldsymbol {f}}_{\!\!1}$ is shown in figures 15(![]() $b$) and 15(
$b$) and 15(![]() $e$), and to
$e$), and to ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ in figures 15(
$\hat {\boldsymbol {f}}_{\!\!2}$ in figures 15(![]() $c$) and 15(
$c$) and 15(![]() $f$).
$f$).
First, from figures 15(![]() $a$) and 15(
$a$) and 15(![]() $d$), we see that the inviscid equations do match the resolvent modes reasonably well. This shows that, like we observed for the 1-D resolvent, the 2-D resolvent also admits wave-like solutions in the free stream, which can be analytically modelled as solutions to the inviscid free stream equations. Second, comparing figures 15(
$d$), we see that the inviscid equations do match the resolvent modes reasonably well. This shows that, like we observed for the 1-D resolvent, the 2-D resolvent also admits wave-like solutions in the free stream, which can be analytically modelled as solutions to the inviscid free stream equations. Second, comparing figures 15(![]() $b$) and 15(
$b$) and 15(![]() $c$), we see that when the forcing is allowed reach to
$c$), we see that when the forcing is allowed reach to ![]() $y=3$,
$y=3$, ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ contributes dominantly to the mode. In other words, in this case, through the direct route, the dilatational component of the forcing to the momentum equations, and the forcing to the energy and continuity equations force the mode. However, from figures 15(
$\hat {\boldsymbol {f}}_{\!\!2}$ contributes dominantly to the mode. In other words, in this case, through the direct route, the dilatational component of the forcing to the momentum equations, and the forcing to the energy and continuity equations force the mode. However, from figures 15(![]() $e$) and 15(
$e$) and 15(![]() $f$), we see that this observation is no longer true when the forcing is within the buffer layer alone. In this case, through the indirect route, the solenoidal component of the forcing to the momentum equations
$f$), we see that this observation is no longer true when the forcing is within the buffer layer alone. In this case, through the indirect route, the solenoidal component of the forcing to the momentum equations ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ dominates the response.
$\hat {\boldsymbol {f}}_{\!\!1}$ dominates the response.
Therefore, similar to the 1-D resolvent, there are two routes through which the Mach waves in the 2-D resolvent can be forced: (i) the direct and (ii) the indirect route. The indirect route likely becomes more important when considering modes forced by the buffer layer of the flow. This is consistent with the results obtained from the 1-D resolvent, and therefore shows that, in this case, the conclusions drawn here from the 1-D resolvent analysis are valid for the trends from the 2-D resolvent. Also consistent with the 1-D resolvent, by varying ![]() $k_z$, we can obtain modes (at a range of
$k_z$, we can obtain modes (at a range of ![]() $k_x$) with different ratios of the solenoidal to the dilatational responses (not shown here for brevity). It would be interesting, in the future, to understand how the pressure radiations obtained in (8.2) may relate to mechanisms where the spatial modulation of wavepackets leads to radiation (e.g. Reba, Narayanan & Colonius Reference Reba, Narayanan and Colonius2010; Jordan & Colonius Reference Jordan and Colonius2013). A detailed analysis of the 2-D resolvent is therefore important and is a crucial future direction of work.
$k_x$) with different ratios of the solenoidal to the dilatational responses (not shown here for brevity). It would be interesting, in the future, to understand how the pressure radiations obtained in (8.2) may relate to mechanisms where the spatial modulation of wavepackets leads to radiation (e.g. Reba, Narayanan & Colonius Reference Reba, Narayanan and Colonius2010; Jordan & Colonius Reference Jordan and Colonius2013). A detailed analysis of the 2-D resolvent is therefore important and is a crucial future direction of work.
9. Conclusions
We identified the forcing mechanisms that separately amplify the subsonic and the supersonic modes in a linearised Navier–Stokes equations-based model of compressible boundary layer flows. The resolvent analysis framework was used for this purpose, where the nonlinear terms of the linearised momentum (![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}$), continuity (
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}$), continuity (![]() $\hat {f}_\rho$) and temperature (
$\hat {f}_\rho$) and temperature (![]() $\hat {f}_\theta$) equations were treated as a forcing to the linearised equations. To identify the separate forcing mechanisms that are active, we use a Helmholtz decomposition of
$\hat {f}_\theta$) equations were treated as a forcing to the linearised equations. To identify the separate forcing mechanisms that are active, we use a Helmholtz decomposition of ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}$ that gives two components: (i) the divergence-free solenoidal component
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}$ that gives two components: (i) the divergence-free solenoidal component ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ and (ii) the curl-free dilatational component
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ and (ii) the curl-free dilatational component ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d$.
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d$.
First, considering the subsonic modes, these are structures localised within the boundary layer and are similar to structures in the incompressible flow (figures 2 and figure 6). The velocity fluctuations of these structures are divergence-free (figure 3). Only the solenoidal component of the forcing to the momentum equations ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ can amplify these modes (figure 11). All the other components of forcing, which includes the dilatational component
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ can amplify these modes (figure 11). All the other components of forcing, which includes the dilatational component ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d$ as well as
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d$ as well as ![]() $\hat {f}_\rho$ and
$\hat {f}_\rho$ and ![]() $\hat {f}_\theta$, play a negligible role in amplifying the subsonic modes. This is consistent with what has been previously observed in the incompressible regime (e.g. Rosenberg & McKeon Reference Rosenberg and McKeon2019). It is interesting that similar trends are here obtained for compressible flows as well.
$\hat {f}_\theta$, play a negligible role in amplifying the subsonic modes. This is consistent with what has been previously observed in the incompressible regime (e.g. Rosenberg & McKeon Reference Rosenberg and McKeon2019). It is interesting that similar trends are here obtained for compressible flows as well.
Now considering the supersonic modes, i.e. the resolvent Mach waves, these modes are pressure fluctuations that radiate into the free stream of the boundary layer (figure 5). These modes contribute to the energy in the free stream of the flow (figures 6). The most amplified resolvent Mach waves start radiating from the edge of the boundary layer, where the dampening effect of viscosity is negligible. Importantly, within the free stream, these resolvent modes closely follow the trends of the inviscid Mach waves (Mack Reference Mack1984). We identified two routes through which these modes can be amplified: (i) the ‘direct route’, where ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d$,
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^d$, ![]() $\hat {f}_\rho$ and
$\hat {f}_\rho$ and ![]() $\hat {f}_\theta$ directly force these modes; and (ii) the ‘indirect route’, where
$\hat {f}_\theta$ directly force these modes; and (ii) the ‘indirect route’, where ![]() $\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ forces a response in wall-normal velocity
$\hat {\boldsymbol{f}}_{\!\!\boldsymbol{u}}^s$ forces a response in wall-normal velocity ![]() $\hat {v}$ through incompressible-like mechanisms and this
$\hat {v}$ through incompressible-like mechanisms and this ![]() $\hat {v}$ in turn forces the supersonic mode. The direct route of forcing appears dominant for a majority of the supersonic modes considered. However, the indirect route plays the dominant role for Mach waves that are forced by the buffer layer of the flow. This distinction is crucial since DNS studies have found that the buffer layer of compressible boundary layers contains the sources that generate the Mach waves found in the free stream (e.g. Duan et al. Reference Duan, Choudhari and Wu2014). We also show that these observations are more generally true for a streamwise developing boundary layer as well.
$\hat {v}$ in turn forces the supersonic mode. The direct route of forcing appears dominant for a majority of the supersonic modes considered. However, the indirect route plays the dominant role for Mach waves that are forced by the buffer layer of the flow. This distinction is crucial since DNS studies have found that the buffer layer of compressible boundary layers contains the sources that generate the Mach waves found in the free stream (e.g. Duan et al. Reference Duan, Choudhari and Wu2014). We also show that these observations are more generally true for a streamwise developing boundary layer as well.
These observations, as well as preliminary discussions regarding the free stream inclination angles of these resolvent modes, show that, in the future, constructing resolvent-based low-rank models of free stream disturbances in the real flow is crucial for further understanding and modelling of these structures. Additionally, considering the role of the solenoidal component of the forcing in exciting the subsonic modes and the supersonic modes from the buffer layer, it would be interesting to see how much of the flow can be modelled by considering this solenoidal component of the forcing alone, instead of the much higher-rank full forcing.
Acknowledgements
We acknowledge support from the Air Force Office of Scientific Research grant FA9550-20-1-0173. We would also like to thank Professor L. Duan for providing us with the 2-D mean profiles used in § 8 and Professor A. Leonard for helpful discussions regarding this work. We are also grateful to the anonymous referees for their valuable suggestions and questions regarding this work.
Declaration of interests
The authors report no conflicts of interest.
Appendix A. Suboptimal modes
So far, we have only considered the leading resolvent mode. For the sake of completeness, in this section, we will briefly consider the effect that the different forcing components have on the suboptimal modes while noting that for a more complete understanding of if, and how many, suboptimal modes should be considered, we will require resolvent-based reconstructions of DNS data. In figure 16, the first 10 resolvent modes, i.e. those corresponding to ![]() $\sigma _1\unicode{x2013} \sigma _{10}$ in (2.8) are shown. Two
$\sigma _1\unicode{x2013} \sigma _{10}$ in (2.8) are shown. Two ![]() $(k_x,k_z)$ pairs are considered: (i) the subsonic mode indicated by the diamond (
$(k_x,k_z)$ pairs are considered: (i) the subsonic mode indicated by the diamond (![]() $\blacklozenge$) in figure 1(
$\blacklozenge$) in figure 1(![]() $b$) is shown in figure 16(
$b$) is shown in figure 16(![]() $a$) and (ii) the supersonic mode MW1 indicated by the square (
$a$) and (ii) the supersonic mode MW1 indicated by the square (![]() $\blacksquare$) in figure 1(
$\blacksquare$) in figure 1(![]() $b$) is shown in figure 16(
$b$) is shown in figure 16(![]() $b$). The Chu norm of the response to the full resolvent forcing is depicted in black, while the response to
$b$). The Chu norm of the response to the full resolvent forcing is depicted in black, while the response to ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ is shown in blue and to
$\hat {\boldsymbol {f}}_{\!\!1}$ is shown in blue and to ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ is shown in red. (The energy of the full response is equal to the sum of the energies of the responses to
$\hat {\boldsymbol {f}}_{\!\!2}$ is shown in red. (The energy of the full response is equal to the sum of the energies of the responses to ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ and
$\hat {\boldsymbol {f}}_{\!\!1}$ and ![]() $\hat {\boldsymbol {f}}_{\!\!2}$, and twice the cross-correlation between these responses. While the energies of the responses to
$\hat {\boldsymbol {f}}_{\!\!2}$, and twice the cross-correlation between these responses. While the energies of the responses to ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ and
$\hat {\boldsymbol {f}}_{\!\!1}$ and ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ are positive, their cross-correlation can be negative. This is why, for certain modes such as for the
$\hat {\boldsymbol {f}}_{\!\!2}$ are positive, their cross-correlation can be negative. This is why, for certain modes such as for the ![]() $N=4$ mode in figure 16(
$N=4$ mode in figure 16(![]() $b$), energy of the response to
$b$), energy of the response to ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ or
$\hat {\boldsymbol {f}}_{\!\!1}$ or ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ is higher than the full response in black.)
$\hat {\boldsymbol {f}}_{\!\!2}$ is higher than the full response in black.)

Figure 16. Chu norm of the response of the resolvent operator to the full resolvent forcing mode ![]() $\boldsymbol {\phi }_i$ (black) as well as the two components of the forcing
$\boldsymbol {\phi }_i$ (black) as well as the two components of the forcing ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ (blue) and
$\hat {\boldsymbol {f}}_{\!\!1}$ (blue) and ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ (red) for the first 10 resolvent modes. (a) Subsonic mode considered in figure 4 and (b) supersonic mode considered in figure 8.
$\hat {\boldsymbol {f}}_{\!\!2}$ (red) for the first 10 resolvent modes. (a) Subsonic mode considered in figure 4 and (b) supersonic mode considered in figure 8.
Considering the response of the subsonic mode in figure 16(![]() $a$), we find that the first three modes are captured by the solenoidal component of the forcing (the red dots are not visible for
$a$), we find that the first three modes are captured by the solenoidal component of the forcing (the red dots are not visible for ![]() $N=1,2$ since they fall below the range of the
$N=1,2$ since they fall below the range of the ![]() $y$-axis shown in the figure). When considering the supersonic mode, we find that the second resolvent mode is captured by the solenoidal component of the forcing. This brings us back to the observation that MW1 exists in a region of the wavenumber space where both incompressible-like and purely compressible mechanisms coexist, and also that incompressible-like mechanisms can excite compressible modes. The compressible mechanisms forced by the direct route are most active for the mode considered here, and therefore appears as the first resolvent mode. For the current work, we leave the discussion of the suboptimal modes at this point, while noting that this topic requires further investigation, especially when constructing resolvent-based low-rank models of the free stream.
$y$-axis shown in the figure). When considering the supersonic mode, we find that the second resolvent mode is captured by the solenoidal component of the forcing. This brings us back to the observation that MW1 exists in a region of the wavenumber space where both incompressible-like and purely compressible mechanisms coexist, and also that incompressible-like mechanisms can excite compressible modes. The compressible mechanisms forced by the direct route are most active for the mode considered here, and therefore appears as the first resolvent mode. For the current work, we leave the discussion of the suboptimal modes at this point, while noting that this topic requires further investigation, especially when constructing resolvent-based low-rank models of the free stream.
Appendix B. Grid convergence
To discretise the equations in (2.1) in the wall-normal direction, we use a summation-by-parts finite difference scheme with ![]() $N=401$ grid points. A grid stretching method is employed to properly resolve the wall-normal direction (Mattsson & Nordström Reference Mattsson and Nordström2004; Kamal et al. Reference Kamal, Rigas, Lakebrink and Colonius2020) (see § 2.4). This grid stretching introduces spurious numerical oscillations in the modes obtained. Following Appelö & Colonius (Reference Appelö and Colonius2009), a damping layer along with an artificial viscosity is used to remedy these spurious oscillations. The role of this damping layer is to slow down the waves within it and to implement it, we use the damping layer and artificial viscosity defined by Appelö & Colonius (Reference Appelö and Colonius2009) (for the damping layer, equation (4) of Appelö & Colonius (Reference Appelö and Colonius2009) with
$N=401$ grid points. A grid stretching method is employed to properly resolve the wall-normal direction (Mattsson & Nordström Reference Mattsson and Nordström2004; Kamal et al. Reference Kamal, Rigas, Lakebrink and Colonius2020) (see § 2.4). This grid stretching introduces spurious numerical oscillations in the modes obtained. Following Appelö & Colonius (Reference Appelö and Colonius2009), a damping layer along with an artificial viscosity is used to remedy these spurious oscillations. The role of this damping layer is to slow down the waves within it and to implement it, we use the damping layer and artificial viscosity defined by Appelö & Colonius (Reference Appelö and Colonius2009) (for the damping layer, equation (4) of Appelö & Colonius (Reference Appelö and Colonius2009) with ![]() $p=4$,
$p=4$, ![]() $q=4$ and
$q=4$ and ![]() $\epsilon _L=10^{-4}$ is used, and for the artificial viscosity, equation (9) from the reference with
$\epsilon _L=10^{-4}$ is used, and for the artificial viscosity, equation (9) from the reference with ![]() $K=[0]$ and
$K=[0]$ and ![]() $\gamma _k=0.15$ is used). It is ensured that at least
$\gamma _k=0.15$ is used). It is ensured that at least ![]() $20$ grid points are included within the damping layer.
$20$ grid points are included within the damping layer.
It is important to consider whether the grid stretching and the introduction of the damping layer impact the conclusions drawn in the current work. To investigate this, in figure 17, the real and imaginary parts of the pressure obtained from the leading resolvent response for the supersonic mode ![]() $\lambda _x=5$,
$\lambda _x=5$, ![]() $\lambda _y=3.5$ and
$\lambda _y=3.5$ and ![]() $c\approx U(y^+=15)$ are shown. Three grids are considered in figure 17: (i) Case 1 (grey solid line), the same grid as that used in this work, but without a damping layer; (ii) Case 2 (red solid line), the grid used in this work with
$c\approx U(y^+=15)$ are shown. Three grids are considered in figure 17: (i) Case 1 (grey solid line), the same grid as that used in this work, but without a damping layer; (ii) Case 2 (red solid line), the grid used in this work with ![]() $N=401$ and
$N=401$ and ![]() $y_{{{max}}}=4\delta$ for the subsonic modes and
$y_{{{max}}}=4\delta$ for the subsonic modes and ![]() $y_{{{max}}}=3l$ for the supersonic modes (where
$y_{{{max}}}=3l$ for the supersonic modes (where ![]() $l$ is the wavelength of the Mach waves); and (iii) Case 3 (blue dashed line), with
$l$ is the wavelength of the Mach waves); and (iii) Case 3 (blue dashed line), with ![]() $N=601$ and
$N=601$ and ![]() $y_{{{max}}}=5\delta$ for the subsonic modes and
$y_{{{max}}}=5\delta$ for the subsonic modes and ![]() $y_{{{max}}}=4l$ for the supersonic modes. The red shaded regions towards the free stream indicate the extent of the damping layer for Case 2, and the blue shaded region indicates the damping layer for Case 3 (since the number of points in the damping layer is fixed to be
$y_{{{max}}}=4l$ for the supersonic modes. The red shaded regions towards the free stream indicate the extent of the damping layer for Case 2, and the blue shaded region indicates the damping layer for Case 3 (since the number of points in the damping layer is fixed to be ![]() $20$, the wall-normal extent of the damping layer changes with
$20$, the wall-normal extent of the damping layer changes with ![]() $N$). To keep the comparison consistent across the three cases, all the responses are set to zero within the red shaded region and beyond into the free stream. Considering the grey lines from Case 1, where no damping layer or artificial viscosity is included, we observe sawtooth oscillations that arise due to the grid stretching. From the modes obtained with damping layers, we note that this damping removes the sawtooth oscillations. Comparing the responses from Case 2 and Case 3, we notice that there are small differences between the modes. This is probably expected given that the extent of the damping layer is different for the two cases. Importantly, these differences do not impact the conclusions drawn in the current work. To see this, in figures 19(
$N$). To keep the comparison consistent across the three cases, all the responses are set to zero within the red shaded region and beyond into the free stream. Considering the grey lines from Case 1, where no damping layer or artificial viscosity is included, we observe sawtooth oscillations that arise due to the grid stretching. From the modes obtained with damping layers, we note that this damping removes the sawtooth oscillations. Comparing the responses from Case 2 and Case 3, we notice that there are small differences between the modes. This is probably expected given that the extent of the damping layer is different for the two cases. Importantly, these differences do not impact the conclusions drawn in the current work. To see this, in figures 19(![]() $a$)–19(
$a$)–19(![]() $c$), we reproduce figures 11(
$c$), we reproduce figures 11(![]() $a$)–11(
$a$)–11(![]() $c$) using a higher number of grid points and a larger
$c$) using a higher number of grid points and a larger ![]() $y_{{{max}}}$. In other words, the data plotted in figures 19(
$y_{{{max}}}$. In other words, the data plotted in figures 19(![]() $a$)–19(
$a$)–19(![]() $c$) are computed using the grid Case 3, while the data in figures 11(
$c$) are computed using the grid Case 3, while the data in figures 11(![]() $a$)–11(
$a$)–11(![]() $c$) were computed using the grid Case 2. We see that there are no significant differences that arise due to an increase in the number of grid points as well as an increase in the maximum extent of the wall-normal grid.
$c$) were computed using the grid Case 2. We see that there are no significant differences that arise due to an increase in the number of grid points as well as an increase in the maximum extent of the wall-normal grid.

Figure 17. Real and imaginary parts of the pressure from the leading resolvent response for a supersonic mode. The mode corresponds to ![]() $\lambda _x=5$,
$\lambda _x=5$, ![]() $\lambda _z=3.5$ and
$\lambda _z=3.5$ and ![]() $c=\bar {U}(y^+\approx 15)$ for a compressible boundary layer over an adiabatic wall with
$c=\bar {U}(y^+\approx 15)$ for a compressible boundary layer over an adiabatic wall with ![]() $Ma=4$ and
$Ma=4$ and ![]() $Re_\tau = 400$ (the mode indicated by the square (
$Re_\tau = 400$ (the mode indicated by the square (![]() $\blacksquare$) in figure 1(b)). The modes obtained using three different grids are shown: (i) Case 1, the grid used in this work but without a damping layer; (ii) Case 2, the grid used in this work with
$\blacksquare$) in figure 1(b)). The modes obtained using three different grids are shown: (i) Case 1, the grid used in this work but without a damping layer; (ii) Case 2, the grid used in this work with ![]() $N=401$,
$N=401$, ![]() $y_{{{max}}}=4\delta$ and
$y_{{{max}}}=4\delta$ and ![]() $y_{{{max}}}=3l$ in the subsonic and supersonic regions, respectively; and (iii) Case 3, with
$y_{{{max}}}=3l$ in the subsonic and supersonic regions, respectively; and (iii) Case 3, with ![]() $N=601$,
$N=601$, ![]() $y_{{{max}}}=5\delta$ and
$y_{{{max}}}=5\delta$ and ![]() $y_{{{max}}}=4l$ in the subsonic and supersonic regions, respectively.
$y_{{{max}}}=4l$ in the subsonic and supersonic regions, respectively.
To investigate the effect of the damping layer a little further, in figure 18, the real part of the pressure obtained using the grid Case 2 is shown, with the strength of the artificial viscosity increasing moving from left to right. We notice that there is a beating in the pressure fluctuations that is made worse as the strength of this artificial viscosity increases. This is likely due to reflections introduced by the artificial viscosity. This shows that we should exercise caution when introducing artificial viscosity (removing the artificial viscosity is not a valid option since it will re-introduce the sawtooth oscillations). However, the results in the current work are not affected by the strength of the artificial viscosity. To illustrate this, in figures 19(![]() $d$)–19(
$d$)–19(![]() $f$), we reproduce figures 11(
$f$), we reproduce figures 11(![]() $a$)–11(
$a$)–11(![]() $c$) with a grid that uses a stronger artificial viscosity (the strength is determined by the value of
$c$) with a grid that uses a stronger artificial viscosity (the strength is determined by the value of ![]() $\gamma _k$, which increases from
$\gamma _k$, which increases from ![]() $0.15$ in figures 11(
$0.15$ in figures 11(![]() $a$)–11(
$a$)–11(![]() $c$) to
$c$) to ![]() $1.5$ in figures 19(
$1.5$ in figures 19(![]() $d$)–19(
$d$)–19(![]() $f$). There are no significant differences introduced by this change in the strength of the damping layer.
$f$). There are no significant differences introduced by this change in the strength of the damping layer.

Figure 18. Pressure fluctuations obtained from the leading resolvent response for a supersonic mode with the red and blue contours representing positive and negative pressure fluctuations, respectively. The mode corresponds to ![]() $\lambda _x=5$,
$\lambda _x=5$, ![]() $\lambda _z=3.5$ and
$\lambda _z=3.5$ and ![]() $c=\bar {U}(y^+\approx 15)$ for a compressible boundary layer over an adiabatic wall with
$c=\bar {U}(y^+\approx 15)$ for a compressible boundary layer over an adiabatic wall with ![]() $Ma=4$ and
$Ma=4$ and ![]() $Re_\tau = 400$ (the mode indicated by the square (
$Re_\tau = 400$ (the mode indicated by the square (![]() $\blacksquare$) in figure 1b). The strength of the damping layer used increases from (a)
$\blacksquare$) in figure 1b). The strength of the damping layer used increases from (a) ![]() $0.15$ to (b)
$0.15$ to (b) ![]() $0.5$ and then to (c)
$0.5$ and then to (c) ![]() $1.5$.
$1.5$.

Figure 19. Chu norm of the response of the resolvent operator to (a,d) the full leading resolvent forcing mode ![]() $\boldsymbol {\phi }_1$ as well as the two components of the forcing (b,e)
$\boldsymbol {\phi }_1$ as well as the two components of the forcing (b,e) ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ and (c,f)
$\hat {\boldsymbol {f}}_{\!\!1}$ and (c,f) ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ as a function of the streamwise (
$\hat {\boldsymbol {f}}_{\!\!2}$ as a function of the streamwise (![]() $\lambda _x$) and spanwise (
$\lambda _x$) and spanwise (![]() $\lambda _z$) wavelengths for a fixed value of phase speed
$\lambda _z$) wavelengths for a fixed value of phase speed ![]() $c=\bar {U}(y^+\approx 15)$. The
$c=\bar {U}(y^+\approx 15)$. The ![]() $Ma=4$,
$Ma=4$, ![]() $Re_\tau =400$ turbulent boundary layer over an adiabatic wall (that was also considered in figure 11) is shown. (a–c) Responses obtained using
$Re_\tau =400$ turbulent boundary layer over an adiabatic wall (that was also considered in figure 11) is shown. (a–c) Responses obtained using ![]() $N=601$ grid points and a
$N=601$ grid points and a ![]() $y_{{{max}}} = 5\delta$ in the subsonic region and
$y_{{{max}}} = 5\delta$ in the subsonic region and ![]() $y_{{{max}}} = 4l$ in the supersonic region. (d–f) Responses obtained with a grid where the strength of the damping layer is increased to
$y_{{{max}}} = 4l$ in the supersonic region. (d–f) Responses obtained with a grid where the strength of the damping layer is increased to ![]() $\gamma _k=1.5$ (from the
$\gamma _k=1.5$ (from the ![]() $\gamma _k=0.15$ used for figure 11). These responses are compared with those shown in figures 11(
$\gamma _k=0.15$ used for figure 11). These responses are compared with those shown in figures 11(![]() $a$)–11(
$a$)–11(![]() $c$) to illustrate the insensitivity of the obtained results to the grid used. The dashed lines in all figures indicate the relative Mach number equal to unity line.
$c$) to illustrate the insensitivity of the obtained results to the grid used. The dashed lines in all figures indicate the relative Mach number equal to unity line.
The main conclusions drawn in the current work are therefore not significantly impacted by changes in the grid used.
Appendix C. Laminar boundary layer
We will also briefly consider the case of a laminar compressible boundary layer and show that the trends discussed here are more generally applicable to compressible laminar boundary layers as well. The Reynolds number for the laminar case is defined as ![]() $Re=U_\infty l/\nu _\infty$, where the reference length scale is defined as
$Re=U_\infty l/\nu _\infty$, where the reference length scale is defined as ![]() $l=(x\nu _\infty /U_\infty )^{1/2}$. The mean streamwise velocity
$l=(x\nu _\infty /U_\infty )^{1/2}$. The mean streamwise velocity ![]() $\bar {U}$ and temperature
$\bar {U}$ and temperature ![]() $\bar {T}$ profiles for the compressible laminar boundary layers that are required as input to the model are obtained from the Mangler–Levy–Lees similarity profiles as done by Malik (Reference Malik1990). In figures 20(
$\bar {T}$ profiles for the compressible laminar boundary layers that are required as input to the model are obtained from the Mangler–Levy–Lees similarity profiles as done by Malik (Reference Malik1990). In figures 20(![]() $a$)–20(
$a$)–20(![]() $c$), we reproduce figures 11(
$c$), we reproduce figures 11(![]() $a$)–11(
$a$)–11(![]() $c$) for the case of an
$c$) for the case of an ![]() $Ma=8$ and
$Ma=8$ and ![]() $Re=1000$ laminar compressible boundary layer. We see that, similar to the case of the turbulent flow,
$Re=1000$ laminar compressible boundary layer. We see that, similar to the case of the turbulent flow, ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ and
$\hat {\boldsymbol {f}}_{\!\!1}$ and ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ approximately capture the subsonic and supersonic regions, separately.
$\hat {\boldsymbol {f}}_{\!\!2}$ approximately capture the subsonic and supersonic regions, separately.

Figure 20. Chu norm of the response of the resolvent operator to (a) the full leading resolvent forcing mode ![]() $\boldsymbol {\phi }_1$ as well as the two components of the forcing (b)
$\boldsymbol {\phi }_1$ as well as the two components of the forcing (b) ![]() $\hat {\boldsymbol {f}}_{\!\!1}$ and (c)
$\hat {\boldsymbol {f}}_{\!\!1}$ and (c) ![]() $\hat {\boldsymbol {f}}_{\!\!2}$ as a function of the streamwise (
$\hat {\boldsymbol {f}}_{\!\!2}$ as a function of the streamwise (![]() $\lambda _x$) and spanwise (
$\lambda _x$) and spanwise (![]() $\lambda _z$) wavelengths for a fixed value of phase speed
$\lambda _z$) wavelengths for a fixed value of phase speed ![]() $c=0.5$. The
$c=0.5$. The ![]() $Ma=8$,
$Ma=8$, ![]() $Re=1000$ laminar boundary layer over an adiabatic wall is considered. This figure is equivalent to figure 11 for the case of a laminar flow. The dashed lines indicate the relative Mach number equal to unity line.
$Re=1000$ laminar boundary layer over an adiabatic wall is considered. This figure is equivalent to figure 11 for the case of a laminar flow. The dashed lines indicate the relative Mach number equal to unity line.