Published online by Cambridge University Press: 03 May 2018
Solid-state convection can take place in the rocky or icy mantles of planetary objects, and these mantles can be surrounded above or below or both by molten layers of similar composition. A flow towards the interface can proceed through it by changing phase. This behaviour is modelled by a boundary condition taking into account the competition between viscous stress in the solid, which builds topography of the interface with a time scale
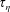 $\unicode[STIX]{x1D70F}_{\unicode[STIX]{x1D702}}$
, and convective transfer of the latent heat in the liquid from places of the boundary where freezing occurs to places of melting, which acts to erase topography, with a time scale
$\unicode[STIX]{x1D70F}_{\unicode[STIX]{x1D702}}$
, and convective transfer of the latent heat in the liquid from places of the boundary where freezing occurs to places of melting, which acts to erase topography, with a time scale
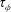 $\unicode[STIX]{x1D70F}_{\unicode[STIX]{x1D719}}$
. The ratio
$\unicode[STIX]{x1D70F}_{\unicode[STIX]{x1D719}}$
. The ratio
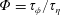 $\unicode[STIX]{x1D6F7}=\unicode[STIX]{x1D70F}_{\unicode[STIX]{x1D719}}/\unicode[STIX]{x1D70F}_{\unicode[STIX]{x1D702}}$
controls whether the boundary condition is the classical non-penetrative one (
$\unicode[STIX]{x1D6F7}=\unicode[STIX]{x1D70F}_{\unicode[STIX]{x1D719}}/\unicode[STIX]{x1D70F}_{\unicode[STIX]{x1D702}}$
controls whether the boundary condition is the classical non-penetrative one (
 $\unicode[STIX]{x1D6F7}\rightarrow \infty$
) or allows for a finite flow through the boundary (small
$\unicode[STIX]{x1D6F7}\rightarrow \infty$
) or allows for a finite flow through the boundary (small
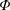 $\unicode[STIX]{x1D6F7}$
). We study Rayleigh–Bénard convection in a plane layer subject to this boundary condition at either or both its boundaries using linear and weakly nonlinear analyses. When both boundaries are phase-change interfaces with equal values of
$\unicode[STIX]{x1D6F7}$
). We study Rayleigh–Bénard convection in a plane layer subject to this boundary condition at either or both its boundaries using linear and weakly nonlinear analyses. When both boundaries are phase-change interfaces with equal values of
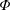 $\unicode[STIX]{x1D6F7}$
, a non-deforming translation mode is possible with a critical Rayleigh number equal to
$\unicode[STIX]{x1D6F7}$
, a non-deforming translation mode is possible with a critical Rayleigh number equal to
 $24\unicode[STIX]{x1D6F7}$
. At small values of
$24\unicode[STIX]{x1D6F7}$
. At small values of
 $\unicode[STIX]{x1D6F7}$
, this mode competes with a weakly deforming mode having a slightly lower critical Rayleigh number and a very long wavelength,
$\unicode[STIX]{x1D6F7}$
, this mode competes with a weakly deforming mode having a slightly lower critical Rayleigh number and a very long wavelength,
 $\unicode[STIX]{x1D706}_{c}\sim 8\sqrt{2}\unicode[STIX]{x03C0}/3\sqrt{\unicode[STIX]{x1D6F7}}$
. Both modes lead to very efficient heat transfer, as expressed by the relationship between the Nusselt and Rayleigh numbers. When only one boundary is subject to a phase-change condition, the critical Rayleigh number is
$\unicode[STIX]{x1D706}_{c}\sim 8\sqrt{2}\unicode[STIX]{x03C0}/3\sqrt{\unicode[STIX]{x1D6F7}}$
. Both modes lead to very efficient heat transfer, as expressed by the relationship between the Nusselt and Rayleigh numbers. When only one boundary is subject to a phase-change condition, the critical Rayleigh number is
 $\mathit{Ra}_{c}=153$
and the critical wavelength is
$\mathit{Ra}_{c}=153$
and the critical wavelength is
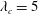 $\unicode[STIX]{x1D706}_{c}=5$
. The Nusselt number increases approximately two times faster with the Rayleigh number than in the classical case with non-penetrative conditions, and the average temperature diverges from
$\unicode[STIX]{x1D706}_{c}=5$
. The Nusselt number increases approximately two times faster with the Rayleigh number than in the classical case with non-penetrative conditions, and the average temperature diverges from
 $1/2$
when the Rayleigh number is increased, towards larger values when the bottom boundary is a phase-change interface.
$1/2$
when the Rayleigh number is increased, towards larger values when the bottom boundary is a phase-change interface.