Published online by Cambridge University Press: 23 April 2021
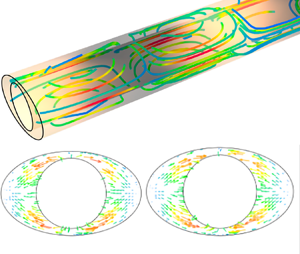
The two-dimensional laminar flow of a viscous fluid induced by peristalsis due to a moving wall wave has been studied previously for a rectangular channel, a circular tube and a concentric circular annulus. Here, we study peristaltic flow in a non-axisymmetric annular tube: in this case, the flow is three-dimensional, with motions in the azimuthal direction. This type of geometry is motivated by experimental observations of the pulsatile flow of cerebrospinal fluid along perivascular spaces surrounding arteries in the brain, which is at least partially driven by peristaltic pumping due to pulsations of the artery. These annular perivascular spaces are often eccentric and the outer boundary is seldom circular: their cross-sections can be well matched by a simple, adjustable model consisting of an inner circle (the outer wall of the artery) and an outer ellipse (the outer edge of the perivascular space), not necessarily concentric. We use this geometric model as a basis for numerical simulations of peristaltic flow: the adjustability of the model makes it suitable for other applications. We concentrate on the general effects of the non-axisymmetric configuration on the flow and do not attempt to specifically model perivascular pumping. We use a finite-element scheme to compute the flow in the annulus driven by a propagating sinusoidal radial displacement of the inner wall. Unlike the peristaltic flow in a concentric circular annulus, the flow is fully three-dimensional: azimuthal pressure variations drive an oscillatory flow in and out of the narrower gaps, inducing an azimuthal wiggle in the streamlines. We examine the dependence of the flow on the elongation of the outer elliptical wall and the eccentricity of the configuration. We find that the time-averaged volumetric flow is always in the same direction as the peristaltic wave and decreases with increasing ellipticity or eccentricity. The additional shearing motion in the azimuthal direction will increase mixing and enhance Taylor dispersion in these flows, effects that might have practical applications.