Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-31T22:08:23.212Z Has data issue: false hasContentIssue false
  • English
  • Français

Onset of thermoacoustic instability in turbulent combustors: an emergence of synchronized periodicity through formation of chimera-like states

Published online by Cambridge University Press:  15 December 2016

Sirshendu Mondal ,
Vishnu R. Unni  and
R. I. Sujith
Sirshendu Mondal*
Affiliation:
Department of Aerospace Engineering, Indian Institute of Technology Madras, Chennai, 600036, India
Vishnu R. Unni
Affiliation:
Department of Aerospace Engineering, Indian Institute of Technology Madras, Chennai, 600036, India
R. I. Sujith
Affiliation:
Department of Aerospace Engineering, Indian Institute of Technology Madras, Chennai, 600036, India
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Thermoacoustic systems with a turbulent reactive flow, prevalent in the fields of power and propulsion, are highly susceptible to oscillatory instabilities. Recent studies showed that such systems transition from combustion noise to thermoacoustic instability through a dynamical state known as intermittency, where bursts of large-amplitude periodic oscillations appear in a near-random fashion in between regions of low-amplitude aperiodic fluctuations. However, as these analyses were in the temporal domain, this transition remains still unexplored spatiotemporally. Here, we present the spatiotemporal dynamics during the transition from combustion noise to limit cycle oscillations in a turbulent bluff-body stabilized combustor. 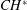To that end, we acquire the pressure oscillations and the field of heat release rate oscillations through high-speed chemiluminescence ($CH^{\ast }$) images of the reaction zone. With a view to get an insight into this complex dynamics, we compute the instantaneous phases between acoustic pressure and local heat release rate oscillations. We observe that the aperiodic oscillations during combustion noise are phase asynchronous, while the large-amplitude periodic oscillations seen during thermoacoustic instability are phase synchronous. We find something interesting during intermittency: patches of synchronized periodic oscillations and desynchronized aperiodic oscillations coexist in the reaction zone. In other words, the emergence of order from disorder happens through a dynamical state wherein regions of order and disorder coexist, resembling a chimera state. Generally, mutually coupled chaotic oscillators synchronize but retain their dynamical nature; the same is true for coupled periodic oscillators. In contrast, during intermittency, we find that patches of desynchronized aperiodic oscillations turn into patches of synchronized periodic oscillations and vice versa. Therefore, the dynamics of local heat release rate oscillations change from aperiodic to periodic as they synchronize intermittently. The temporal variations in global synchrony, estimated through the Kuramoto order parameter, echoes the breathing nature of a chimera state.

JFM classification

Nonlinear Dynamical Systems: Nonlinear Dynamical Systems Instability: Nonlinear instability Reacting Flows: Turbulent reacting flows
Type
Papers
Information
Journal of Fluid Mechanics , Volume 811 , 25 January 2017 , pp. 659 - 681
Check for updates
Copyright
© 2016 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abrams, D. M., Mirollo, R., Strogatz, S. H. & Wiley, D. A. 2008 Solvable model for chimera states of coupled oscillators. Phys. Rev. Lett. 101 (8), 084103.Google ScholarPubMed
Abrams, D. M. & Strogatz, S. H. 2004 Chimera states for coupled oscillators. Phys. Rev. Lett. 93 (17), 174102.CrossRefGoogle ScholarPubMed
Abugov, D. I. & Obrezkov, O. I. 1978 Acoustic noise in turbulent flames. Combust. Explos. Shock Waves 14 (5), 606–612.CrossRefGoogle Scholar
Akkerman, V. & Law, C. K. 2013 Effect of acoustic coupling on power-law flame acceleration in spherical confinement. Phys. Fluids 25 (1), 013602.CrossRefGoogle Scholar
Akkerman, V. & Law, C. K. 2016 Coupling of harmonic flow oscillations to combustion instability in premixed segments of triple flames. Combust. Flame 172, 342–348.CrossRefGoogle Scholar
Akkerman, V., Law, C. K. & Bychkov, V. 2011 Self-similar accelerative propagation of expanding wrinkled flames and explosion triggering. Phys. Rev. E 83 (2), 026305.CrossRefGoogle ScholarPubMed
Arumugam, E. M. E. & Spano, M. L. 2015 A chimeric path to neuronal synchronization. Chaos: An Interdiscipl. J. Nonlinear Sci. 25 (1), 013107.Google Scholar
Balasubramanian, K. & Sujith, R. I. 2008 Non-normality and nonlinearity in combustion–acoustic interaction in diffusion flames. J. Fluid Mech. 594, 29–57.CrossRefGoogle Scholar
Blasius, B., Amit, H. & Lewi, S. 1999 Complex dynamics and phase synchronization in spatially extended ecological systems. Nature 399, 354–359.CrossRefGoogle ScholarPubMed
Boccaletti, S., Kurths, J., Osipov, G., Valladares, D. L. & Zhou, C. S. 2002 The synchronization of chaotic systems. Phys. Rep. 366 (1), 1–101.CrossRefGoogle Scholar
Bychkov, V. 1999 Analytical scalings for flame interaction with sound waves. Phys. Fluids 11 (10), 3168–3173.CrossRefGoogle Scholar
Chaté, H. & Manneville, P. 1987 Transition to turbulence via spatiotemporal intermittency. Phys. Rev. Lett. 58 (2), 112–115.CrossRefGoogle Scholar
Culick, F. E. C. 1987 A note on Rayleigh’s criterion. Combust. Sci. Technol. 56 (4–6), 159–166.CrossRefGoogle Scholar
Domen, S., Gotoda, H., Kuriyama, T., Okuno, Y. & Tachibana, S. 2015 Detection and prevention of blowout in a lean premixed gas-turbine model combustor using the concept of dynamical system theory. Proc. Combust. Inst. 35 (3), 3245–3253.CrossRefGoogle Scholar
Emerson, B., Lieuwen, T. & Juniper, M. P. 2016 Local stability analysis and eigenvalue sensitivity of reacting bluff-body wakes. J. Fluid Mech. 788, 549–575.CrossRefGoogle Scholar
Emerson, B., O’Connor, J., Juniper, M. & Lieuwen, T. 2012 Density ratio effects on reacting bluff-body flow field characteristics. J. Fluid Mech. 706, 219–250.CrossRefGoogle Scholar
Fisher, S. C. & Rahman, S. A.2009 Remembering the giants: Apollo rocket propulsion development. NASA/SP-2009-4545.Google Scholar
Gotoda, H., Shinoda, Y., Kobayashi, M. & Okuno, Y. 2014 Detection and control of combustion instability based on the concept of dynamical system theory. Phy. Rev. E 89 (2), 022910.Google ScholarPubMed
Guethe, F., Guyot, D., Singla, G., Noiray, N. & Schuermans, B. 2012 Chemiluminescence as diagnostic tool in the development of gas turbines. Appl. Phys. B 107 (3), 619–636.CrossRefGoogle Scholar
Hardalupas, Y. & Orain, M. 2004 Local measurements of the time-dependent heat release rate and equivalence ratio using chemiluminescent emission from a flame. Combust. Flame 139 (3), 188–207.CrossRefGoogle Scholar
Hubbard, S. & Dowling, A. P.1998 Acoustic instabilities in premix burners. AIAA Paper 98-2272.CrossRefGoogle Scholar
Kabiraj, L. & Sujith, R. I. 2012 Nonlinear self-excited thermoacoustic oscillations: intermittency and flame blowout. J. Fluid Mech. 713, 376–397.CrossRefGoogle Scholar
Keller, J. O., Vaneveld, L., Korschelt, D., Hubbard, G. L., Ghoniem, A. F., Daily, J. W. & Oppenheim, A. K. 1982 Mechanism of instabilities in turbulent combustion leading to flashback. AIAA J. 20 (2), 254–262.CrossRefGoogle Scholar
Kiss, I. Z., Vilmos, G. & John, L. H. 2000 Experiments on synchronization and control of chaos on coupled electrochemical oscillators. J. Phys. Chem. B 104, 7554–7560.CrossRefGoogle Scholar
Komarek, T. & Polifke, W. 2010 Impact of swirl fluctuations on the flame response of a perfectly premixed swirl burner. Trans. ASME J. Engng Gas Turbines Power 132 (6), 061503.CrossRefGoogle Scholar
Kuramoto, Y. 2012 Chemical Oscillations, Waves, and Turbulence, vol. 19. Springer Science & Business Media.Google Scholar
Kuramoto, Y. & Battogtokh, D. 2002 Coexistence of coherence and incoherence in nonlocally coupled phase oscillators. Nonlinear Phenom. Complex Syst. 5 (4), 380–385.Google Scholar
Leon, G. 2001 Synchronization and rhythmic processes in physiology. Nature 410, 277–284.Google Scholar
Lieuwen, T. C. 2001 Phase drift characteristics of self-excited, combustion-driven oscillations. J. Sound Vib. 242 (5), 893–905.CrossRefGoogle Scholar
Lieuwen, T. C. 2012 Unsteady Combustor Physics. Cambridge University Press.CrossRefGoogle Scholar
Lieuwen, T. C. 2002 Experimental investigation of limit-cycle oscillations in an unstable gas turbine combustor. J. Propul. Power 18 (1), 61–67.CrossRefGoogle Scholar
Lieuwen, T. C. & Yang, V. 2005 Combustion instabilities in gas turbine engines (operational experience, fundamental mechanisms and modeling). Prog. Astronaut. Aeronaut. 210.Google Scholar
Lieuwen, T. & Zinn, B. T. 2000 Application of multipole expansions to sound generation from ducted unsteady combustion processes. J. Sound Vib. 235 (3), 405–414.CrossRefGoogle Scholar
Madjarova, V., Kadono, H. & Toyooka, S. 2003 Dynamic electronic speckle pattern interferometry (DESPI) phase analyses with temporal Hilbert transform. Opt. Express 11 (6), 617–623.CrossRefGoogle ScholarPubMed
Nair, V.2014 Role of intermittency in the onset of combustion instability, PhD thesis, IIT Madras, India.Google Scholar
Nair, V. & Sujith, R. I. 2014 Multifractality in combustion noise: predicting an impending instability. J. Fluid Mech. 747, 635–655.CrossRefGoogle Scholar
Nair, V. & Sujith, R. I. 2016 Precursors to self-sustained oscillations in aeroacoustic systems. Intl J. Aeroacoust. 15 (3), 312–323.CrossRefGoogle Scholar
Nair, V., Thampi, G., Karuppusamy, S., Gopalan, S. & Sujith, R. 2013 Loss of chaos in combustion noise as a precursor of impending combustion instability. Intl J. Spray Combust. Dyn. 5 (4), 273–290.CrossRefGoogle Scholar
Nair, V., Thampi, G. & Sujith, R. I. 2014 Intermittency route to thermoacoustic instability in turbulent combustors. J. Fluid Mech. 756, 470–487.CrossRefGoogle Scholar
Noiray, N. & Schuermans, B. 2013 Deterministic quantities characterizing noise driven Hopf bifurcations in gas turbine combustors. Intl J. Non-Linear Mech. 50, 152–163.CrossRefGoogle Scholar
Panaggio, M. J. & Abrams, D. M. 2015 Chimera states: coexistence of coherence and incoherence in networks of coupled oscillators. Nonlinearity 28 (3), R67–R87.Google Scholar
Pikovsky, A., Rosenblum, M. & Kurths, J. 2003 Synchronization: A Universal Concept in Nonlinear Sciences, vol. 12. Cambridge University Press.Google Scholar
Poinsot, T. J., Trouve, A. C., Veynante, D. P., Candel, S. M. & Esposito, E. J. 1987 Vortex-driven acoustically coupled combustion instabilities. J. Fluid Mech. 177, 265–292.CrossRefGoogle Scholar
Romano, M. C., Thiel, M., Kurths, J., Kiss, I. Z. & Hudson, J. L. 2005 Detection of synchronization for non-phase-coherent and non-stationary data. Europhys. Lett. 71 (3), 466–472.CrossRefGoogle Scholar
Sattelmayer, T. 2003 Influence of the combustor aerodynamics on combustion instabilities from equivalence ratio fluctuations. Trans. ASME J. Engng Gas Turbines Power 125 (1), 11–19.CrossRefGoogle Scholar
Searby, G. 1992 Acoustic instability in premixed flames. Combust. Sci. Technol. 81 (4–6), 221–231.CrossRefGoogle Scholar
Searby, G. & Rochwerger, D. 1991 A parametric acoustic instability in premixed flames. J. Fluid Mech. 231, 529–543.CrossRefGoogle Scholar
Sethares, W. A. 2007 Rhythm and Transforms. Springer Science & Business Media.Google Scholar
Shanbhogue, S., Shin, D. H., Hemchandra, S., Plaks, D. & Lieuwen, T. 2009a Flame-sheet dynamics of bluff-body stabilized flames during longitudinal acoustic forcing. Proc. Combust. Inst. 32 (2), 1787–1794.CrossRefGoogle Scholar
Shanbhogue, S. J., Seelhorst, M. & Lieuwen, T. 2009b Vortex phase-jitter in acoustically excited bluff body flames. Intl J. Spray Combust. Dynam. 1 (3), 365–387.CrossRefGoogle Scholar
Shraiman, B. I. 1986 Order, disorder, and phase turbulence. Phys. Rev. Lett. 57, 325–328.CrossRefGoogle ScholarPubMed
Smith, D. A.1985 Experimental study of acoustically excited, vortex driven, combustion instability within a rearward facing step combustor. PhD thesis, California Inst. Tech., Pasadena, USA.Google Scholar
Strahle, W. 1978 Combustion noise. Prog. Energy Combust. Sci. 4 (3), 157–176.CrossRefGoogle Scholar
Suresha, S., Sujith, R. I., Emerson, B. & Lieuwen, T. 2016 Nonlinear dynamics and intermittency in a turbulent reacting wake with density ratio as bifurcation parameter. Phys. Rev. E 94, 042206.Google Scholar
Thumuluru, S. K. & Lieuwen, T. 2009 Characterization of acoustically forced swirl flame dynamics. Proc. Combust. Inst. 32 (2), 2893–2900.CrossRefGoogle Scholar
Tony, J., Gopalakrishnan, E. A., Sreelekha, E. & Sujith, R. I. 2015 Detecting deterministic nature of pressure measurements from a turbulent combustor. Phys. Rev. E 92 (6), 062902.CrossRefGoogle ScholarPubMed
Trickey, S. T., Virgin, L. N. & Dowell, E. H. 2002 The stability of limit–cycle oscillations in a nonlinear aeroelastic system. Proc. Math. Phys. Engng Sci. 458 (2025), 2203–2226.CrossRefGoogle Scholar
Tyson, J. J. 1994 What everyone should know about the Belousov–Zhabotinsky reaction. In Frontiers in Mathematical Biology, pp. 569–587. Springer.CrossRefGoogle Scholar
Unni, V. R. & Sujith, R. I. 2015 Multifractal characteristics of combustor dynamics close to lean blowout. J. Fluid Mech. 784, 30–50.CrossRefGoogle Scholar
Venkatramani, J., Nair, V., Sujith, R. I., Gupta, S. & Sarkar, S. 2016 Precursors to flutter instability by an intermittency route: a model free approach. J. Fluids Struct. 61, 376–391.CrossRefGoogle Scholar
Wilhite, J. M., Dolan, B. J., Gomez, R. V., Kabiraj, L., Paschereit, C. O. & Gutmark, E.2016 Analysis of combustion oscillations in a staged MLDI burner using decomposition methods and recurrence analysis. AIAA Paper 2016-1156.CrossRefGoogle Scholar
Winfree, A. T. 2001 The Geometry of Biological Time, vol. 12. Springer Science & Business Media.CrossRefGoogle Scholar
Wu, X. & Law, C. K. 2009 Flame-acoustic resonance initiated by vortical disturbances. J. Fluid Mech. 634, 321–357.CrossRefGoogle Scholar
Zukoski, E. E. & Smith, D. A.1985 Combustion instability sustained by unsteady vortex combustion. In AIAA, SA Propulsion Conference, Monterey, CA.CrossRefGoogle Scholar