Article contents
On the flow separation mechanism in the inverse Leidenfrost regime
Published online by Cambridge University Press: 09 June 2020
Abstract
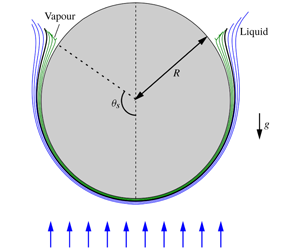
The inverse Leidenfrost regime occurs when a heated object in relative motion with a liquid is surrounded by a stable vapour layer, drastically reducing the hydrodynamic drag at large Reynolds numbers due to a delayed separation of the flow. To elucidate the physical mechanisms that control separation, here we report a numerical study of the boundary layer equations describing the liquid–vapour flow around a solid sphere whose surface temperature is above the Leidenfrost point. Our analysis reveals that the dynamics of the thin layer of vaporised liquid controls the downstream evolution of the flow, which cannot be properly described substituting the vapour layer by an effective slip length. In particular, the dominant mechanism responsible for the separation of the flow is the onset of vapour recirculation caused by the adverse pressure gradient in the rearward half of the sphere, leading to an explosive growth of the vapour-layer thickness due to the accumulation of vapour mass. Buoyancy forces are shown to have an important effect on the onset of recirculation, and thus on the separation angle. Our results compare favourably with previous experiments.
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2020. Published by Cambridge University Press
References
- 9
- Cited by


