Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-20T17:32:50.118Z Has data issue: false hasContentIssue false
  • English
  • Français

Motion of a capsule in a curved tube

Published online by Cambridge University Press:  25 November 2020

Saman Ebrahimi ,
Peter Balogh  and
Prosenjit Bagchi Open the ORCID record for Prosenjit Bagchi [Opens in a new window]
Saman Ebrahimi
Affiliation:
Mechanical and Aerospace Engineering Department, Rutgers, The State University of New Jersey, Piscataway, NJ08854, USA
Peter Balogh
Affiliation:
Mechanical and Aerospace Engineering Department, Rutgers, The State University of New Jersey, Piscataway, NJ08854, USA
Prosenjit Bagchi*
Affiliation:
Mechanical and Aerospace Engineering Department, Rutgers, The State University of New Jersey, Piscataway, NJ08854, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

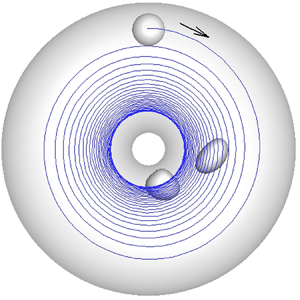
Cross-streamline migration of deformable capsules is studied in three-dimensional curved vessels using a numerical model. Two geometries are chosen: torus vessels to study the effect of constant vessel curvature, and U-shaped vessels to study the effect of change in curvature. A wide range of inertia is considered to include a broad range of applications, from the microcirculation to inertial microfluidics. Vessel curvature and change in curvature are shown to affect capsule migration because of the way they affect the undisturbed flow. In toroidal vessels at negligible inertia, no secondary flow exists and the axial fluid velocity is shifted toward the inner surface of the vessel. In this limit, capsules settle at a location that is away from the vessel centreline, and between the location of the maximum fluid velocity and the innermost surface. Increasing the vessel curvature results in the equilibrium position being increasingly closer to the inner surface. The results suggest the presence of a curvature-induced migration that drives the capsule continuously toward the region of higher streamline curvature. The equilibrium locations are on the symmetry plane of the torus, and are stable equilibria when inertia is negligible. At finite inertia, capsules released on the symmetry plane first settle at an equilibrium located on this plane, which may either be stable or unstable. This equilibrium location depends on both capsule deformability (characterised by capillary number, $Ca$) and the tube Reynolds number $R{e_t}$. It is closer to the inner edge of the torus for smaller $R{e_t}$ and larger $Ca$, and toward the outer edge for larger $R{e_t}$ and smaller $Ca$. This dependence of the equilibria arises due to the secondary flow (or Dean's vortex), which opposes the curvature-induced inward migration. The equilibrium locations on the symmetry plane are unstable if the secondary flow is strong, in which case capsules depart the symmetry plane, and set on to a spiralling motion, eventually settling near the centres of the Dean's vortices. For the U-shaped vessels, the curvature change is shown to create a radial velocity component, even at small inertia, that can be of comparable magnitude to the axial velocity, and that increases with increasing curvature. This radial velocity causes a large and abrupt change in capsule trajectory toward the inner side of the vessel where the curvature increases, but toward the outer side where the curvature decreases.

JFM classification

Biological Fluid Dynamics: Capsule/cell dynamics
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 907 , 25 January 2021 , A28
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alghalibi, D., Rosti, M. E. & Brandt, L. 2019 Inertial migration of a deformable particle in pipe flow. Phys. Rev. Fluids 4, 104201.CrossRefGoogle Scholar
Aouane, O., Thiébaud, M., Benyoussef, A., Wagner, C. & Misbah, C. 2014 Vesicle dynamics in a confined Poiseuille flow: from steady state to chaos. Phys. Rev. E 90, 033011.CrossRefGoogle Scholar
Asmolov, E. S. 1999 The inertial lift on a spherical particle in a plane Poiseuille flow at large channel Reynolds number. J. Fluid Mech. 381, 63–87.CrossRefGoogle Scholar
Aubert, J. H. & Tirrell, M. 1980 Macromolecules in nonhomogeneous velocity gradient fields. J. Chem. Phys. 72, 2694–2701.CrossRefGoogle Scholar
Balogh, P. & Bagchi, P. 2017 A computational approach to modeling cellular-scale blood flow in complex geometry. J. Comput. Phys. 334, 280–307.CrossRefGoogle Scholar
Balogh, P. & Bagchi, P. 2019 The cell-free layer in simulated microvascular networks. J. Fluid Mech. 864, 768–806.CrossRefGoogle Scholar
Bhagat, A. A. S., Kuntaegowdanahalli, S. S. & Papautsky, I. 2008 Continuous particle separation in spiral microchannels using dean flows and differential migration. Lab on a Chip 8, 1906–1914.CrossRefGoogle ScholarPubMed
Blinder, P., Tsai, P. S., Kaufhold, J. P., Knutsen, P. M., Suhl, H. & Kleinfeld, D. 2013 The cortical angiome: an interconnected vascular network with noncolumnar patterns of blood flow. Nat. Neurosci. 16, 889–897.CrossRefGoogle ScholarPubMed
Chadwick, R. 1985 Slow viscous flow inside a torus – the resistance of small tortuous blood vessels. Q. Appl. Maths 43, 317–323.CrossRefGoogle Scholar
Chan, P. C.-H. & Leal, L. G. 1979 The motion of a deformable drop in a second-order fluid. J. Fluid Mech. 92, 131–170.CrossRefGoogle Scholar
Chan, P. C.-H. & Leal, L. G. 1981 An experimental study of drop migration in shear flow between concentric cylinders. Intl J. Multiphase Flow 7, 83–94.CrossRefGoogle Scholar
Cheung, A. T. W., Chen, P. C. Y., Larkin, E. C., Duong, P. L., Ramanujan, S., Tablin, F. & Wun, T. 2002 Microvascular abnormalities in sickle cell disease: a computer-assisted intravital microscopy study. Blood 99, 3999–4005.CrossRefGoogle ScholarPubMed
Cheung, C. Y., Zheng, Y., Hsu, W., Lee, M. L., Lau, Q. P., Mitchell, P., Wang, J. J., Klein, R. & Wong, T. Y. 2011 Retinal vascular tortuosity, blood pressure, and cardiovascular risk factors. Ophthalmology 118, 812–818.CrossRefGoogle ScholarPubMed
Coupier, G., Kaoui, B., Podgorski, T. & Misbah, C. 2008 Noninertial lateral migration of vesicles in bounded Poiseuille flow. Phys. Fluids 20, 111702.CrossRefGoogle Scholar
Crowl, L. M. & Fogelson, A. L. 2011 Analysis of mechanisms for platelet near-wall excess under arterial blood flow conditions. J. Fluid Mech. 676, 348–375.CrossRefGoogle Scholar
Danker, G., Vlahovska, P. M. & Misbah, C. 2009 Vesicles in Poiseuille flow. Phys. Rev. Lett. 102, 148102.CrossRefGoogle ScholarPubMed
Dean, W. R. 1928 The stream-line motion of fluid in a curved pipe. Phil. Mag. 5, 673–695.CrossRefGoogle Scholar
Di Carlo, D., Irimia, D., Tompkin, R. G. & Toner, M. 2007 Continuous inertial focusing, ordering, and separation of particles in microchannels. Proc. Natl Acad. Sci. USA 104, 18892–18897.CrossRefGoogle ScholarPubMed
Doddi, S. K. & Bagchi, P. 2008 Lateral migration of a capsule in a plane Poiseuille flow in a channel. Intl J. Multiphase Flow 34, 966–986.CrossRefGoogle Scholar
Eckstein, E. C., Bilsker, D. L. & Tilles, A. W. 1987 Transport of platelets in flowing blood. Annu. N.Y. Acad. Sci. 516, 442–452.CrossRefGoogle ScholarPubMed
Farutin, A. & Misbah, C. 2013 Analytical and numerical study of three main migration laws for vesicles under flow. Phys. Rev. Lett. 110, 108104.CrossRefGoogle ScholarPubMed
Fedosov, D. A., Caswell, B., Popel, A. S. & Karniadakis, G. E. 2010 Blood flow and cell-free layer in microvessels. Microcirculation 17, 615–628.CrossRefGoogle ScholarPubMed
Fedosov, D. A., Fornleitner, J. & Gompper, G. 2012 Margination of white blood cells in microcapillary flow. Phys. Rev. Lett. 108, 028104.CrossRefGoogle ScholarPubMed
Geislinger, T. M., Eggart, B., Braunmüller, S., Schmid, L. & Franke, T. 2012 Separation of blood cells using hydrodynamic lift. Appl. Phys. Lett. 100, 183701.CrossRefGoogle Scholar
Ghigliotti, G., Rahimian, A., Biros, G. & Misbah, C. 2011 Vesicle migration and spatial organization driven by flow line curvature. Phys. Rev. Lett. 106, 028101.CrossRefGoogle ScholarPubMed
Goh, C. J., Phan Thien, N. & Atkinson, J. D. 1984 On migration effects in circular Couette flow. J. Chem. Phys. 81, 6259–6265.CrossRefGoogle Scholar
Goldsmith, H. L. 1971 Red cell motions and wall interactions in tube flow. Fed. Proc. 30, 1578–1590.Google ScholarPubMed
Gossett, D. R. & Di Carlo, D. 2019 Particle focusing mechanisms in curving confined flows. Anal. Chem. 81, 8459–8465.CrossRefGoogle Scholar
Grandchamp, X., Coupier, G., Srivastav, A., Minetti, C. & Podgorski, T. 2013 Lift and down-gradient shear-induced diffusion in red blood cell suspensions. Phys. Rev. Lett. 110, 108101.CrossRefGoogle ScholarPubMed
Griggs, A. J., Zinchenko, A. Z. & Davis, R. H. 2007 Low-Reynolds-number motion of a deformable drop between two parallel plane walls. Intl J. Multiphase Flow 33, 182–206.CrossRefGoogle Scholar
Han, H.-C. 2012 Twisted blood vessels: symptoms, etiology and biomechanical mechanisms. J. Vasc. Res. 49, 185–197.CrossRefGoogle ScholarPubMed
Helmy, A. & Barthes-Biesel, D. 1982 Migration of a spherical capsule freely suspended in an unbounded parabolic flow. J. Mec. Theor. Appl. 1, 859–880.Google Scholar
Henríquez Rivera, R. G., Zhang, X. & Graham, M. D. 2016 Mechanistic theory of margination and flow-induced segregation in confined multicomponent suspensions: simple shear and Poiseuille flows. Phys. Rev. Fluids 1, 060501.CrossRefGoogle Scholar
Hur, S. C., Henderson-maclennan, N. K., Mccabe, E. R. B. & Di Carlo, D. 2011 Deformability-based cell classification and enrichment using inertial microfluidics. Lab on a Chip 11, 912–920.CrossRefGoogle ScholarPubMed
Kaoui, B., Ristow, G., Cantat, I., Misbah, C. & Zimmermann, W. 2008 Lateral migration of a two-dimensional vesicle in unbounded Poiseuille flow. Phys. Rev. E 77, 021903.CrossRefGoogle ScholarPubMed
Karimi, A., Yazdi, S. & Ardekani, A. M. 2013 Hydrodynamic mechanisms of cell and particles trapping in microfluidics. Biomicrofluidics 7, 021501.CrossRefGoogle ScholarPubMed
Katanov, D., Gompper, G. & Fedosov, D. A. 2015 Microvascular blood flow resistance: role of red blood cell migration and dispersion. Microvasc. Res. 99, 57–66.CrossRefGoogle ScholarPubMed
Kilimnik, A., Mao, W. & Alexeev, A. 2011 Inertial migration of deformable capsules in channel flow. Phys. Fluids 23, 123302.CrossRefGoogle Scholar
Krüger, T., Kaoui, B. & Harting, J. 2014 Interplay of inertia and deformability on rheological properties of a suspension of capsules. J. Fluid Mech. 751, 725–745.CrossRefGoogle Scholar
Kumar, A. & Graham, M. D. 2012 Mechanism of margination in confined flows of blood and other multicomponent suspensions. Phys. Rev. Lett. 109, 108102.CrossRefGoogle ScholarPubMed
Li, X. & Pozrikidis, C. 2000 Wall-bounded and channel flow of suspensions of liquid drops. Intl J. Multiphase Flow 26, 1247–1279.CrossRefGoogle Scholar
Losserand, S., Coupier, G. & Podgorski, T. 2019 Migration velocity of red blood cells in microchannels. Microvasc. Res. 124, 30–36.CrossRefGoogle ScholarPubMed
Magnaudet, J. 2003 Small inertial effects on a spherical bubble, drop or particle moving near a wall in a time-dependent linear flow. J. Fluid Mech. 485, 115–142.CrossRefGoogle Scholar
Magnaudet, J., Takagi, S. & Legendre, D. 2003 Drag deformation and lateral migration of a buoyant drop moving near a wall. J. Fluid Mech. 476, 115–157.CrossRefGoogle Scholar
Martel, J. M. & Toner, M. 2014 Inertial focusing in microfluidics. Annu. Rev. Biomed. Engng 16, 371–396.CrossRefGoogle ScholarPubMed
Matas, J.-P., Morris, J. F. & Guazzelli, E. 2004 Inertial migration of rigid spherical particles in Poiseuille flow. J. Fluid Mech. 515, 171–195.CrossRefGoogle Scholar
Mehrabadi, M., Ku, D. N. & Aidun, C. K. 2016 Effects of shear rate, confinement, and particle parameters on margination in blood flow. Phys. Rev. E 93, 023109.CrossRefGoogle ScholarPubMed
Misbah, C. 2012 Vesicles, capsules and red blood cells under flow. J. Phys: Conf. Ser. 392, 012005.Google Scholar
Mortazavi, S. & Tryggvason, G. 2000 A numerical study of the motion of drops in Poiseuille flow. Part 1. Lateral migration of one drop. J. Fluid Mech. 411, 325–350.CrossRefGoogle Scholar
Nait-Ouhra, A., Guckenberger, A., Farutin, A., Ez-Zahraouy, H., Benyoussef, A., Gekle, S. & Misbah, C. 2018 Lateral vesicle migration in a bounded shear flow: viscosity contrast leads to off-centered solutions. Phys. Rev. Fluids 3, 123601.CrossRefGoogle Scholar
Nix, S., Imai, Y. & Ishikawa, T. 2016 Lateral migration of a capsule in a parabolic flow. J. Biomech. 49, 2249–2254.CrossRefGoogle Scholar
Nix, S., Imai, Y., Matsunaga, D., Yamaguchi, T. & Ishikawa, T. 2014 Lateral migration of a spherical capsule near a plane wall in stokes flow. Phys. Rev. E 90, 043009.CrossRefGoogle Scholar
Qi, Q. M. & Shaqfeh, E. S. G. 2017 Theory to predict particle migration and margination in the pressure-driven channel flow of blood. Phys. Rev. Fluids 2, 093102.CrossRefGoogle Scholar
Schonberg, J. A. & Hinch, E. J. 1989 Inertial migration of a sphere in Poiseuille flow. J. Fluid Mech. 203, 517–524.CrossRefGoogle Scholar
Segre, G. & Silberberg, A. 1961 Radial particle displacements in Poiseuille flow of suspensions. Nature (London) 189, 209–210.CrossRefGoogle Scholar
Shafer, R. H., Laiken, N. & Zimm, B. H. 1974 Radial migration of DNA molecules in cylindrical flow. Biophys. Chem. 2, 180–184.CrossRefGoogle ScholarPubMed
Shapira, M. & Haber, S. 1990 Low Reynolds number motion of a droplet in shear flow including wall effects. Intl J. Multiphase Flow 16, 305–321.CrossRefGoogle Scholar
Shin, S. J. & Sung, H. J. 2011 Inertial migration of an elastic capsule in a Poiseuille flow. Phys. Rev. E 83, 046321.CrossRefGoogle Scholar
Siggers, J. H. & Waters, S. L. 2005 Steady flows in pipes with finite curvature. Phys. Fluids 17, 077102.CrossRefGoogle Scholar
Sing, R. K., Li, X. & Sarkar, K. 2014 Lateral migration of a capsule in plane shear near a wall. J. Fluid Mech. 739, 421–443.CrossRefGoogle Scholar
Skalak, R., Tozeren, A., Zarda, P. & Chien, S. 1973 Strain energy function of red blood cell membranes. Biophys. J. 13, 245–264.CrossRefGoogle ScholarPubMed
Smart, J. R. & Leighton, D. T. 1991 Measurement of the drift of a droplet due to the presence of a plane. Phys. Fluids 3, 21–31.CrossRefGoogle Scholar
Uijttewaal, W. S. J. & Nijhof, E. J. 1995 The motion of a droplet subjected to linear shear flow including the presence of a wall. J. Fluid Mech. 302, 45–60.CrossRefGoogle Scholar
Vakoc, B. J., Lanning, R. M., Tyrrell, J. A., Padera, T. P., Bartlett, L. A., Stylianopoulos, T., Munn, L. L., Tearney, G. J., Fukumura, D., Jain, R. K., et al. . 2009 Three-dimensional microscopy of the tumor microenvironment in vivo using optical frequency domain imaging. Nat. Med. 15, 1219–1223.CrossRefGoogle ScholarPubMed
Verkaik, A., Beulen, B., Bogaerds, A., Rutten, M. & van de Vosse, F. 2009 Estimation of volume flow in curved tubes based on analytical and computational analysis of axial velocity profiles. Phys. Fluids 21, 023602.CrossRefGoogle Scholar
Wagner, R. C., Czymmek, K. & Hossler, F. E. 2006 Confocal microscopy, computer modeling, and quantification of glomerular vascular corrosion casts. Microsc. Microanal. 12, 262–268.CrossRefGoogle ScholarPubMed
Wang, C. & Bassingthwaighte, J. 2003 Blood flow in small curved tubes. J. Biomech. Engng 125, 910–913.CrossRefGoogle ScholarPubMed
Warkiani, M. E., Khoo, B. L., Wu, L., Tay, A. K. P., Bhagat, A. A. S., Han, J. & Lim, C. T. 2016 Ultra-fast, label-free isolation of circulating tumor cells from blood using spiral microfluidics. Nat. Protoc. 11, 134–148.CrossRefGoogle ScholarPubMed
Yang, B. H., Wang, J., Joseph, D. D., Hu, H. H., Pan, T.-W. & Glowinski, R. 2005 Migration of a sphere in tube flow. J. Fluid Mech. 540, 109–131.CrossRefGoogle Scholar
Yazdani, A. & Bagchi, P. 2013 Influence of membrane viscosity on capsule dynamics in shear flow. J. Fluid Mech. 718, 569–595.CrossRefGoogle Scholar
Ye, T., Phan-Thien, N., Khoo, B. C. & Li, Y. 2018 Flow patterns and red blood cell dynamics in a U-bend. J. Appl. Phys. 124, 124701.CrossRefGoogle Scholar
Ye, T., Phan-Thien, N., Lim, C. T. & Li, Y. 2017 Red blood cell motion and deformation in a curved microvessel. J. Biomech. 65, 12–22.CrossRefGoogle Scholar
Zhao, H., Shaqfeh, E. S. G. & Narsimhan, V. 2012 Shear-induced particle migration and margination in a cellular suspension. Phys. Fluids 24, 011902.CrossRefGoogle Scholar
Zhao, H., Spann, A. P. & Shaqfeh, E. S. G. 2011 The dynamics of a vesicle in a wall-bound shear flow. Phys. Fluids 23, 121901.CrossRefGoogle Scholar
Zong-Can, O. & Helfrich, W. 1989 Bending energy of vesicle membranes: general expressions for the first, second, and third variation of the shape energy and applications to spheres and cylinders. Phys. Rev. A 39, 5280–5288.CrossRefGoogle Scholar
Supplementary material: File

Ebrahimi et al. supplementary material

Ebrahimi et al. supplementary material

Download Ebrahimi et al. supplementary material(File)
File 119.6 KB