Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-12-01T12:37:58.076Z Has data issue: false hasContentIssue false
  • English
  • Français

Linear shape oscillations and polymeric time scales of viscoelastic drops

Published online by Cambridge University Press:  25 September 2013

Günter Brenn  and
Stephan Teichtmeister
Günter Brenn*
Affiliation:
Institute of Fluid Mechanics and Heat Transfer, Graz University of Technology, Inffeldgasse 25/F, 8010 Graz, Austria
Stephan Teichtmeister
Affiliation:
Institute of Fluid Mechanics and Heat Transfer, Graz University of Technology, Inffeldgasse 25/F, 8010 Graz, Austria
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We study small-amplitude axisymmetric shape oscillations of viscoelastic drops in a gas. The Jeffreys model is used as the rheological constitutive equation of the liquid, which represents a liquid with a frequency-dependent dynamic viscosity. 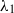The analysis of the time-dependent deformations caused by the oscillations yields the characteristic equation for the complex frequency, which describes the oscillation frequency and damping rate dependence on the viscous liquid behaviour and the stress relaxation and deformation retardation time scales ${\lambda }_{1} $ and ${\lambda }_{2} $ involved in the viscoelastic material law. The aim of this study is to quantify the influences of the two time scales on the oscillation behaviour of the drop and to propose an experimental method to determine one of the time scales by measuring damped oscillations of a drop. A proof-of-concept experiment is presented to show the potential and limitations of the method. Results show that values of 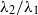${\lambda }_{2} / {\lambda }_{1} $ from these measurements are orders of magnitude smaller than typical values used in simulations of viscoelastic flows.

JFM classification

Waves/Free-surface Flows: Capillary waves Drops and Bubbles: Drops Non-Newtonian Flows: Viscoelasticity
Type
Papers
Information
Journal of Fluid Mechanics , Volume 733 , 25 October 2013 , pp. 504 - 527
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Apfel, R. E., Tian, Y. R., Jankovsky, J., Shi, T., Chen, X., Holt, R. G., Trinh, E., Croonquist, A., Thornton, K. C., Sacco, A. Jr., Coleman, C., Leslie, F. W. & Matthiesen, D. H. 1997 Free oscillations and surfactant studies of superdeformed drops in microgravity. Phys. Rev. Lett. 78, 1912–1915.Google Scholar
Aske, N., Orr, R. & Sjöblom, J. 2002 Dilatational elasticity moduli of water–crude oil interfaces using the oscillating pendant drop. J. Dispersion Sci. Technol. 23, 809–825.Google Scholar
Bauer, H. F. 1985 Surface and interface oscillations in an immiscible spherical visco-elastic system. Acta Mechanica 55, 127–149.CrossRefGoogle Scholar
Bauer, H. F. & Eidel, W. 1987 Vibrations of a visco-elastic spherical immiscible liquid system. Z. Angew. Math. Mech. – J. Appl. Math. Mech. 67, 525–535.Google Scholar
Bird, R. B., Armstrong, R. C. & Hassager, O. 1987 Dynamics of Polymeric Liquids. John Wiley & Sons.Google Scholar
Bird, R. B., Stewart, W. E. & Lightfoot, E. N. 1960 Transport Phenomena. John Wiley & Sons.Google Scholar
Chandrasekhar, S. 1959 The oscillations of a viscous liquid globe. Proc. Lond. Math. Soc. 9, 141–149.Google Scholar
Denn, M. M. 1990 Issues in viscoelastic fluid mechanics. Annu. Rev. Fluid Mech. 22, 13–34.Google Scholar
Egry, I., Lohöfer, G., Seyhan, I., Schneider, S. & Feuerbacher, B. 1998 Viscosity of the eutectic ${\mathrm{Pd} }_{78} {\mathrm{Cu} }_{6} {\mathrm{Si} }_{16} $ measured by the oscillating drop technique in microgravity. Appl. Phys. Lett. 73, 462–463.Google Scholar
Giesekus, H. W. 1994 Phänomenologische Rheologie – Eine Einführung (Phenomenological Rheology – An Introduction). Springer, (in German).Google Scholar
Hiller, W. J. & Kowalewski, T. A. 1989 Surface tension measurements by the oscillating droplet method. Physico-Chem. Hydrodyn. 11, 103–112.Google Scholar
Ho-Minh, D., Mai-Duy, N. & Tran-Cong, T. 2010 A Cartesian-grid integrated-RBF method for viscoelastic flows. IOP Conf. Ser.: Mater. Sci. Engng 10, 012210.CrossRefGoogle Scholar
Hsu, C. J. & Apfel, R. E. 1985 A technique for measuring interfacial tension by quadrupole oscillation of drops. J. Colloid Interface Sci. 107, 467–476.Google Scholar
Huang, P. Y., Hu, H. H. & Joseph, D. D. 1998 Direct simulation of the sedimentation of elliptic particles in Oldroyd-B fluids. J. Fluid Mech. 362, 297–325.CrossRefGoogle Scholar
Joseph, D. D. 1990 Fluid Dynamics of Viscoelastic Liquids. Springer.Google Scholar
Khismatullin, D. B. & Nadim, A. 2001 Shape oscillations of a viscoelastic drop. Phys. Rev. E 63, 061508.Google Scholar
Kovalchuk, V. I., Krägel, J., Aksenenko, E. V., Loglio, G. & Liggieri, L. 2001 Oscillating bubble and drop techniques. In Novel Methods to Study Interfacial Layers, pp. 485–516. Elsevier.CrossRefGoogle Scholar
Lamb, H. 1881 On the oscillations of a viscous spheroid. Proc. Lond. Math. Soc. 13, 51–66.Google Scholar
Lamb, H. 1932 Hydrodynamics, 6th edn. Cambridge University Press.Google Scholar
Larson, R. G. 1988 Constitutive Equations for Polymer Melts and Solutions. Butterworths.Google Scholar
Miller, C. A. & Scriven, L. E. 1968 The oscillations of a fluid droplet immersed in another fluid. J. Fluid Mech. 32, 417–435.Google Scholar
Perez, M., Salvo, L., Suéry, M., Bréchet, Y. & Papoular, M. 2000 Contactless viscosity measurement by oscillations of gas-levitated drops. Phys. Rev. E 61, 2669–2675.Google Scholar
Phillips, T. N. & Williams, A. J. 1999 Viscoelastic flow through a planar contraction using a semi-Lagrangian finite volume method. J. Non-Newtonian Fluid Mech. 87, 215–246.CrossRefGoogle Scholar
Prosperetti, A. 1980 Free oscillations of drops and bubbles: the initial-value problem. J. Fluid Mech. 100, 333–347.Google Scholar
Rayleigh, Lord (J. W. Strutt) 1879 On the capillary phenomena of jets. Proc. R. Soc. Lond. A 29, 71–97.Google Scholar
Stelter, M., Brenn, G., Yarin, A. L., Singh, R. P. & Durst, F. 2000 Validation and application of a novel elongational device for polymer solutions. J. Rheol. 44, 595–616.Google Scholar
Tian, Y. R., Holt, R. G. & Apfel, R. E. 1995 Investigations of liquid surface rheology of surfactant solutions by droplet shape oscillations: theory. Phys. Fluids 7, 2938–2949.CrossRefGoogle Scholar
Trinh, E., Zwern, A. & Wang, T. G. 1982 An experimental study of small-amplitude drop oscillations in immiscible liquid systems. J. Fluid Mech. 115, 453–474.Google Scholar
Tsamopoulos, J. A. & Brown, R. A. 1983 Nonlinear oscillations of inviscid drops and bubbles. J. Fluid Mech. 127, 519–537.CrossRefGoogle Scholar
Yarin, A. L., Brenn, G., Kastner, O., Rensink, D. & Tropea, C. 1999 Evaporation of acoustically levitated droplets. J. Fluid Mech. 399, 151–204.CrossRefGoogle Scholar