Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-26T16:25:44.620Z Has data issue: false hasContentIssue false
  • English
  • Français

Internal shear layers in librating spherical shells: the case of periodic characteristic paths

Published online by Cambridge University Press:  23 March 2022

Jiyang He Open the ORCID record for Jiyang He [Opens in a new window] ,
Benjamin Favier Open the ORCID record for Benjamin Favier [Opens in a new window] ,
Michel Rieutord Open the ORCID record for Michel Rieutord [Opens in a new window]  and
Stéphane Le Dizès Open the ORCID record for Stéphane Le Dizès [Opens in a new window]
Jiyang He*
Affiliation:
Aix Marseille Univ, CNRS, Centrale Marseille, IRPHE, F-13384Marseille, France
Benjamin Favier
Affiliation:
Aix Marseille Univ, CNRS, Centrale Marseille, IRPHE, F-13384Marseille, France
Michel Rieutord
Affiliation:
IRAP, Université de Toulouse, CNRS, UPS, CNES, 14 avenue Édouard Belin, F-31400Toulouse, France
Stéphane Le Dizès
Affiliation:
Aix Marseille Univ, CNRS, Centrale Marseille, IRPHE, F-13384Marseille, France
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

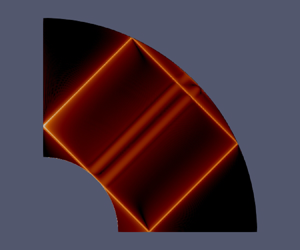
Internal shear layers generated by the longitudinal libration of the inner core in a spherical shell rotating at a rate $\varOmega ^*$ are analysed asymptotically and numerically. The forcing frequency is chosen as $\sqrt {2}\varOmega ^*$ such that the layers issued from the inner core at the critical latitude in the form of concentrated conical beams draw a simple rectangular pattern in meridional cross-sections. The asymptotic structure of the internal shear layers is described by extending the self-similar solution known for open domains to closed domains where reflections on the boundaries occur. The periodic ray path ensures that the beams remain localised around it. Asymptotic solutions for both the main beam along the critical line and the weaker secondary beam perpendicular to it are obtained. The asymptotic predictions are compared with direct numerical results obtained for Ekman numbers as low as $E=10^{-10}$. The agreement between the asymptotic predictions and numerical results improves as the Ekman number decreases. The asymptotic scalings in $E^{1/12}$ and $E^{1/4}$ for the amplitudes of the main and secondary beams, respectively, are recovered numerically. Since the self-similar solution is singular on the axis, a new local asymptotic solution is derived close to the axis and is also validated numerically. This study demonstrates that, in the limit of vanishing Ekman numbers and for particular frequencies, the main features of the flow generated by a librating inner core are obtained by propagating through the spherical shell the self-similar solution generated by the singularity at the critical latitude on the inner core.

JFM classification

Boundary Layers: Boundary layer separation Geophysical and Geological Flows: Waves in rotating fluids
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 939 , 25 May 2022 , A3
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Aldridge, K.D. & Toomre, A. 1969 Axisymmetric inertial oscillations of a fluid in a rotating spherical container. J. Fluid Mech. 37 (2), 307–323.10.1017/S0022112069000565CrossRefGoogle Scholar
Baines, P.G. 1971 a The reflexion of internal/inertial waves from bumpy surfaces. J. Fluid Mech. 46 (2), 273–291.10.1017/S0022112071000533CrossRefGoogle Scholar
Baines, P.G. 1971 b The reflexion of internal/inertial waves from bumpy surfaces. Part 2. Split reflexion and diffraction. J. Fluid Mech. 49 (1), 113–131.10.1017/S0022112071001952CrossRefGoogle Scholar
Brouzet, C., Ermanyuk, E.V., Joubaud, S., Sibgatullin, I. & Dauxois, T. 2016 Energy cascade in internal-wave attractors. Europhys. Lett. 113 (4), 44001.10.1209/0295-5075/113/44001CrossRefGoogle Scholar
Calkins, M.A., Noir, J., Eldredge, J.D. & Aurnou, J.M. 2010 Axisymmetric simulations of libration-driven fluid dynamics in a spherical shell geometry. Phys. Fluids 22 (8), 086602.10.1063/1.3475817CrossRefGoogle Scholar
Cébron, D., Laguerre, R., Noir, J. & Schaeffer, N. 2019 Precessing spherical shells: flows, dissipation, dynamo and the lunar core. Geophys. J. Intl 219 (Supplement_1), S34–S57.10.1093/gji/ggz037CrossRefGoogle Scholar
Cébron, D., Le Bars, M., Noir, J. & Aurnou, J.M. 2012 Libration driven elliptical instability. Phys. Fluids 24 (6), 061703.10.1063/1.4729296CrossRefGoogle Scholar
Dauxois, T., Joubaud, S., Odier, P. & Venaille, A. 2018 Instabilities of internal gravity wave beams. Annu. Rev. Fluid Mech. 50, 131–156.10.1146/annurev-fluid-122316-044539CrossRefGoogle Scholar
Favier, B., Barker, A.J., Baruteau, C. & Ogilvie, G.I. 2014 Non-linear evolution of tidally forced inertial waves in rotating fluid bodies. Mon. Not. R. Astron. Soc. 439 (1), 845–860.10.1093/mnras/stu003CrossRefGoogle Scholar
Favier, B., Grannan, A.M., Le Bars, M. & Aurnou, J.M. 2015 Generation and maintenance of bulk turbulence by libration-driven elliptical instability. Phys. Fluids 27 (6), 066601.10.1063/1.4922085CrossRefGoogle Scholar
Grannan, A.M., Le Bars, M., Cébron, D. & Aurnou, J.M. 2014 Experimental study of global-scale turbulence in a librating ellipsoid. Phys. Fluids 26 (12), 126601.10.1063/1.4903003CrossRefGoogle Scholar
Greenspan, H.P. 1968 The Theory of Rotating Fluids. CUP Archive.Google Scholar
Hollerbach, R. & Kerswell, R.R. 1995 Oscillatory internal shear layers in rotating and precessing flows. J. Fluid Mech. 298, 327–339.10.1017/S0022112095003338CrossRefGoogle Scholar
Kerswell, R.R. 1995 On the internal shear layers spawned by the critical regions in oscillatory Ekman boundary layers. J. Fluid Mech. 298, 311–325.10.1017/S0022112095003326CrossRefGoogle Scholar
Kistovich, Y.V. & Chashechkin, Y.D. 1994 Reflection of packets of internal waves from a rigid plane in a viscous fluid. Izv. Atmos. Ocean. Phys. 30, 752–758.Google Scholar
Koch, S., Harlander, U., Egbers, C. & Hollerbach, R. 2013 Inertial waves in a spherical shell induced by librations of the inner sphere: experimental and numerical results. Fluid Dyn. Res. 45 (3), 035504.10.1088/0169-5983/45/3/035504CrossRefGoogle Scholar
Le Bars, M., Cébron, D. & Le Gal, P. 2015 Flows driven by libration, precession, and tides. Annu. Rev. Fluid Mech. 47 (1), 163–193.10.1146/annurev-fluid-010814-014556CrossRefGoogle Scholar
Le Dizès, S. 2015 Wave field and zonal flow of a librating disk. J. Fluid Mech. 782, 178–208.10.1017/jfm.2015.530CrossRefGoogle Scholar
Le Dizès, S. 2020 Reflection of oscillating internal shear layers: nonlinear corrections. J. Fluid Mech. 899, A21.10.1017/jfm.2020.464CrossRefGoogle Scholar
Le Dizès, S. & Le Bars, M. 2017 Internal shear layers from librating objects. J. Fluid Mech. 826, 653–675.10.1017/jfm.2017.473CrossRefGoogle Scholar
Le Reun, T., Favier, B. & Le Bars, M. 2019 Experimental study of the nonlinear saturation of the elliptical instability: inertial wave turbulence versus geostrophic turbulence. J. Fluid Mech. 879, 296–326.10.1017/jfm.2019.646CrossRefGoogle Scholar
Lemasquerier, D., Grannan, A.M., Vidal, J., Cébron, D., Favier, B., Bars, M.L. & Aurnou, J.M. 2017 Libration-driven flows in ellipsoidal shells. J. Geophys. Res. Planets 122 (9), 1926–1950.10.1002/2017JE005340CrossRefGoogle Scholar
Lin, Y. & Noir, J. 2021 Libration-driven inertial waves and mean zonal flows in spherical shells. Geophys. Astrophys. Fluid Dyn. 115 (3), 258–279.10.1080/03091929.2020.1761350CrossRefGoogle Scholar
Lin, Y. & Ogilvie, G.I. 2018 Tidal dissipation in rotating fluid bodies: the presence of a magnetic field. Mon. Not. R. Astron. Soc. 474 (2), 1644–1656.10.1093/mnras/stx2764CrossRefGoogle Scholar
Lin, Y. & Ogilvie, G.I. 2021 Resonant tidal responses in rotating fluid bodies: global modes hidden beneath localized wave beams. Astrophys. J. Lett. 918 (1), L21.10.3847/2041-8213/ac1f23CrossRefGoogle Scholar
Maas, L.R.M. 2001 Wave focusing and ensuing mean flow due to symmetry breaking in rotating fluids. J. Fluid Mech. 437, 13–28.10.1017/S0022112001004074CrossRefGoogle Scholar
Maas, L., Benielli, D., Sommeria, J. & Lam, F.-P. 1997 Observation of an internal wave attractor in a confined, stably stratified fluid. Nature 388 (6642), 557–561.10.1038/41509CrossRefGoogle Scholar
Manders, A.M.M. & Maas, L.R.M. 2003 Observations of inertial waves in a rectangular basin with one sloping boundary. J. Fluid Mech. 493, 59–88.10.1017/S0022112003005998CrossRefGoogle Scholar
McEwan, A.D. 1970 Inertial oscillations in a rotating fluid cylinder. J. Fluid Mech. 40 (3), 603–640.10.1017/S0022112070000344CrossRefGoogle Scholar
Moore, D.W. & Saffman, P.G. 1969 The structure of free vertical shear layers in a rotating fluid and the motion produced by a slowly rising body. Phil. Trans. R. Soc. Lond. A 264 (1156), 597–634.Google Scholar
Morize, C., Le Bars, M., Le Gal, P. & Tilgner, A. 2010 Experimental determination of zonal winds driven by tides. Phys. Rev. Lett. 104 (21), 214501.10.1103/PhysRevLett.104.214501CrossRefGoogle ScholarPubMed
Mowbray, D.E. & Rarity, B.S.H. 1967 A theoretical and experimental investigation of the phase configuration of internal waves of small amplitude in a density stratified liquid. J. Fluid Mech. 28, 1–16.10.1017/S0022112067001867CrossRefGoogle Scholar
Noir, J., Brito, D., Aldridge, K. & Cardin, P. 2001 Experimental evidence of inertial waves in a precessing spheroidal cavity. Geophys. Res. Lett. 28 (19), 3785–3788.10.1029/2001GL012956CrossRefGoogle Scholar
Noir, J., Cébron, D., Le Bars, M., Sauret, A. & Aurnou, J.M. 2012 Experimental study of libration-driven zonal flows in non-axisymmetric containers. Phys. Earth Planet. Inter. 204, 1–10.10.1016/j.pepi.2012.05.005CrossRefGoogle Scholar
Noir, J., Hemmerlin, F., Wicht, J., Baca, S.M. & Aurnou, J.M. 2009 An experimental and numerical study of librationally driven flow in planetary cores and subsurface oceans. Phys. Earth Planet. Inter. 173 (1-2), 141–152.10.1016/j.pepi.2008.11.012CrossRefGoogle Scholar
Ogilvie, G.I. 2005 Wave attractors and the asymptotic dissipation rate of tidal disturbances. J. Fluid Mech. 543, 19–44.10.1017/S0022112005006580CrossRefGoogle Scholar
Ogilvie, G.I. 2009 Tidal dissipation in rotating fluid bodies: a simplified model. Mon. Not. R. Astron. Soc. 396 (2), 794–806.10.1111/j.1365-2966.2009.14814.xCrossRefGoogle Scholar
Phillips, O.M. 1963 Energy transfer in rotating fluids by reflection of inertial waves. Phys. Fluids 6 (4), 513–520.10.1063/1.1706766CrossRefGoogle Scholar
Phillips, O.M. 1966 The Dynamics of the Upper Ocean. Cambridge University Press.Google Scholar
Rieutord, M. 1987 Linear theory of rotating fluids using spherical harmonics part I: steady flows. Geophys. Astrophys. Fluid Dyn. 39 (3), 163–182.10.1080/03091928708208811CrossRefGoogle Scholar
Rieutord, M., Georgeot, B. & Valdettaro, L. 2001 Inertial waves in a rotating spherical shell: attractors and asymptotic spectrum. J. Fluid Mech. 435, 103–144.10.1017/S0022112001003718CrossRefGoogle Scholar
Rieutord, M. & Valdettaro, L. 1997 Inertial waves in a rotating spherical shell. J. Fluid Mech. 341, 77–99.10.1017/S0022112097005491CrossRefGoogle Scholar
Rieutord, M. & Valdettaro, L. 2010 Viscous dissipation by tidally forced inertial modes in a rotating spherical shell. J. Fluid Mech. 643, 363–394.10.1017/S002211200999214XCrossRefGoogle Scholar
Rieutord, M. & Valdettaro, L. 2018 Axisymmetric inertial modes in a spherical shell at low Ekman numbers. J. Fluid Mech. 844, 597–634.10.1017/jfm.2018.201CrossRefGoogle Scholar
Rieutord, M., Valdettaro, L. & Georgeot, B. 2002 Analysis of singular inertial modes in a spherical shell: the slender toroidal shell model. J. Fluid Mech. 463, 345–360.10.1017/S0022112002008881CrossRefGoogle Scholar
Roberts, P.H. & Stewartson, K. 1963 On the stability of a Maclaurin spheroid of small viscosity. Astrophys. J. 137, 777–790.10.1086/147555CrossRefGoogle Scholar
Stewartson, K. 1966 On almost rigid rotations. Part 2. J. Fluid Mech. 26 (1), 131–144.10.1017/S0022112066001137CrossRefGoogle Scholar
Thomas, N.H. & Stevenson, T.N. 1972 A similarity solution for viscous internal waves. J. Fluid Mech. 54 (3), 495–506.10.1017/S0022112072000837CrossRefGoogle Scholar
Thomas, P.C., Tajeddine, R., Tiscareno, M.S., Burns, J.A., Joseph, J., Loredo, T.J., Helfenstein, P. & Porco, C. 2016 Enceladus's measured physical libration requires a global subsurface ocean. Icarus 264, 37–47.10.1016/j.icarus.2015.08.037CrossRefGoogle Scholar
Walton, I.C. 1975 a On waves in a thin rotating spherical shell of slightly viscous fluid. Mathematika 22 (1), 46–59.10.1112/S0025579300004496CrossRefGoogle Scholar
Walton, I.C. 1975 b Viscous shear layers in an oscillating rotating fluid. Proc. R. Soc. Lond. A 344 (1636), 101–110.Google Scholar