Published online by Cambridge University Press: 19 September 2014
This study considers the interaction of two identical solid axisymmetric bodies (of diameter 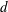 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}d$ and thickness
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}d$ and thickness 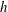 $h$) freely falling in a fluid at rest. We determine the domains of existence of the different interaction behaviour of the two bodies (i.e. attraction, repulsion and indifference) as a function of their initial relative position. We then investigate in detail the case of bodies falling in tandem, for both rectilinear and periodic paths, and the associated attraction behaviour. For all the Reynolds numbers and aspect ratios of the bodies (
$h$) freely falling in a fluid at rest. We determine the domains of existence of the different interaction behaviour of the two bodies (i.e. attraction, repulsion and indifference) as a function of their initial relative position. We then investigate in detail the case of bodies falling in tandem, for both rectilinear and periodic paths, and the associated attraction behaviour. For all the Reynolds numbers and aspect ratios of the bodies (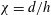 $\chi = d/h$) investigated, the trailing body catches up with the leading body. We provide a quantitative description of the kinematics leading to the regrouping of the bodies and analyse its relationship with the wake of the leading body. In the case of rectilinear paths, a dynamical model that takes into account the axial evolution of the wake of the leading body is proposed to reproduce the acceleration observed for the trailing body until a vertical separation distance between the bodies of 1.5 diameters. In parallel, direct numerical simulations (DNS) of the flow about two fixed bodies in tandem in an oncoming flow are carried out, providing a good estimation of the motion of the bodies for separation distances larger than 5 diameters. For periodic paths, the kinematics leading to the regrouping of the bodies is slower than for rectilinear paths. However, in this case, the interaction also leads to significant changes in the characteristics of the oscillatory motion and is strongly dependent on the aspect ratio of the bodies. To explain the observed differences, we consider the effect of the transverse inhomogeneity of the wake of the leading body on the oscillatory motion of the trailing disk.
$\chi = d/h$) investigated, the trailing body catches up with the leading body. We provide a quantitative description of the kinematics leading to the regrouping of the bodies and analyse its relationship with the wake of the leading body. In the case of rectilinear paths, a dynamical model that takes into account the axial evolution of the wake of the leading body is proposed to reproduce the acceleration observed for the trailing body until a vertical separation distance between the bodies of 1.5 diameters. In parallel, direct numerical simulations (DNS) of the flow about two fixed bodies in tandem in an oncoming flow are carried out, providing a good estimation of the motion of the bodies for separation distances larger than 5 diameters. For periodic paths, the kinematics leading to the regrouping of the bodies is slower than for rectilinear paths. However, in this case, the interaction also leads to significant changes in the characteristics of the oscillatory motion and is strongly dependent on the aspect ratio of the bodies. To explain the observed differences, we consider the effect of the transverse inhomogeneity of the wake of the leading body on the oscillatory motion of the trailing disk.
The movie illustrates the interaction of two thick disks (diameter/thickness = 3) falling in tandem at a Reynolds number close to 100. Two fluorescent dyes were used to visualize the wake of the bodies. The camera is moving vertically at the velocity of the leading body (which is falling at 19 mm/s). We observe that the trailing body accelerates thanks to the entrainment provided by the wake of the leading body and catches up with the leading body. Then, the disks separate laterally and eventually fall side by side.
The movie illustrates the interaction of two thin disks (diameter/thickness = 10) falling in tandem at a Reynolds number close to 80. Two fluorescent dyes were used to visualize the wake of the bodies. The camera is moving vertically at the velocity of the leading body (which is falling at 11 mm/s). We observe that the trailing body accelerates thanks to the entrainment provided by the wake of the leading body and catches up with the leading body. The wakes of the disks merge in a single wake and the bodies continue their fall together adopting a stable Y-configuration.