Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Regan, Marc A.
and
Mahesh, Krishnan
2019.
Adjoint sensitivity and optimal perturbations of the low-speed jet in cross-flow.
Journal of Fluid Mechanics,
Vol. 877,
Issue. ,
p.
330.
Chang, Jianlong
Du, Yang
Shao, Xudong
Zhao, Yongjuan
and
Zheng, Shizhen
2019.
Investigation and analysis of vortex and application of jet in crossflow.
Case Studies in Thermal Engineering,
Vol. 14,
Issue. ,
p.
100459.
Chauvat, Guillaume
Peplinski, Adam
Henningson, Dan S.
and
Hanifi, Ardeshir
2020.
Global linear analysis of a jet in cross-flow at low velocity ratios.
Journal of Fluid Mechanics,
Vol. 889,
Issue. ,
Liu, Enhui
Liu, Xiao
Zhao, Majie
Zheng, Hongtao
Lu, Jinghe
and
Zhang, Zhihao
2020.
Turbulent fuel-air mixing study of jet in crossflow at different velocity ratios using LES.
International Journal of Heat and Fluid Flow,
Vol. 85,
Issue. ,
p.
108633.
Wei, Nathaniel J.
Brownstein, Ian D.
Cardona, Jennifer L.
Howland, Michael F.
and
Dabiri, John O.
2021.
Near-wake structure of full-scale vertical-axis wind turbines.
Journal of Fluid Mechanics,
Vol. 914,
Issue. ,
Chen, Ziyu
Hu, Kexin
Mao, Yinbo
Su, Xinrong
and
Yuan, Xin
2021.
Simple integral model for trajectories of jet deflection in crossflow.
Physics of Fluids,
Vol. 33,
Issue. 11,
Klotz, L.
Pavlenko, A.M.
and
Wesfreid, J.E.
2021.
Experimental measurements in plane Couette–Poiseuille flow: dynamics of the large- and small-scale flow.
Journal of Fluid Mechanics,
Vol. 912,
Issue. ,
Mahjoub, Hammouda
Romdhane, Sahar Ben
Said, Nejla Mahjoub
El-Saeedy, Halemah Ibrahim
Znaidia, Sami
and
El-Rahman, Magda Abd
2022.
Near Source Modeling of Pollutant Emissions From an Elevated Source Over an Urban Area Under Cross High Ventilation.
Journal of Thermal Science and Engineering Applications,
Vol. 14,
Issue. 3,
Morse, Nicholas
and
Mahesh, Krishnan
2023.
Effect of tabs on the shear layer dynamics of a jet in cross-flow.
Journal of Fluid Mechanics,
Vol. 958,
Issue. ,
Gupta, Jyoti
and
Saha, Arun K.
2024.
Characteristics of the jet shear layer of a round elevated jet in crossflow.
Experimental Thermal and Fluid Science,
Vol. 158,
Issue. ,
p.
111269.
Klotz, L.
Bukowski, K.
and
Gumowski, K.
2024.
Influence of porous material on the flow behind a backward-facing step: experimental study.
Journal of Fluid Mechanics,
Vol. 998,
Issue. ,
Lv, Yafei
Huang, Biao
Liu, Taotao
and
Wei, Haipeng
2024.
The flow characteristics for gas jet in liquid crossflow with special emphasis on the vortex-cavity interaction.
European Journal of Mechanics - B/Fluids,
Vol. 104,
Issue. ,
p.
136.
Martins, Francisco C.
and
Pereira, José C. F.
2024.
Numerical Study of Laminar Unsteady Circular and Square Jets in Crossflow in the Low Velocity Ratio Regime.
Fluids,
Vol. 9,
Issue. 12,
p.
292.
Broumand, Mohsen
Yun, Sean
and
Hong, Zekai
2025.
A Large Eddy Simulation Study on Hydrogen Microjets in Hot Vitiated Crossflow.
Journal of Engineering for Gas Turbines and Power,
Vol. 147,
Issue. 3,
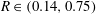 $R\in (0.14,0.75)$ and crossflow Reynolds numbers
$R\in (0.14,0.75)$ and crossflow Reynolds numbers 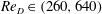 $Re_{D}\in (260,640)$. From spectral analysis we characterize the Strouhal number and amplitude of the hairpin instability as a function of
$Re_{D}\in (260,640)$. From spectral analysis we characterize the Strouhal number and amplitude of the hairpin instability as a function of 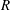 $R$ and
$R$ and 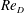 $Re_{D}$. We demonstrate that the dynamics of the hairpins is well described by the Landau model, and, hence, that the instability occurs through Hopf bifurcation, similarly to other hydrodynamical oscillators such as wake behind different bluff bodies. Using the Landau model, we determine the precise threshold values of hairpin shedding. We also study the spatial dependence of this hydrodynamical instability, which shows a global behaviour.
$Re_{D}$. We demonstrate that the dynamics of the hairpins is well described by the Landau model, and, hence, that the instability occurs through Hopf bifurcation, similarly to other hydrodynamical oscillators such as wake behind different bluff bodies. Using the Landau model, we determine the precise threshold values of hairpin shedding. We also study the spatial dependence of this hydrodynamical instability, which shows a global behaviour.