Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-12-02T21:58:51.361Z Has data issue: false hasContentIssue false
  • English
  • Français

Experimental study and modelling of unsteady aerodynamic forces and moment on flat plate in high amplitude pitch ramp motion

Published online by Cambridge University Press:  03 May 2018

Yuelong Yu ,
Xavier Amandolese Open the ORCID record for Xavier Amandolese [Opens in a new window] ,
Chengwei Fan  and
Yingzheng Liu
Yuelong Yu
Affiliation:
Gas Turbine Research Institute/School of Mechanical Engineering, Shanghai Jiao Tong University, 800 Dongchuan Road, Shanghai 200240, PR China
Xavier Amandolese*
Affiliation:
LadHyX, CNRS-Ecole Polytechnique, F-91128 Palaiseau, France Conservatoire National des Arts et Métiers, F-75141 Paris, France
Chengwei Fan
Affiliation:
LadHyX, CNRS-Ecole Polytechnique, F-91128 Palaiseau, France Institute of Engineering Thermophysics, Shanghai Jiao Tong University, 800 Dongchuan Road, Shanghai 200240, PR China
Yingzheng Liu
Affiliation:
Gas Turbine Research Institute/School of Mechanical Engineering, Shanghai Jiao Tong University, 800 Dongchuan Road, Shanghai 200240, PR China
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

This paper examines the unsteady lift, drag and moment coefficients experienced by a thin airfoil in high-amplitude pitch ramp motion. Experiments have been carried out in a wind tunnel at moderate Reynolds number ( 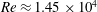$Re\approx 1.45\times 10^{4}$ ), using a rigid flat-plate model. Forces and moments have been measured for reduced pitch rates ranging from 0.01 to 0.18, four maximum pitch angles ( 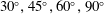$30^{\circ },45^{\circ },60^{\circ },90^{\circ }$ ) and different pivot axis locations between the leading and the trailing edge. Results confirm that for reduced pitch rates lower than 0.03, the unsteady aerodynamics is limited to a stall delay effect. For higher pitch rates, the unsteady response is dominated by a buildup of the circulation, which increases with the pitch rate and the absolute distance between the pivot axis and the 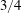$3/4$ -chord location. This circulatory effect induces an overshoot in the normal force and moment coefficients, which is slightly reduced for a flat plate with a finite aspect ratio close to 8 in comparison with the two-dimensional configuration. A new time-dependent model has been tested for both the normal force and moment coefficients. It is mainly based on the superposition of step responses, using the Wagner function and a time-varying input that accounts for the nonlinear variation of the steady aerodynamics, the pivot point location and an additional circulation which depends on the pitch rate. When compared with experiments, it gives satisfactory results for 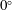$0^{\circ }$ to 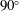$90^{\circ }$ pitch ramp motion and captures the main effect of reduced pitch rate and pivot point location.

JFM classification

Aerodynamics: Aerodynamics Biological Fluid Dynamics: Swimming/flying
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 846 , 10 July 2018 , pp. 82 - 120
Check for updates
Copyright
© 2018 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Anderson, J. D. Jr 2010 Fundamentals of Aerodynamics. Tata McGraw-Hill Education.Google Scholar
Ansari, S., Żbikowski, R. & Knowles, K. 2006 Aerodynamic modelling of insect-like flapping flight for micro air vehicles. Prog. Aerosp. Sci. 42, 129–172.CrossRefGoogle Scholar
Babinsky, H., Stevens, P., Jones, A. R., Bernal, L. P. & Ol, M. V.2016 Low order modelling of lift forces for unsteady pitching and surging wings. In 54th AIAA Aerospace Sciences Meeting, AIAA SciTech Forum, AIAA 2016-0290.Google Scholar
Beddoes, T. 1982 Practical computation of unsteady lift. Vertica 8 (1), 55–71.Google Scholar
Cory, R. & Tedrake, R.2008 Experiments in fixed-wing UAV perching. In AIAA Guidance, Navigation and Control Conference and Exhibit, Guidance, Navigation, and Control and Co-located Conferences, AIAA 2008-7256.Google Scholar
Desbiens, A. L., Asbeck, A. & Cutkosky, M. 2010 Hybrid aerial and scansorial robotics. In Robotics and Automation (ICRA), 2010 IEEE International Conference on Robotics and Automation, pp. 1114–1115. IEEE.Google Scholar
Dickinson, M. H. 1999 Wing rotation and the aerodynamic basis of insect flight. Science 284, 1954–1960.Google Scholar
Donely, P.1950 Summary of information relating to gust loads on airplanes. NACA Technical Note 1976.Google Scholar
Farren, W.1935 The reaction on a wing whose angle of incidence is changing rapidly. Aeronautical Research Committee, Reports and Memoranda 1648.Google Scholar
Ford, C. P. & Babinsky, H. 2013 Lift and the leading-edge vortex. J. Fluid Mech. 720, 280–313.CrossRefGoogle Scholar
Forsythe, G. E., Moler, C. B. & Malcolm, M. A. 1977 Computer Methods for Mathematical Computations. Prentice-Hall.Google Scholar
Fung, Y. C. 2002 An Introduction to the Theory of Aeroelasticity. Dover Publications.Google Scholar
Gault, D. E.1957 An investigation at low speed of the flow over a simulated flat plate at small angles of attack using pitot-static and hot-wire probes. NACA Tech. Note 3876.Google Scholar
Granlund, K., Ol, M. & Bernal, L.2011a Experiments on pitching plates: force and flowfield measurements at low Reynolds numbers. In 49th AIAA Aerospace Sciences Meeting including the New Horizons Forum and Aerospace Exposition, Aerospace Sciences Meetings, AIAA 2011-872.Google Scholar
Granlund, K., Ol, M. & Bernal, L.2011b Flowfield evolution vs. lift coefficient history for rapidly-pitching low aspect ratio plates. In 6th AIAA Theoretical Fluid Mechanics Conference, Fluid Dynamics and Co-located Conferences, AIAA 2011-3118.Google Scholar
Granlund, K. O., Ol, M. V. & Bernal, L. P. 2013 Unsteady pitching flat plates. J. Fluid Mech. 733 (6), 429–434.Google Scholar
Granlund, K., Ol, M., Garmann, D., Visbal, M. & Bernal, L.2010 Experiments and computations on abstractions of perching. In 28th AIAA Applied Aerodynamics Conference, Fluid Dynamics and Co-located Conferences, AIAA 2010-4943.Google Scholar
Green, S. I. 1995 Wing Tip Vortices. Springer.Google Scholar
Guerrero, J., Pacioselli, C., Pralits, J., Negrello, F., Silvestri, P., Lucifredi, A. & Bottaro, A. 2016 Preliminary design of a small-sized flapping UAV: I. Aerodynamic performance and static longitudinal stability. Meccanica 51, 1343–1367.CrossRefGoogle Scholar
Harper, P. W. & Flanigan, R. E.1950 The effect of rate of change of angle of attack on the maximum lift of a small model. NACA Tech. Note 2061.Google Scholar
Helin, H. E. & Walker, J. M.1985 Interrelated effects of pitch rate and pivot point on airfoil dynamic stall. In 23rd Aerospace Sciences Meeting, AIAA 85-0130.Google Scholar
Herbst, W.1983 Supermaneuverability (for fighter aircraft tactical capabilities). Jahrestagung der Deutsche Gesellschaft für Luft- und Raumfahrt, Munich, West Germany, p. 17.Google Scholar
Jones, R. T.1940 The unsteady lift of a wing of finite aspect ratio. NACA Tech. Rep. 681.Google Scholar
Koochesfahani, M. M. & Smiljanovski, V. 1993 Initial acceleration effects on flow evolution around airfoils pitching to high angles of attack. AIAA J. 31, 1529–1531.Google Scholar
Kramer, V. M. 1932 Die Zunahme des Maximalauftriebes von Tragflugeln bei plotzlicher Anstellwinkelvergrosserung (Boeneffekt). Z. Flugtech. Motorluftschiff 23, 185–189.Google Scholar
Leishman, J. G. 2006 Principles of Helicopter Aerodynamics with CD Extra. Cambridge University Press.Google Scholar
Leishman, J. G. & Beddoes, T. 1989 A semi-empirical model for dynamic stall. J. Am. Helicopter Soc. 34, 3–17.Google Scholar
Lorber, P. F. & Carta, F. 1988 Airfoil dynamic stall at constant pitch rate and high Reynolds number. J. Aircraft 25, 548–556.Google Scholar
Mancini, P., Manar, F., Granlund, K., Ol, M. V. & Jones, A. R. 2015 Unsteady aerodynamic characteristics of a translating rigid wing at low Reynolds number. Phys. Fluids 27 (12), 2691–2704.Google Scholar
McCroskey, W.1981 The phenomenon of dynamic stall. NASA Tech. Mem. 81264.Google Scholar
Mueller, T. J. 2001 Fixed and Flapping Wing Aerodynamics for Micro Air Vehicle Applications. American Institute of Aeronautics and Astronautics.Google Scholar
Ol, M. V., Altman, A., Eldredge, J. D., Garmann, D. J. & Lian, Y.2010 Resume of the AIAA FDTC low Reynolds number discussion group’s canonical cases. In 48th AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace Exposition, AIAA 2010-1085.Google Scholar
Ol, M. V., Eldredge, J. D. & Wang, C. 2009 High-amplitude pitch of a flat plate: an abstraction of perching and flapping. Intl J. Micro Air Vehicles 1, 203–216.Google Scholar
Ramezani, A., Chung, S.-J. & Hutchinson, S. 2017 A biomimetic robotic platform to study flight specializations of bats. Sci. Robot. 2, eaal2505.CrossRefGoogle ScholarPubMed
Reich, G., Wojnar, O. & Albertani, R.2009 Aerodynamic performance of a notional perching MAV design. In 47th AIAA Aerospace Sciences Meeting, AIAA 2009-63.Google Scholar
Sane, S. P. & Dickinson, M. H. 2002 The aerodynamic effects of wing rotation and a revised quasi-steady model of flapping flight. J. Expl Biol. 205, 1087–1096.Google Scholar
Sheng, W., Galbraith, R. M. & Coton, F. 2006 A new stall-onset criterion for low speed dynamic-stall. J. Solar Energy Engng 128, 461–471.Google Scholar
Sheng, W., Galbraith, R. A. M. & Coton, F. N. 2008 Prediction of dynamic stall onset for oscillatory low-speed airfoils. J. Fluids Engng 130 (10), 1135–1150.Google Scholar
Shyy, W., Aono, H., Chimakurthi, S. K., Trizila, P., Kang, C.-K., Cesnik, C. E. & Liu, H. 2010 Recent progress in flapping wing aerodynamics and aeroelasticity. Prog. Aerosp. Sci. 46, 284–327.Google Scholar
Shyy, W., Lian, Y., Tang, J., Viieru, D. & Liu, H. 2007 Aerodynamics of Low Reynolds Number Flyers. Cambridge University Press.Google Scholar
Son, O., Cetiner, O., Stevens, P., Babinsky, H., Manar, F., Mancini, P., Jones, A. R., Ol, M. V. & Gozukara, A. C.2016 Parametric variations in aspect ratio, leading edge and planform shapes for the rectilinear pitch cases of AVT-202. In 54th AIAA Aerospace Sciences Meeting, AIAA 2016-0289.Google Scholar
Stevens, P. & Babinsky, H. 2017 Experiments to investigate lift production mechanisms on pitching flat plates. Exp. Fluids 58, 7.Google Scholar
Strickland, J. & Graham, G. 1987 Force coefficients for a NACA-0015 airfoil undergoing constant pitchrate motions. AIAA J. 25, 622–624.Google Scholar
Taha, H. E., Hajj, M. R. & Beran, P. S. 2014 State-space representation of the unsteady aerodynamics of flapping flight. Aerosp. Sci. Technol. 34, 1–11.Google Scholar
Taha, H. E., Hajj, M. R. & Nayfeh, A. H. 2012 Flight dynamics and control of flapping-wing MAVs: a review. Nonlinear Dyn. 70, 907–939.Google Scholar
Terutsuki, D., Deng, X., Crossley, W. A. & Kohtake, N. 2015 Concept selection method in reverse to describe mission that best use a new technology: Ornithopter. In 2015 IEEE Aerospace Conference, pp. 1–8.Google Scholar
Theodorsen, T.1935 General theory of aerodynamic instability and the mechanism of flutter. NACA Technical Report 496.Google Scholar
Tran, C. & Petot, D. 1980 Semi-empirical model for the dynamic stall of airfoils in view of the application to the calculation of responses of a helicopter blade in forward flight. Vertica 5 (1), 35–53.Google Scholar
Videler, J., Stamhuis, E. & Povel, G. 2004 Leading-edge vortex lifts swifts. Science 306, 1960–1962.Google Scholar
Wagner, H. 1925 Über die Entstehung des dynamischen Auftriebes von Tragflügeln. Z. Angew. Math. Mech. 5, 17–35.Google Scholar
Walker, J., Helin, H. & Strickland, J. 1985 An experimental investigation of an airfoil undergoing large-amplitude pitching motions. AIAA J. 23, 1141–1142.Google Scholar
Wick, B. H.1954 Study of the subsonic forces and moments on an inclined plate of infinite span. NACA Tech. Note 3221.Google Scholar
Yu, H.-T. & Bernal, L.2013 Effect of pivot point on aerodynamic force and vortical structure of pitching flat plate wings. In 51st AIAA Aerospace Sciences Meeting including the New Horizons Forum and Aerospace Exposition, Aerospace Sciences Meetings, AIAA 2013-0792.Google Scholar