Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-21T07:50:13.089Z Has data issue: false hasContentIssue false
  • English
  • Français

Unstable Richtmyer–Meshkov growth of solid and liquid metals in vacuum

Published online by Cambridge University Press:  13 June 2012

W. T. Buttler ,
D. M. Oró ,
D. L. Preston ,
K. O. Mikaelian ,
F. J. Cherne ,
R. S. Hixson ,
F. G. Mariam ,
C. Morris ,
J. B. Stone  and
G. Terrones
...Show all authors
W. T. Buttler*
Affiliation:
Los Alamos National Laboratory, Physics, Los Alamos, NM 87544, USA
D. M. Oró
Affiliation:
Los Alamos National Laboratory, Physics, Los Alamos, NM 87544, USA
D. L. Preston
Affiliation:
Los Alamos National Laboratory, X-Computational Physics, Los Alamos, NM 87544, USA
K. O. Mikaelian
Affiliation:
Lawrence Livermore National Laboratory, B-Division, Livermore, CA 94550, USA
F. J. Cherne
Affiliation:
Los Alamos National Laboratory, Shock & Detonation Physics, Los Alamos, NM 87544, USA
R. S. Hixson
Affiliation:
Los Alamos National Laboratory, Physics, Los Alamos, NM 87544, USA
F. G. Mariam
Affiliation:
Los Alamos National Laboratory, Physics, Los Alamos, NM 87544, USA
C. Morris
Affiliation:
Los Alamos National Laboratory, Physics, Los Alamos, NM 87544, USA
J. B. Stone
Affiliation:
Los Alamos National Laboratory, Physics, Los Alamos, NM 87544, USA
G. Terrones
Affiliation:
Los Alamos National Laboratory, X-Theoretical Design, Los Alamos, NM 87544, USA
D. Tupa
Affiliation:
Los Alamos National Laboratory, Physics, Los Alamos, NM 87544, USA
*
†Email address for correspondence: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We present experimental results supporting physics-based ejecta model development, where our main assumption is that ejecta form as a special limiting case of a Richtmyer–Meshkov (RM) instability at a metal–vacuum interface. From this assumption, we test established theory of unstable spike and bubble growth rates, rates that link to the wavelength and amplitudes of surface perturbations. We evaluate the rate theory through novel application of modern laser Doppler velocimetry (LDV) techniques, where we coincidentally measure bubble and spike velocities from explosively shocked solid and liquid metals with a single LDV probe. 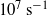We also explore the relationship of ejecta formation from a solid material to the plastic flow stress it experiences at high-strain rates () and high strains (700 %) as the fundamental link to the onset of ejecta formation. Our experimental observations allow us to approximate the strength of Cu at high strains and strain rates, revealing a unique diagnostic method for use at these extreme conditions.

JFM classification

Drops and Bubbles: Breakup/coalescence Instability: Nonlinear instability
Type
Papers
Information
Journal of Fluid Mechanics , Volume 703 , 25 July 2012 , pp. 60 - 84
Copyright
Copyright © Cambridge University Press 2012

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1. Bourne, N. K. & Gray, G. T. III 2005a Computational design of recovery experiments for ductile materials. Proc. R. Soc. A 461, 3297–3313.CrossRefGoogle Scholar
2. Bourne, N. K. & Gray, G. T. III. 2005b Soft-recovery of shocked polymers and composites. J. Phys. D 38, 3690–3694.CrossRefGoogle Scholar
3. Buttler, W. T. 2008 Comment on ‘Accuracy limits and window corrections for photon Doppler velocimetry’ (J. Appl. Phys. 101, 013523 (2007)). J. Appl. Phys. 103, 046102.Google Scholar
4. Buttler, W. T., Lamoreaux, S. K., Omenetto, F. G. & Torgerson, J. R. 2004 Optical velocimetry. arXiv:physics/0409073v1.Google Scholar
5. Buttler, W. T., Routley, N., Hixson, R. S., King, N. S. P., Olson, R. T., Rigg, P. A., Rimmer, A. & Zellner, M. B. 2007a Method to separate and determine the amount of ejecta produced in a second material-fragmentation event. Appl. Phys. Lett. 90, 151921.CrossRefGoogle Scholar
6. Buttler, W. T. & Zellner, M. B. 2007 Tin ejecta data review: toward a statistical material fragmentation model, Milestone 2478. Tech. Rep. LA-UR-07-6522, Los Alamos National Laboratory.Google Scholar
7. Buttler, W. T., Zellner, M. B., Olson, R. T., Rigg, P. A., Hixson, R. S., Hammerberg, J. E., Obst, A. W., Payton, J. R., Iverson, A. & Young, J. 2007b Dynamic comparisons of piezoelectric ejecta diagnostics. J. Appl. Phys. 101, 063547.Google Scholar
8. Buttler, W. T. & Zellner, M. B. Unpublished results gathered between 2005 and 2011.Google Scholar
9. Dimonte, G. & Ramaprabhu, R. 2010 Simulations and model of the nonlinear Richtmyer–Meshkov instability. Phys. Fluids 22, 014104.CrossRefGoogle Scholar
10. Douence, V. M., Bai, Y., Durmus, H., Joshi, A. B., Pettersson, P.-O., Sahoo, D., Kwiatkowski, K., King, N. S., Morris, C. & Wilke, M. D. 2005 Hybrid image sensor with multiple on-chip frame storage for ultrahigh-speed imaging. Proc. SPIE 5580, 226–234.Google Scholar
11. Forman, J. W. Jr., George, E. W. & Lewis, R. D. 1965a Feasibility study of a laser flowmeter for local velocity measurements in gas flow fields. Tech. Note #149, Teledyne Brown Engineering Co. Inc., Huntsville, AL.Google Scholar
12. Forman, J. W. Jr., George, E. W. & Lewis, R. D. 1965b Measurement of localized fluid flow velocities in gasses with a laser Doppler flowmeter. Appl. Phys. Lett. 7, 77–78.Google Scholar
13. Greeff, C., Chisolm, E. & George, D. 2005 SESAME 2161: An explicit multiphase equation of state for tin. Tech. Rep. LA-UR-05-9414, Los Alamos National Laboratory.Google Scholar
14. King, N. S. P., Ables, E., Adams, K., Alrick, K. R., Amann, J. F., Balzar, S., Barnes, P. D. Jr., Crow, M. L., Cushing, S. B., Eddleman, J. C., Fife, T. T., Flores, P., Fujino, D., Gallegos, R. A., Gray, N. T., Hartouni, E. P., Hogan, G. E., Holmes, V. H., Jaramillo, S. A., Knudsson, J. N., London, R. K., Lopez, R. R., McDonald, T. E., McClelland, J. B., Merrill, F. E., Morley, K. B., Morris, C. L., Naivar, F. J., Parker, E. L., Park, H. S., Pazuchanics, P. D., Pillai, C., Riedel, C. M., Sarracino, J. S., Shelley, F. E. Jr., Stacy, H. L., Takala, B. E., Thompson, R., Tucker, H. E., Yates, G. J., Ziock, H.-J. & Zumbro, J. D. 1999 An 800-MeV proton radiography facility for dynamic experiments. Nucl. Instrum. Meth. A 424, 84–91.CrossRefGoogle Scholar
15. Mabire, C. & Hereil, P. L. 2000a Shock induced polymorphic transition and melting of tin. In Proceedings of the American Physical Society Topical Group on Shock Compression of Condensed Matter, Snowbird, Ut, 27 June–2 July 1999, vol. 505, pp. 93-96.Google Scholar
16. Mabire, C. & Hereil, P. L. 2000b Shock induced polymorphic transition and melting of tin up to 53 GPa (experimental study and modelling). J. Physique IV 10, 749–754.Google Scholar
17. Merrill, F. E., Campos, E., Espinoza, C., Hogan, G., Hollander, B., Lopez, J., Mariam, F. G., Morley, D., Morris, C. L., Murray, M., Saunders, A., Schwartz, C. & Thompson, T. N. 2011 Magnifying lens for 800 MeV proton radiography. Rev. Sci. Instrum. 82, 103709.CrossRefGoogle ScholarPubMed
18. Meshkov, E. E. 1969 Instability in shock-accelerated boundary separating two gasses. Izv. Akad. Nauk SSSR Mekh. Zhidk. Gaza 5, 151–158.Google Scholar
19. Meyer, K. A. & Blewett, P. J. 1972 Numerical investigation of the stability of a shock-accelerated interface between two fluids. Phys. Fluids 15, 753–759.CrossRefGoogle Scholar
20. Mikaelian, K. O. 1998 Analytical approach to nonlinear Rayleigh–Taylor and Richtmyer–Meshkov instabilities. Phys. Rev. Lett. 80, 508–511.Google Scholar
21. Mikaelian, K. O. 2010 Analytical approach to nonlinear hydrodynamic instabilities driven by time-dependent accelerations. Phys. Rev. E 81, 016325.Google Scholar
22. Morris, C., Hopson, J. W. & Goldstone, P. 2006 Proton radiography. Los Alamos Science 30, 32–45. http://la-science.lanl.gov/lascience30.shtml.Google Scholar
23. Peterson, J. H., Honnell, K. G., Greeff, C., Johnson, J. D., Boettger, J. C. & Crockett, S. D. 2012 Global equation of state for copper. In Proceedings of the Conference of the American Physical Society Topical Group on Shock Compression of Condensed Matter, Chigago, IL, USA, 26 June–1 July 2011, vol. 1426, pp. 763–766.Google Scholar
24. Piriz, A. R., Lopez-Cela, J. J., Tahir, N. A. & Hoffmann, D. H. H. 2008 Richtmyer–Meshkov instability in elastic–plastic media. Phys. Rev. E 78, 056401.Google Scholar
25. Piriz, A. R., Lopez-Cela, J. J. & Tahir, N. A. 2009 Richtmyer–Meshkov instability as a tool for evaluating material strength under extreme conditions. Nucl. Instrum. Meth. Phys. Res. A 606, 139–141.CrossRefGoogle Scholar
26. Preston, D. L., Tonks, D. L. & Wallace, D. C. 2003 Model of plastic deformations for extreme loading conditions. J. Appl. Phys. 93, 211–220.CrossRefGoogle Scholar
27. Richtmyer, R. D. 1960 Taylor instability in shock acceleration of compressible fluids. Commun. Pure Appl. Math. 13, 297–319.CrossRefGoogle Scholar
28. Strand, O. T., Goosman, D. R., Martinez, C. & Whitworth, T. L. 2006 Compact system for high-speed velocimetry using heterodyne techniques. Rev. Sci. Instrum. 77, 083108.CrossRefGoogle Scholar
29. Velikovich, A. L. & Dimonte, G. 1996 Nonlinear perturbation theory of the incompressible Richtmyer–Meshkov instability. Phys. Rev. Lett. 76, 3112–3115.CrossRefGoogle ScholarPubMed
30. Vogan, W. S., Anderson, W. W., Grover, M., Hammerberg, J. E., King, N. S. P., Lamoreaux, S. K., Macrum, G., Morley, K. B., Rigg, P. A., Stevens, G. D., Turley, W. D., Veeser, L. R. & Buttler, W. T. 2005 Piezoelectric characterization of ejecta from shocked tin surfaces. J. Appl. Phys. 98, 113508.Google Scholar
31. Yeh, Y. & Cummins, H. Z. 1964 Localized fluid flow measurements with an He–Ne laser. App. Phys. Lett. 4, 176–178.Google Scholar
32. Zellner, M. B. & Buttler, W. T. 2008 Exploring Richtmyer–Meshkov instability phenomena and ejecta cloud physics. Appl. Phys. Lett. 93, 114102.Google Scholar
33. Zellner, M. B., Grover, M., Hammerberg, J. E., Hixson, R. S., Iverson, A. J., Macrum, G. S., Morley, K. B., Obst, A. W., Olson, R. T., Payton, J. R., Rigg, P. A., Routley, N., Stevens, G. D., Turley, W. D., Veeser, L. & Buttler, W. T. 2007 Effects of shock breakout pressure on ejection of material from shocked tin surfaces. J. Appl. Phys. 102, 013522. Erratum: Effects of shock-breakout pressure on ejection of material from shocked tin surfaces. J. Appl. Phys. (2008) 103, 109901.CrossRefGoogle Scholar
34. Zellner, M. B., Vogan-McNeil, W., Gray, G. T. III, Huerta, D. C., King, N. S. P., Neal, G. E., Valentine, S. J., Payton, J. R., Rubin, J., Stevens, G. D., Turley, W. D. & Buttler, W. T. 2008a Surface preparation methods to enhance dynamic surface property measurements of shocked metal surfaces. J. Appl. Phys. 103, 083521.Google Scholar
35. Zellner, M. B., Vogan-McNeil, W., Hammerberg, J. E., Hixson, R. S., Obst, A. W., Olson, R. T., Payton, J. R., Rigg, P. A., Routley, N., Stevens, G. D., Turley, W. D., Veeser, L. & Buttler, W. T. 2008b Probing the underlying physics of ejecta production from shocked Sn samples. J. Appl. Phys. 103, 123502.CrossRefGoogle Scholar
36. Zhang, Q. 1998 Analytical solution of Layzer-type approach to unstable interfacial fluid mixing. Phys. Rev. Lett. 81, 3391–3394.CrossRefGoogle Scholar