Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Salesky, Scott T.
and
Anderson, W.
2020.
Revisiting inclination of large-scale motions in unstably stratified channel flow.
Journal of Fluid Mechanics,
Vol. 884,
Issue. ,
Cheng, Cheng
Li, Weipeng
Lozano-Durán, Adrián
and
Liu, Hong
2020.
Uncovering Townsend’s wall-attached eddies in low-Reynolds-number wall turbulence.
Journal of Fluid Mechanics,
Vol. 889,
Issue. ,
2020.
Characteristics of wall-attached motions in open channel flows.
Physics of Fluids,
Vol. 32,
Issue. 5,
p.
055110.
Motoori, Yutaro
and
Goto, Susumu
2020.
Hairpin vortices in the largest scale of turbulent boundary layers.
International Journal of Heat and Fluid Flow,
Vol. 86,
Issue. ,
p.
108658.
Farsiani, Yasaman
Saeed, Zeeshan
Jayaraman, Balaji
and
Elbing, Brian R.
2020.
Modification of turbulent boundary layer coherent structures with drag reducing polymer solution.
Physics of Fluids,
Vol. 32,
Issue. 1,
Deshpande, Rahul
Chandran, Dileep
Monty, Jason P.
and
Marusic, Ivan
2020.
Two-dimensional cross-spectrum of the streamwise velocity in turbulent boundary layers.
Journal of Fluid Mechanics,
Vol. 890,
Issue. ,
Li, Xuebo
Wang, Guohua
and
Zheng, Xiaojing
2021.
Logarithmic energy profile of the streamwise velocity for wall-attached eddies along the spanwise direction in turbulent boundary layer.
Physics of Fluids,
Vol. 33,
Issue. 10,
Deshpande, Rahul
de Silva, Charitha M.
Lee, Myoungkyu
Monty, Jason P.
and
Marusic, Ivan
2021.
Data-driven enhancement of coherent structure-based models for predicting instantaneous wall turbulence.
International Journal of Heat and Fluid Flow,
Vol. 92,
Issue. ,
p.
108879.
Deshpande, Rahul
Monty, Jason P.
and
Marusic, Ivan
2021.
Active and inactive components of the streamwise velocity in wall-bounded turbulence.
Journal of Fluid Mechanics,
Vol. 914,
Issue. ,
Kumar, S. Santosh
Huang, Xinyi
Yang, Xiang
and
Hong, Jiarong
2021.
Three dimensional flow motions in the viscous sublayer.
Theoretical and Applied Mechanics Letters,
Vol. 11,
Issue. 2,
p.
100239.
Cheng, Cheng
Shyy, Wei
and
Fu, Lin
2022.
Streamwise inclination angle of wall-attached eddies in turbulent channel flows.
Journal of Fluid Mechanics,
Vol. 946,
Issue. ,
Wang, Longwei
Pan, Chong
Wang, Jinjun
and
Gao, Qi
2022.
Statistical signatures of component wall-attached eddies in proper orthogonal decomposition modes of a turbulent boundary layer.
Journal of Fluid Mechanics,
Vol. 944,
Issue. ,
Li, Xuebo
Hutchins, Nicholas
Zheng, Xiaojing
Marusic, Ivan
and
Baars, Woutijn J.
2022.
Scale-dependent inclination angle of turbulent structures in stratified atmospheric surface layers.
Journal of Fluid Mechanics,
Vol. 942,
Issue. ,
2023.
Statistical behavior of wall-attached motions in open- and closed-channel flows via direct numerical simulation.
Physics of Fluids,
Vol. 35,
Issue. 4,
Chan, C.I.
and
Chin, R.C.
2023.
Turbulent boundary layer flow over a three-dimensional sinusoidal surface.
Journal of Fluid Mechanics,
Vol. 975,
Issue. ,
2023.
Reduced-order representation of superstructures in a turbulent boundary layer.
Physics of Fluids,
Vol. 35,
Issue. 5,
Chan, C.I.
and
Chin, R.C.
2023.
POD analysis of the turbulent boundary layer flow downstream of miniature vortex generators.
International Journal of Heat and Fluid Flow,
Vol. 103,
Issue. ,
p.
109175.
Maryada, K.R.
Armfield, S.W.
Dhopade, P.
and
Norris, S.E.
2023.
Large-scale motions in a turbulent natural convection boundary layer immersed in a stably stratified environment.
Journal of Fluid Mechanics,
Vol. 967,
Issue. ,
Liu, Yixun
Liu, Chun-Ho
Brasseur, Guy P.
and
Chao, Christopher Y.H.
2023.
Empirical mode decomposition of the atmospheric flows and pollutant transport over real urban morphology.
Environmental Pollution,
Vol. 331,
Issue. ,
p.
121858.
Cheng, Cheng
and
Fu, Lin
2023.
Linear-model-based study of the coupling between velocity and temperature fields in compressible turbulent channel flows.
Journal of Fluid Mechanics,
Vol. 964,
Issue. ,
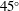 $45^{\circ }$. This is consistent with assumptions used in prior attached eddy model-based simulations. Given that the inclination angle obtained via standard two-point correlations is influenced by the range of scales in the turbulent flow (Marusic, Phys. Fluids, vol. 13 (3), 2001, pp. 735–743), the present result is obtained by isolating the large wall-attached structures from the rest of the turbulence. This is achieved by introducing a spanwise offset between two hot-wire probes, synchronously measuring the streamwise velocity at a near-wall and log-region reference location, to assess the wall coherence. The methodology is shown to be effective by applying it to data sets across Reynolds numbers,
$45^{\circ }$. This is consistent with assumptions used in prior attached eddy model-based simulations. Given that the inclination angle obtained via standard two-point correlations is influenced by the range of scales in the turbulent flow (Marusic, Phys. Fluids, vol. 13 (3), 2001, pp. 735–743), the present result is obtained by isolating the large wall-attached structures from the rest of the turbulence. This is achieved by introducing a spanwise offset between two hot-wire probes, synchronously measuring the streamwise velocity at a near-wall and log-region reference location, to assess the wall coherence. The methodology is shown to be effective by applying it to data sets across Reynolds numbers,  $Re_{\unicode[STIX]{x1D70F}}\sim O(10^{3})$–
$Re_{\unicode[STIX]{x1D70F}}\sim O(10^{3})$–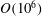 $O(10^{6})$.
$O(10^{6})$.