Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Chantry, M.
and
Kerswell, R. R.
2015.
Localization in a spanwise-extended model of plane Couette flow.
Physical Review E,
Vol. 91,
Issue. 4,
Deguchi, Kengo
2015.
Self-sustained states at Kolmogorov microscale.
Journal of Fluid Mechanics,
Vol. 781,
Issue. ,
Takeishi, Keisuke
Kawahara, Genta
Wakabayashi, Hiroki
Uhlmann, Markus
and
Pinelli, Alfredo
2015.
Localized turbulence structures in transitional rectangular-duct flow.
Journal of Fluid Mechanics,
Vol. 782,
Issue. ,
p.
368.
Hiruta, Yoshiki
and
Toh, Sadayoshi
2015.
Solitary solutions including spatially localized chaos and their interactions in two-dimensional Kolmogorov flow.
Physical Review E,
Vol. 92,
Issue. 6,
Mellibovsky, Fernando
and
Meseguer, Alvaro
2015.
A mechanism for streamwise localisation of nonlinear waves in shear flows.
Journal of Fluid Mechanics,
Vol. 779,
Issue. ,
Zammert, Stefan
and
Eckhardt, Bruno
2015.
Crisis bifurcations in plane Poiseuille flow.
Physical Review E,
Vol. 91,
Issue. 4,
Xi, Li
and
Bai, Xue
2016.
Marginal turbulent state of viscoelastic fluids: A polymer drag reduction perspective.
Physical Review E,
Vol. 93,
Issue. 4,
Kreilos, Tobias
Gibson, John F.
and
Schneider, Tobias M.
2016.
Localized travelling waves in the asymptotic suction boundary layer.
Journal of Fluid Mechanics,
Vol. 795,
Issue. ,
Ritter, Paul
Mellibovsky, Fernando
and
Avila, Marc
2016.
Emergence of spatio-temporal dynamics from exact coherent solutions in pipe flow.
New Journal of Physics,
Vol. 18,
Issue. 8,
p.
083031.
Khapko, T.
Kreilos, T.
Schlatter, P.
Duguet, Y.
Eckhardt, B.
and
Henningson, D. S.
2016.
Edge states as mediators of bypass transition in boundary-layer flows.
Journal of Fluid Mechanics,
Vol. 801,
Issue. ,
Zammert, Stefan
Fischer, Nicolas
and
Eckhardt, Bruno
2016.
Transition in the asymptotic suction boundary layer over a heated plate.
Journal of Fluid Mechanics,
Vol. 803,
Issue. ,
p.
175.
Zammert, Stefan
and
Eckhardt, Bruno
2016.
Streamwise decay of localized states in channel flow.
Physical Review E,
Vol. 94,
Issue. 4,
E, Weinan
and
Wang, Jianchun
2016.
A thermodynamic study of the two-dimensional pressure-driven channel flow.
Discrete and Continuous Dynamical Systems,
Vol. 36,
Issue. 8,
p.
4349.
Wall, D. P.
and
Nagata, M.
2016.
Exact coherent states in channel flow.
Journal of Fluid Mechanics,
Vol. 788,
Issue. ,
p.
444.
Gibson, J. F.
and
Schneider, T. M.
2016.
Homoclinic snaking in plane Couette flow: bending, skewing and finite-size effects.
Journal of Fluid Mechanics,
Vol. 794,
Issue. ,
p.
530.
Zammert, Stefan
and
Eckhardt, Bruno
2017.
Harbingers and latecomers – the order of appearance of exact coherent structures in plane Poiseuille flow.
Journal of Turbulence,
Vol. 18,
Issue. 2,
p.
103.
Barnett, Joshua
Gurevich, Daniel R.
and
Grigoriev, Roman O.
2017.
Streamwise localization of traveling wave solutions in channel flow.
Physical Review E,
Vol. 95,
Issue. 3,
Kushwaha, Anubhav
Park, Jae Sung
and
Graham, Michael D.
2017.
Temporal and spatial intermittencies within channel flow turbulence near transition.
Physical Review Fluids,
Vol. 2,
Issue. 2,
Tao, J. J.
Eckhardt, Bruno
and
Xiong, X. M.
2018.
Extended localized structures and the onset of turbulence in channel flow.
Physical Review Fluids,
Vol. 3,
Issue. 1,
Ritter, Paul
Zammert, Stefan
Song, Baofang
Eckhardt, Bruno
and
Avila, Marc
2018.
Analysis and modeling of localized invariant solutions in pipe flow.
Physical Review Fluids,
Vol. 3,
Issue. 1,
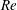 $\mathit{Re}$, but the downstream exponent is essentially independent of
$\mathit{Re}$, but the downstream exponent is essentially independent of  $\mathit{Re}$. In the spanwise direction the decay is compatible with a power-law localisation. As the width increases the localised state undergoes further bifurcations which add additional unstable directions, so that the edge state, the relative attractor on the boundary between the laminar and turbulent motions, in the system becomes chaotic.
$\mathit{Re}$. In the spanwise direction the decay is compatible with a power-law localisation. As the width increases the localised state undergoes further bifurcations which add additional unstable directions, so that the edge state, the relative attractor on the boundary between the laminar and turbulent motions, in the system becomes chaotic.