Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Li, Yuzhu
and
Fuhrman, David R.
2022.
On the turbulence modelling of waves breaking on a vertical pile.
Journal of Fluid Mechanics,
Vol. 953,
Issue. ,
Amroun, Hamdi
Hafid, Fikri
and
Ammi, Mehdi
2022.
Optimization of the Creation of a Training Set for the Calibration of a Model Reproducing the Vibration Behavior of an Overhead Line Conductor.
International Journal of Information and Communication Sciences,
Vol. 7,
Issue. 2,
p.
18.
Buccino, Mariano
Di Leo, Angela
Tuozzo, Sara
Còrdova Lopez, Luìs F.
Calabrese, Mario
and
Dentale, Fabio
2023.
Wave overtopping of a vertical seawall in a surf zone: A joint analysis of numerical and laboratory data.
Ocean Engineering,
Vol. 288,
Issue. ,
p.
116144.
Hu, Zhengyu
and
Li, Yuzhu
2023.
Two-dimensional simulations of large-scale violent breaking wave impacts on a flexible wall.
Coastal Engineering,
Vol. 185,
Issue. ,
p.
104370.
Qwist, Jesper Roland Kjærgaard
and
Christensen, Erik Damgaard
2023.
Development and implementation of a Direct Surface Description method for free surface flows in OpenFOAM.
Coastal Engineering,
Vol. 179,
Issue. ,
p.
104227.
Larsen, Bjarke Eltard
and
Fuhrman, David R.
2023.
Simulation of cross-shore breaker bar development utilizing a stabilized two-equation turbulence model.
Coastal Engineering,
Vol. 180,
Issue. ,
p.
104269.
Burgaard, Karen B.
Carstensen, Stefan
Fuhrman, David R.
Larsen, Bjarke E.
and
O’Neill, Finbarr G.
2023.
Experimental and numerical investigation of a disc-attached cylinder near a wall.
Ocean Engineering,
Vol. 285,
Issue. ,
p.
115366.
Tavakoli, Sasan
Khojasteh, Danial
Haghani, Milad
and
Hirdaris, Spyros
2023.
A review on the progress and research directions of ocean engineering.
Ocean Engineering,
Vol. 272,
Issue. ,
p.
113617.
Kranenborg, J.W.M.
Campmans, G.H.P.
van der Werf, J.J.
McCall, R.T.
Reniers, A.J.H.M.
and
Hulscher, S.J.M.H.
2024.
Effects of free surface modelling and wave-breaking turbulence on depth-resolved modelling of sediment transport in the swash zone.
Coastal Engineering,
Vol. 191,
Issue. ,
p.
104519.
Parvin, Amir H.
Abadie, Stéphane
Omari, Kamal El
and
Guer, Yves Le
2024.
Validation of OpenFOAM with respect to the elementary processes involved in the generation of waves by subaerial landslides.
Applied Ocean Research,
Vol. 153,
Issue. ,
p.
104296.
Tsai, Benjamin
Hsu, Tian‐Jian
Lee, Seok‐Bong
Pontiki, Maria
Puleo, Jack A.
and
Wengrove, Meagan E.
2024.
Large Eddy Simulation of Cross‐Shore Hydrodynamics Under Random Waves in the Inner Surf and Swash Zones.
Journal of Geophysical Research: Oceans,
Vol. 129,
Issue. 9,
Burgaard, Karen B.
Carstensen, Stefan
Fuhrman, David R.
and
O’Neill, Finbarr G.
2024.
Numerical simulation of particles beneath a towed circular cylinder.
Coastal Engineering,
Vol. 189,
Issue. ,
p.
104474.
Shirkavand, Ali
and
Farrahi-Moghaddam, Kambiz
2024.
A non-hydrostatic numerical model for simulating regular wave breaking and surf-swash zone motions.
Scientific Reports,
Vol. 14,
Issue. 1,
Yin, Zegao
Yang, Guilin
Wang, Yanxu
Qiu, Quanlin
and
Jiang, Xiutao
2024.
Numerical simulations of breaking wave propagation through the vegetation on a slope based on a drag coefficient prediction model.
Ocean Engineering,
Vol. 291,
Issue. ,
p.
116440.
Li, Zhisong
and
Guo, Xiaoyu
2024.
Numerical analyses of water swirls in aquaculture tanks.
Physics of Fluids,
Vol. 36,
Issue. 7,
Li, Zhisong
Guo, Xiaoyu
and
Cui, Mingchao
2024.
Sensitive analyses of flow property to turbulence model in swirls simulation within aquaculture tanks.
Ocean Engineering,
Vol. 291,
Issue. ,
p.
116425.
Liu, Yuxuan
van den Bremer, Ton S.
and
Adcock, Thomas A.A.
2024.
Numerical simulation of deep-water wave breaking using RANS: Comparison with experiments.
European Journal of Mechanics - B/Fluids,
Vol. 108,
Issue. ,
p.
211.
Chen, Jinshi
Raubenheimer, Britt
and
Elgar, Steve
2024.
Wave and Roller Transformation Over Barred Bathymetry.
Journal of Geophysical Research: Oceans,
Vol. 129,
Issue. 5,
Shirkavand, Ali
and
Farrahi-Moghaddam, Kambiz
2024.
The application of a non-hydrostatic RANS model for simulating irregular wave breaking on a barred and sloping beach.
Results in Engineering,
Vol. 23,
Issue. ,
p.
102451.
 $\omega$ turbulence closure model for this purpose, where
$\omega$ turbulence closure model for this purpose, where  $\omega$ is the specific dissipation rate. Novel stability analysis proves that, unlike two-equation closures (at least in their standard forms), the stress–
$\omega$ is the specific dissipation rate. Novel stability analysis proves that, unlike two-equation closures (at least in their standard forms), the stress– $\omega$ model is neutrally stable in the idealized potential flow region beneath surface waves. It thus naturally avoids unphysical exponential growth of turbulence prior to breaking, which has plagued numerous prior studies. The analysis is confirmed through simulation of a progressive surface wave train. The stress–
$\omega$ model is neutrally stable in the idealized potential flow region beneath surface waves. It thus naturally avoids unphysical exponential growth of turbulence prior to breaking, which has plagued numerous prior studies. The analysis is confirmed through simulation of a progressive surface wave train. The stress– $\omega$ model is then applied to simulate a turbulent wave boundary layer, demonstrating superior accuracy relative to a two-equation model, especially during flow deceleration. Finally, the stress–
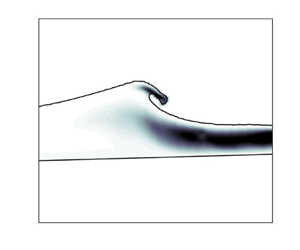
$\omega$ model is then applied to simulate a turbulent wave boundary layer, demonstrating superior accuracy relative to a two-equation model, especially during flow deceleration. Finally, the stress– $\omega$ model is employed to simulate spilling and plunging breaking waves, with seemingly unprecedented accuracy. Specifically, the present work marks the first time that a single turbulence closure model collectively: (1) avoids turbulence over-production prior to breaking, (2) accurately predicts the breaking point, (3) provides reasonable evolution of turbulent normal stresses, while also (4) yielding accurate evolution of undertow velocity structure and magnitude across the surf zone, for both spilling and plunging cases. Differences in the predicted Reynolds shear stresses (hence flow resistance) are identified as key to the improved inner surf zone performance, relative to a state-of-the-art two-equation model.
$\omega$ model is employed to simulate spilling and plunging breaking waves, with seemingly unprecedented accuracy. Specifically, the present work marks the first time that a single turbulence closure model collectively: (1) avoids turbulence over-production prior to breaking, (2) accurately predicts the breaking point, (3) provides reasonable evolution of turbulent normal stresses, while also (4) yielding accurate evolution of undertow velocity structure and magnitude across the surf zone, for both spilling and plunging cases. Differences in the predicted Reynolds shear stresses (hence flow resistance) are identified as key to the improved inner surf zone performance, relative to a state-of-the-art two-equation model.